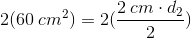We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

Advanced Geometry : Advanced Geometry

Study concepts, example questions & explanations for Advanced Geometry

Create An Account

All Advanced Geometry Resources

6 Diagnostic Tests 57 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Calculating The Length Of The Diagonal Of A Quadrilateral

Rhombus RHOM has area 56.

Which of the following could be true about the values of and ?

Possible Answers:

RO = 4, HM = 14

RO = 14, HM = 14

RO = 16, HM = 7

None of the other responses gives a correct answer.

RO = 28, HM = 28

Correct answer:

RO = 16, HM = 7

Explanation:

The area of a rhombus is half the product of the lengths of its diagonals, which here are $\overline{RO}$ and $\overline{HM}$ . This means

$\frac{1}{2} \cdot RO \cdot HM = 56$

Therefore, we need to test each of the choices to find the pair of diagonal lengths for which this holds.

RO = 28, HM = 28 :

Area: $\frac{1}{2} \cdot RO \cdot HM = \frac{1}{2} \cdot 28 \times 28 = 392$

RO = 14, HM = 14

Area: $\frac{1}{2} \cdot RO \cdot HM = \frac{1}{2} \cdot 14 \times 14 =98$

RO = 4, HM = 14

Area: $\frac{1}{2} \cdot RO \cdot HM = \frac{1}{2} \cdot 4 \times 14 = 28$

RO = 16, HM = 7

Area: $\frac{1}{2} \cdot RO \cdot HM = \frac{1}{2} \cdot16 \times 7 =56$

RO = 16, HM = 7 is the correct choice.

Report an Error

Example Question #51 : Rhombuses

Rhombus RHOM has perimeter 64; $m \angle R = 60 ^{\circ }$ . What is the length of $\overline{HM}$ ?

Possible Answers:

$16\sqrt{2}$

$16\sqrt{3}$

$8\sqrt{3}$

$8\sqrt{2}$

Correct answer:

Explanation:

The sides of a rhombus are all congruent; since the perimeter of Rhombus RHOM is 64, each side measures one fourth of this, or 16.

The referenced rhombus, along with diagonal $\overline{HM}$ , is below:

Rhombus

Since consecutive angles of a rhombus, as with any other parallelogram, are supplementary, $\angle RHO$ and $\angle RMO$ have measure $120^{\circ }$ ; $\overline{HM}$ bisects both into $60 ^{\circ }$ angles, making $\bigtriangleup RHM$ equilangular and, as a consequence, equilateral. Therefore, HM = RH = 16 .

Report an Error

Example Question #2 : Calculating The Length Of The Diagonal Of A Quadrilateral

Rhombus RHOM has perimeter 48; $m \angle R = 60 ^{\circ }$ . What is the length of $\overline{RO}$ ?

Possible Answers:

$6\sqrt{2}$

$6\sqrt{3}$

$12\sqrt{3}$

Correct answer:

$12\sqrt{3}$

Explanation:

The referenced rhombus, along with diagonals $\overline{RO}$ and $\overline{HM}$ , is below.

Rhombus

The four sides of a rhombus have equal measure, so each side has measure one fourth of the perimeter of 48, which is 12.

Since consecutive angles of a rhombus, as with any other parallelogram, are suplementary, $\angle RHO$ and $\angle RMO$ have measure $120^{\circ }$ ; the diagonals bisect $\angle MRH$ and $\angle RHO$ into $30 ^{\circ }$ and $60 ^{\circ }$ angles, respectively, to form four 30-60-90 triangles. $\bigtriangleup HXR$ is one of them; by the 30-60-90 Triangle Theorem, $HX = \frac{1}{2} \cdot RH = \frac{1}{2} \cdot 12 =6$ ,

and

$RX = HX \cdot \sqrt{3} = 6 \sqrt{3}$ .

Since the diagonals of a rhombus bisect each other, $RO = 2 \cdot RX = 2 \cdot 6 \sqrt{3} = 12\sqrt{3}$ .

Report an Error

Example Question #51 : Rhombuses

If the area of a rhombus is $60\:cm^2$ , and the length of one of its diagonals is $2\:cm$ , what must be the length of the other diagonal?

Possible Answers:

$30\:cm$

$60\:cm$

$90\:cm$

$120\:cm$

$45\:cm$

Correct answer:

$60\:cm$

Explanation:

Write the formula for the area of a rhombus.

$A=\frac{d_1\cdot d_2}{2}$

Plug in the given area and diagonal length. Solve for the other diagonal.

$60\:cm^2=\frac{2\:cm\cdot d_2}{2}$

$2(60\:cm^2)=2(\frac{2\:cm\cdot d_2}{2})$

$120\:cm^2=2\:cm\cdot d_2$

$\frac{120\:cm}{2\:cm}=\frac{2\:cm\cdot d_2}{2\:cm}$

$d_2 =60\:cm$

Report an Error

Example Question #141 : Quadrilaterals

ABCD is a rhombus. Find $m(\overline{BD})$ .

Varsity3

Possible Answers:

$m(\overline{BD}) = 36$

$m(\overline{BD}) = 6$

$m(\overline{BD}) \approx 9.83$

$m(\overline{BD}) = 35$

$m(\overline{BD}) = 12$

Correct answer:

$m(\overline{BD}) \approx 9.83$

Explanation:

Using the Law of Sines,

$\frac{sin(35^{o})}{6} = \frac{sin(110^{o})}{BD}$

$BD = \frac{6}{sin(35^{o})}\cdot sin(110^{o})$

$BD = m(\overline{BD})$

$m(\overline{BD})\approx 9.83$

Report an Error

Example Question #142 : Quadrilaterals

Find the lengths of the two diagonals, the longer diagonal is $d_{1}$ , the shorter diagonal is $d_{2}$ .

Varsity1

Possible Answers:

$d_{1} = 13$

$d_{2} = 5$

$d_{1} = 6$

$d_{2} = 12$

$d_{1} = 2$

$d_{2} = 6$

$d_{1} = 12$

$d_{2} = 6$

$d_{1} = 6\cdot \sqrt{3}$

$d_{2} = 6$

Correct answer:

$d_{1} = 6\cdot \sqrt{3}$

$d_{2} = 6$

Explanation:

1) All sides of a rhombus are congruent.

2) Because all sides of a rhombus are congruent, the expressions of the side lengths can be set equal to each other. The resulting equation is then solved,

2x - 4 = 3x - 9

9 - 4 = 3x - 2x

5 = x

x = 5

3) Because the sides of a rhombus are congruent, x = 5 can be substituted into either 2x - 4 or 3x - 9 to find the length of a side,

2(5) - 4 = 6 , or, 3(5) - 9 = 6 .

4) Each of the composing triangles are right triangles, so then is the length of the hypotenuse for each triangle.

5) 6 = 3(2) .

6) The standard 30 - 60 - 90 right triangle has a hypotenuse length equal to .

7) The hypotenuse of a standard 30 - 60 - 90 right triangle is being multiplied by .

The result is , so then is the scale factor for the triangle side lengths.

8) For the standard 30 - 60 - 90 right triangle, the other two side lengths are and $\sqrt{3}$ , so then the height of the triangle from step 7) has a height of 3(1) , and the base length is $3(\sqrt{3})$ .

9) The base of the triangle from step 7) is

$\frac{1}{2}d_{1} = 3\cdot(\sqrt{3})$ ,

and the height is

$\frac{1}{2}d_{2} = 3(1)$ .

10) Diagonal

$d_2=2\cdot \frac{1}{2}d_{2} = 2\cdot3 = 6$ ,

and diagonal

$d_{1} = 2\cdot \frac{1}{2}d_{1} = 2\cdot3(\sqrt{3}) = 6(\sqrt{3})$ .

Report an Error

Example Question #141 : Quadrilaterals

Screen_shot_2015-03-06_at_3.16.41_pm

What is the second diagonal for the above rhombus?

Possible Answers:

Correct answer:

Explanation:

Because a rhombus has vertical and horizontal symmetry, it can be broken into four congruent triangles, each with a hypotenuse of 13 and a base of 5 (half the given diagonal).

The Pythagorean Theorem

a^2+b^2=c^2 will yield,

$\sqrt{169-25}=12$ for the height of the triangles.

The greater diagonal is twice the height of the triangles therefore, the greater diagonal becomes:

$d=12 \cdot 2$

d=24

Report an Error

Example Question #11 : How To Find The Length Of The Diagonal Of A Rhombus

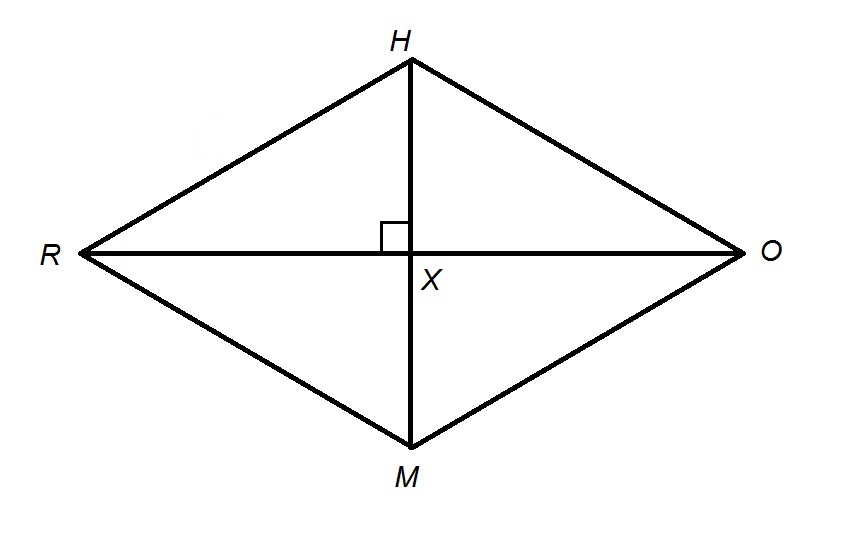
ABCD is rhombus with side lengths in meters. $\overline{AB} = 13$ and $\overline{BD} = 10$ . What is the length, in meters, of $\overline{AC}$ ?

Rhombus_1

Possible Answers:

Correct answer:

Explanation:

A rhombus is a quadrilateral with four sides of equal length. Rhombuses have diagonals that bisect each other at right angles.

Rhombus_2

Thus, we can consider the right triangle AED to find the length of diagonal $\overline{AC}$ . From the given information, each of the sides of the rhombus measures meters and $\overline{BD} = 10$ .

Because the diagonals bisect each other, we know:

$\overline{AD} = 13$

$\overline{ED} = 5$

Using the Pythagorean theorem,

a^2 + b^2 = c^2

$\overline{AE}^2 + 5^2 = 13^2$

$\overline{AE}^2 = 144$

$\overline{AE} = 12$

$\overline{AC} = 24$

Report an Error

Example Question #441 : Advanced Geometry

Let $\small \small f(x)=x^4-5x^3+2x^2+9$ . If $\small g(x)$ is equal to $\small f(x)$ when flipped across the x-axis, what is the equation for $\small g(x)$ ?

Possible Answers:

$\small x^4+5x^3+2x^2+9$

$\small x^4+5x^3+2x^2-9$

$\small -x^4-5x^3-2x^2-9$

$\small x^4-5x^3+2x^2+9$

$\small -x^4+5x^3-2x^2-9$

Correct answer:

$\small -x^4+5x^3-2x^2-9$

Explanation:

When a function $\small f(x)$ is flipped across the x-axis, the new function $\small g(x)$ is equal to $\small -f(x)$ . Therefore, our function $\small g(x)$ is equal to:

$\small g(x)=-f(x)=-(x^4-5x^3+2x^2+9)=-x^4+5x^3-2x^2-9$

Our final answer is therefore $\small g(x)=-x^4+5x^3-2x^2-9$

Report an Error

Example Question #442 : Advanced Geometry

Let $\small f(x)=x^5-2x^4-6x^3+x^2+5x$ . If we let $\small g(x)$ equal $\small f(x)$ when it is flipped across the y-axis, what is the equation for $\small \small g(x)$ ?

Possible Answers:

$\small x^5-2x^4+6x^3-x^2+5x$

$\small x^5+2x^4-6x^3-x^2+5x$

$\small x^5+2x^4+6x^3+x^2+5x$

$\small -x^5+2x^4+6x^3-x^2-5x$

$\small -x^5-2x^4+6x^3+x^2-5x$

Correct answer:

$\small -x^5-2x^4+6x^3+x^2-5x$

Explanation:

When a function $\small f(x)$ is flipped across the y-axis, the resulting function $\small g(x)$ is equal to $\small f(-x)$ . Therefore, to find our $\small g(x)$ , we must substitute in $\small -x$ for every $\small x$ is our equation:

$\small g(x)=(-x)^5-2(-x)^4-6(-x)^3+(-x)^2+5(-x)=-x^5-2x^4+6x^3+x^2-5x$

Our final answer is therefore $\small g(x)=-x^5-2x^4+6x^3+x^2-5x$

Report an Error

View Tutors

Owen E
Certified Tutor

University of Kansas, Bachelors, English. University of Kansas, Masters, TESOL.

View Tutors

Isabella
Certified Tutor

Iowa State University, Bachelor of Science, Mathematics.

View Tutors

Melodi
Certified Tutor

Case Western Reserve University, Bachelor of Science, Computer Science.

All Advanced Geometry Resources

6 Diagnostic Tests 57 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Biology Tutors in San Diego, Calculus Tutors in Houston, Computer Science Tutors in Houston, Spanish Tutors in New York City, SSAT Tutors in Washington DC, SAT Tutors in San Diego, GRE Tutors in Miami, Physics Tutors in San Diego, MCAT Tutors in San Diego, SAT Tutors in New York City

Popular Courses & Classes

ISEE Courses & Classes in New York City, SAT Courses & Classes in Denver, ACT Courses & Classes in San Francisco-Bay Area, GMAT Courses & Classes in Philadelphia, MCAT Courses & Classes in Washington DC, GRE Courses & Classes in Phoenix, SSAT Courses & Classes in Washington DC, GMAT Courses & Classes in Chicago, Spanish Courses & Classes in San Diego, ISEE Courses & Classes in Miami

Popular Test Prep

SAT Test Prep in Houston, LSAT Test Prep in Seattle, GRE Test Prep in Houston, SAT Test Prep in Dallas Fort Worth, MCAT Test Prep in Dallas Fort Worth, GRE Test Prep in Phoenix, SSAT Test Prep in Miami, SAT Test Prep in Washington DC, ACT Test Prep in New York City, MCAT Test Prep in Los Angeles

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Advanced Geometry : Advanced Geometry

Study concepts, example questions & explanations for Advanced Geometry

All Advanced Geometry Resources

Example Questions

Example Question #1 : Calculating The Length Of The Diagonal Of A Quadrilateral

Example Question #51 : Rhombuses

Example Question #2 : Calculating The Length Of The Diagonal Of A Quadrilateral

Example Question #51 : Rhombuses

Example Question #141 : Quadrilaterals

Example Question #142 : Quadrilaterals

Example Question #141 : Quadrilaterals

Example Question #11 : How To Find The Length Of The Diagonal Of A Rhombus

Example Question #441 : Advanced Geometry

Example Question #442 : Advanced Geometry

All Advanced Geometry Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: