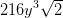Call Now to Set Up Tutoring:

(888) 888-0446

SSAT Upper Level Math : Geometry

Study concepts, example questions & explanations for SSAT Upper Level Math

Create An Account

All SSAT Upper Level Math Resources

6 Diagnostic Tests 311 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #861 : Ssat Upper Level Quantitative (Math)

The distance from one vertex of a cube to its opposite vertex is one foot. Give the volume of the cube in inches.

Possible Answers:

$216 \textup{ in}^{3}$

$266 \sqrt{2} \textup{ in}^{3}$

$192 \sqrt{3} \textup{ in}^{3}$

$288 \textup{ in}^{3}$

$64 \sqrt{3} \textup{ in}^{3}$

Correct answer:

$192 \sqrt{3} \textup{ in}^{3}$

Explanation:

Since we are looking at inches, we will look at one foot as twelve inches.

Let be the length of one edge of the cube. By the three-dimensional extension of the Pythagorean Theorem,

$s^{2}+s^{2}+s^{2}=12^{2}$

$3s^{2}=144$

$s^{2}=48$

$s= \sqrt{48} = \sqrt{16} \cdot \sqrt{3} = 4\sqrt{3}$ inches.

Cube this sidelength to get the volume:

$s^{3}= \left ( 4\sqrt{3} \right )^{3} = 4^{3} \cdot \left ( \sqrt{3} \right ) ^{3} = 64 \cdot 3 \sqrt{3}= 192 \sqrt{3}$ cubic inches.

Report an Error

Example Question #862 : Ssat Upper Level Quantitative (Math)

The distance from one vertex of a perfectly cubic aquarium to its opposite vertex is 4.5 meters. Give the volume of the aquarium in liters.

cubic meter = 1,000 liters.

Possible Answers:

$6,750 \sqrt{2} \textup{ L}$

$6,750 \textup{ L}$

$10,125 \textup{ L}$

$10,125\sqrt{3}\textup{ L}$

$91,125 \textrm{ L}$

Correct answer:

$10,125\sqrt{3}\textup{ L}$

Explanation:

Let be the length of one edge of the cube. By the three-dimensional extension of the Pythagorean Theorem,

$s^{2}+s^{2}+s^{2}=4.5^{2}$

$3s^{2}=20.25$

$s^{2} =6.75 = \frac{27}{4}$

$s = \sqrt{\frac{27}{4}} = \frac{\sqrt{27}}{\sqrt{4}}} = \frac{ \sqrt{9} \cdot \sqrt{3}}{2}= \frac{3\sqrt{3}}{2}$ meters.

Cube this sidelength to get the volume:

$s ^{3 } =\left ( \frac{3\sqrt{3}}{2} \right )^{3 }= \frac{3^{3 } \left (\sqrt{3}\right )^{3 }}{2^{3 } } = \frac{ 27 \cdot 3\sqrt{3} }{8 } = \frac{ 81 \sqrt{3} }{8 }$ cubic meters.

To convert this to liters, multiply by 1,000:

$\frac{ 81\sqrt{3} }{8 } \cdot 1,000 = \frac{81\sqrt{3} }{8 } \cdot \frac{1,000}{1} = \frac{ 81\sqrt{3} }{1 } \cdot \frac{125}{1}= 10,125\sqrt{3}$ liters.

Report an Error

Example Question #641 : Geometry

Find the volume of a cube with a side length of .

Possible Answers:

800

$8\textup{,}000$

$4\textup{,}000$

400

$6\textup{,}000$

Correct answer:

$8\textup{,}000$

Explanation:

Write the formula for the volume of a cube.

$V=a^3=(20)^3=8\textup{,}000$

The correct answer is $8\textup{,}000$ .

Report an Error

Example Question #1 : How To Find The Volume Of A Polyhedron

Find the volume of a square pyramid that has a height of and a side length of .

Possible Answers:

Correct answer:

Explanation:

The formula to find the volume of a square pyramid is

$\text{Volume}=(side)^2\frac{height}{3}$

So plugging in the information given from the question,

$\text{Volume}=4^2\frac{9}{3}=16\times3=48$

Report an Error

Example Question #2 : How To Find The Volume Of A Polyhedron

Find the volume of a square pyramid with a height of and a length of a side of its square base of .

Possible Answers:

Correct answer:

Explanation:

The formula to find the volume of a square pyramid is

$\text{Volume}=(side)^2\frac{height}{3}$

So plugging in the information given from the question,

$\text{Volume}=(3^2)\frac{12}{3}=9\times 4=36$

Report an Error

Example Question #3 : How To Find The Volume Of A Polyhedron

Find the volume of a regular hexagonal prism that has a height of . The side length of the hexagon base is .

Possible Answers:

$660\sqrt3$

$4320\sqrt3$

$2160\sqrt3$

$1440\sqrt3$

Correct answer:

$2160\sqrt3$

Explanation:

The formula to find the volume of a hexagonal prism is

$\text{Volume}=\frac{3\sqrt3}{2}(side)^2(height)$

Plugging in the values given by the question will give

$\text{Volume}=\frac{3\sqrt3}{2}(12^2)(10)=2160\sqrt3$

Report an Error

Example Question #4 : How To Find The Volume Of A Polyhedron

In terms of , find the volume of a regular hexagonal prism that has a height of . The hexagon base has side lengths of .

Possible Answers:

$360x^2\sqrt3$

$120x\sqrt3$

$240x\sqrt3$

$120x^2\sqrt3$

Correct answer:

$120x^2\sqrt3$

Explanation:

The formula to find the volume of a hexagonal prism is

$\text{Volume}=\frac{3\sqrt3}{2}(side)^2(height)$

Plugging in the values given by the question will give

$\text{Volume}=\frac{3\sqrt3}{2}(4x)^2(5)=120x^2\sqrt3$

Report an Error

Example Question #4 : How To Find The Volume Of A Polyhedron

Find the volume of a regular tetrahedron with a side length of .

Possible Answers:

$966\sqrt2$

$1728\sqrt2$

$288\sqrt2$

$144\sqrt2$

Correct answer:

$144\sqrt2$

Explanation:

The formula to find the volume of a tetrahedron is

$\text{Volume}=\frac{\sqrt2}{12}(side)^3$

Plugging in the information given by the question gives

$\text{Volume}=\frac{\sqrt2}{12}(12)^3=144\sqrt2$

Report an Error

Example Question #5 : How To Find The Volume Of A Polyhedron

Find the volume of a regular octahedron that has a side length of .

Possible Answers:

$100\sqrt2$

$\frac{512\sqrt2}{3}$

$\frac{500\sqrt2}{3}$

$512\sqrt2$

Correct answer:

$\frac{512\sqrt2}{3}$

Explanation:

Use the following formula to find the volume of a regular octahedron:

$\text{Volume}=\frac{\sqrt2}{3}(side)^3$

Plugging in the information from the question,

$\text{Volume}=\frac{\sqrt2}{3}(8)^3=\frac{512\sqrt2}{3}$

Report an Error

Example Question #6 : How To Find The Volume Of A Polyhedron

Find the volume of a regular octahedron that has a side length of .

Possible Answers:

$216y^3\sqrt2$

$144y^3\sqrt2$

$72y^3\sqrt2$

$84y^3\sqrt2$

Correct answer:

$72y^3\sqrt2$

Explanation:

Use the following formula to find the volume of a regular octahedron:

$\text{Volume}=\frac{\sqrt2}{3}(side)^3$

Plugging in the information from the question,

$\text{Volume}=\frac{\sqrt2}{3}(6y)^3=72y^3\sqrt2$

Report an Error

View SSAT- Upper Level Tutors

Keith
Certified Tutor

Xavier University, Bachelor in Business Administration, Accounting. Ohio University-Main Campus, Masters in Education, Mathem...

View SSAT- Upper Level Tutors

Crystal
Certified Tutor

SUNY at Binghamton, Bachelor of Science, Mechanical Engineering.

View SSAT- Upper Level Tutors

Brandon
Certified Tutor

Flager College, Bachelor, Elementary Education .

All SSAT Upper Level Math Resources

6 Diagnostic Tests 311 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Reading Tutors in Chicago, ACT Tutors in San Diego, LSAT Tutors in Atlanta, Math Tutors in Los Angeles, LSAT Tutors in Philadelphia, Computer Science Tutors in Phoenix, Algebra Tutors in Philadelphia, ACT Tutors in Boston, ISEE Tutors in Seattle, GMAT Tutors in Philadelphia

Popular Courses & Classes

MCAT Courses & Classes in Miami, SAT Courses & Classes in Miami, LSAT Courses & Classes in Houston, MCAT Courses & Classes in Washington DC, ISEE Courses & Classes in Miami, GRE Courses & Classes in Chicago, Spanish Courses & Classes in Seattle, GRE Courses & Classes in Seattle, SAT Courses & Classes in Seattle, GMAT Courses & Classes in San Francisco-Bay Area

Popular Test Prep

SSAT Test Prep in San Diego, ACT Test Prep in Los Angeles, ISEE Test Prep in Denver, LSAT Test Prep in Philadelphia, GRE Test Prep in Boston, ISEE Test Prep in San Diego, SSAT Test Prep in Philadelphia, LSAT Test Prep in New York City, MCAT Test Prep in Miami, GMAT Test Prep in Chicago

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

SSAT Upper Level Math : Geometry

Study concepts, example questions & explanations for SSAT Upper Level Math

All SSAT Upper Level Math Resources

Example Questions

Example Question #861 : Ssat Upper Level Quantitative (Math)

Example Question #862 : Ssat Upper Level Quantitative (Math)

Example Question #641 : Geometry

Example Question #1 : How To Find The Volume Of A Polyhedron

Example Question #2 : How To Find The Volume Of A Polyhedron

Example Question #3 : How To Find The Volume Of A Polyhedron

Example Question #4 : How To Find The Volume Of A Polyhedron

Example Question #4 : How To Find The Volume Of A Polyhedron

Example Question #5 : How To Find The Volume Of A Polyhedron

Example Question #6 : How To Find The Volume Of A Polyhedron

All SSAT Upper Level Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: