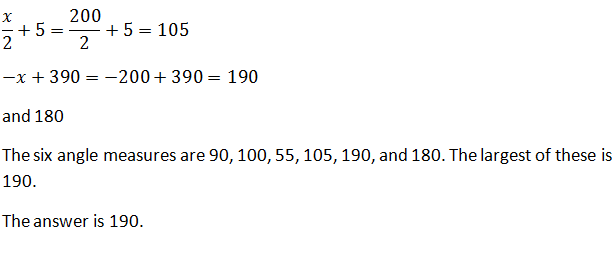Call Now to Set Up Tutoring:

(888) 888-0446

PSAT Math : Plane Geometry

Study concepts, example questions & explanations for PSAT Math

Create An Account

All PSAT Math Resources

10 Diagnostic Tests 421 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #2 : How To Find The Length Of The Diagonal Of A Hexagon

Regular hexagon ABCDEF has side length 1.

Give the length of diagonal $\overline{A E}$ .

Possible Answers:

$\frac{\sqrt{2}}{2}$

$\sqrt{2}$

$\frac{\sqrt{3}}{2}$

$\frac{3}{2}$

$\sqrt{3}$

Correct answer:

$\sqrt{3}$

Explanation:

The key is to examine $\Delta AED$ in thie figure below:

Hexagon_50

Each interior angle of a regular hexagon, including $\angle F$ , measures $120^{\circ }$ , so it can be easily deduced by way of the Isosceles Triangle Theorem that $m \angle FEA = 30^{\circ }$ . To find $m \angle AED$ we subtract $m \angle FEA = 30^{\circ }$ from $m \angle FED = 120^{\circ }$ . Thus resullting in $m \angle AED=90^{\circ}$

Also, by symmetry,

$m \angle EDA = \frac{1}{2} \cdot 120^{\circ } = 60^{\circ }$ ,

so $m \angle EAD= 30^{\circ }$ ,

and $\Delta AED$ is a $30^{\circ}-60^{\circ}-90^{\circ}$ triangle whose short leg $\overline{ED}$ has length ED = 1 .

The long leg $\overline{AE}$ of this $30^{\circ}-60^{\circ}-90^{\circ}$ triangle measures $\sqrt{3}$ times the length of short leg $\overline{ED}$ , so $AE= \sqrt{3}$ .

Report an Error

Example Question #581 : Geometry

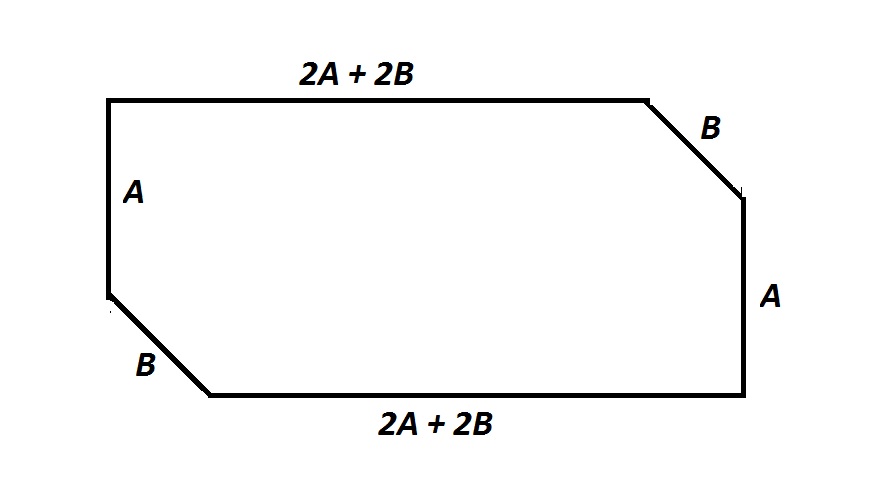
Hexagon

Note: Figure NOT drawn to scale.

The perimeter of the above hexagon is 888. Also, A-B = 10 . Evaluate .

Possible Answers:

A = 79

$A = 93\frac{4}{5}$

$A = 60\frac{1}{2}$

Insufficient information is given to answer the problem.

A = 116

Correct answer:

A = 79

Explanation:

The perimeter of the figure can be expressed in terms of the variables by adding:

$A + B + A + B + \left (2A + 2B \right ) + \left (2A + 2B \right ) = P$

Simplify and set P = 888 :

A + A + 2A + 2A + B+ B +2B + 2B= 888

6A + 6B= 888

$6\left (A + B \right )= 888$

A + B = 148

Along with A-B = 10 , we now have a system of equations to solve for by adding:

A + B = 148

$\underline{A-B =\; 10}$

$2A = \; \; \; \; \; \; 158$

A = 79

Report an Error

Example Question #1 : How To Find The Length Of The Side Of A Hexagon

Hexagon

Note: Figure NOT drawn to scale.

The perimeter of the above figure is 132. What is A + B ?

Possible Answers:

$26\frac{2}{5}$

$16 \frac{1}{2}$

Correct answer:

Explanation:

The perimeter of the figure can be expressed in terms of the variables by adding:

$A + B + A + B + \left (2A + 2B \right ) + \left (2A + 2B \right ) = P$

Simplify and set P = 132 :

A + A + 2A + 2A + B+ B +2B + 2B= 132

6A + 6B= 132

$6\left (A + B \right )= 132$

A + B = 22

Report an Error

Example Question #2 : Hexagons

Hexagon

Note: Figure NOT drawn to scale.

The perimeter of the above figure is 600. The ratio of to is 3:2 . Evaluate .

Possible Answers:

B = 72

B = 48

B = 100

B = 40

B = 60

Correct answer:

B = 40

Explanation:

The perimeter of the figure can be expressed in terms of the variables by adding:

$A + B + A + B + \left (2A + 2B \right ) + \left (2A + 2B \right ) = P$

Simplify and set P = 600 :

A + A + 2A + 2A + B+ B +2B + 2B= 600

6A + 6B= 600

Since the ratio of to is equivalent to 3:2 - or

$\frac{A}{B} = \frac{3}{2}$ ,

then

$A = \frac{3}{2} B$

and we can substitute as follows:

$6 \cdot \frac{3}{2}B + 6B= 600$

9B + 6B= 600

15B = 600

B = 40

Report an Error

Example Question #2 : Hexagons

Possible Answers:

190

210

180

200

170

Correct answer:

190

Explanation:

Report an Error

Example Question #21 : Geometry

If a triangle has 180 degrees, what is the sum of the interior angles of a regular octagon?

Possible Answers:

540

720

1260

900

1080

Correct answer:

1080

Explanation:

The sum of the interior angles of a polygon is given by 180(n - 2) where = number of sides of the polygon. An octagon has 8 sides, so the formula becomes 180(8 -2) = 1080

Report an Error

Example Question #2 : How To Find An Angle In A Hexagon

In a rectangular hexagon, what is the meaure of each interior angle?

Possible Answers:

120 degrees

72 degrees

90 degrees

150 degrees

105 degrees

Correct answer:

120 degrees

Explanation:

The sum of the interior angles of a hexagon must equal 720 degrees. Because the hexagon is regular, all of the interior angles will have the same measure. A hexagon has six sides and six interior angles. Therefore, each angle measures $\frac{720}{6}=120$ .

Report an Error

Example Question #1 : How To Find An Angle In A Hexagon

Hexagon

Note:Figure NOT drawn to scale.

Refer to the above figure. Evaluate .

Possible Answers:

x = 130

x = 140

x = 100

x = 110

x = 120

Correct answer:

x = 130

Explanation:

The sum of the degree measures of the angles of a (six-sided) hexagon, is

$180 ^{\circ } \times (6 - 2) = 180 ^{\circ } \times 4 = 720^{\circ }$

We can solve for in the equation

x + 92 + 164 + 90 + 137 + 107 = 720

x +590 = 720

x = 130

Report an Error

Example Question #581 : Geometry

Hexagon

Note: Figure NOT drawn to scale.

Refer to the above figure. Evaluate .

Possible Answers:

x = 101

x = 111

x = 106

x = 116

x = 121

Correct answer:

x = 111

Explanation:

The sum of the degree measures of the angles of a (six-sided) hexagon, is

$180 ^{\circ } \times (6 - 2) = 180 ^{\circ } \times 4 = 720^{\circ }$

We can solve for in the equation

x + x+ 164 + 90 + 137 + 107 = 720

2x + 498 = 720

2x = 222

x=111

Report an Error

Example Question #582 : Geometry

Three angles of a hexagon measure $65^{\circ}, 74^{\circ}, 89^{\circ}$ . The other three angles are congruent to one another. What is the measure of each of the latter three angles?

Possible Answers:

This hexagon cannot exist.

$164^{\circ}$

$174^{\circ}$

$48^{\circ}$

$58^{\circ}$

Correct answer:

$164^{\circ}$

Explanation:

The sum of the degree measures of the angles of a (six-sided) hexagon, is

$180 ^{\circ } \times (6 - 2) = 180 ^{\circ } \times 4 = 720^{\circ }$

Let be the common measure of the three congruent angles in question. We can solve for in the equation

x + x + x + 65+ 74 + 89 = 720

3 x + 228 = 720

3 x = 492

x = 164

Report an Error

View PSAT Mathematics Tutors

Darian
Certified Tutor

University of North Texas, Current Undergrad Student, English.

View PSAT Mathematics Tutors

Michael
Certified Tutor

University of Massachusetts Amherst, Bachelor in Arts, Political Science and Government. University of Massachusetts Amherst,...

View PSAT Mathematics Tutors

Joy
Certified Tutor

Boston University, Bachelor in Arts, Conservation Biology.

All PSAT Math Resources

10 Diagnostic Tests 421 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

ACT Tutors in Denver, MCAT Tutors in Dallas Fort Worth, Computer Science Tutors in San Diego, French Tutors in Dallas Fort Worth, Computer Science Tutors in Dallas Fort Worth, Biology Tutors in Boston, Math Tutors in Boston, Calculus Tutors in Atlanta, Spanish Tutors in Phoenix, MCAT Tutors in Miami

Popular Courses & Classes

LSAT Courses & Classes in Boston, SAT Courses & Classes in Houston, LSAT Courses & Classes in Dallas Fort Worth, GMAT Courses & Classes in Phoenix, MCAT Courses & Classes in Dallas Fort Worth, ACT Courses & Classes in Los Angeles, GMAT Courses & Classes in Washington DC, MCAT Courses & Classes in Washington DC, ACT Courses & Classes in Phoenix, ISEE Courses & Classes in San Diego

Popular Test Prep

SAT Test Prep in Denver, GRE Test Prep in Denver, MCAT Test Prep in San Diego, SAT Test Prep in Seattle, GRE Test Prep in Phoenix, ACT Test Prep in Dallas Fort Worth, GRE Test Prep in San Francisco-Bay Area, SSAT Test Prep in San Francisco-Bay Area, ISEE Test Prep in Miami, LSAT Test Prep in Denver

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

PSAT Math : Plane Geometry

Study concepts, example questions & explanations for PSAT Math

All PSAT Math Resources

Example Questions

Example Question #2 : How To Find The Length Of The Diagonal Of A Hexagon

Example Question #581 : Geometry

Example Question #1 : How To Find The Length Of The Side Of A Hexagon

Example Question #2 : Hexagons

Example Question #2 : Hexagons

Example Question #21 : Geometry

Example Question #2 : How To Find An Angle In A Hexagon

Example Question #1 : How To Find An Angle In A Hexagon

Example Question #581 : Geometry

Example Question #582 : Geometry

All PSAT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: