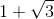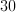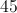Call Now to Set Up Tutoring:

(888) 888-0446

PSAT Math : Plane Geometry

Study concepts, example questions & explanations for PSAT Math

Create An Account

All PSAT Math Resources

10 Diagnostic Tests 421 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : How To Find The Length Of A Diagonal Of A Polygon

Regular Polygon ABCDEFGHIJKL (a twelve-sided polygon, or dodecagon) has sidelength 1.

Give the length of diagonal $\overline{AD}$ to the nearest tenth.

Possible Answers:

$\frac{2 + \sqrt{2}}{2}$

$1 + \sqrt{2}$

$\frac{2 + \sqrt{3}}{2}$

$1 + \sqrt{3}$

Correct answer:

$1 + \sqrt{3}$

Explanation:

The trick is to construct segments perpendicular to $\overline{AD}$ from and , calling the points of intersection and respectively.

Dodecagon

Each interior angle of a regular dodecagon measures

$\frac{\left ( 12 - 2\right ) 180^{\circ }}{12} = 150^{\circ }$ .

Since $\overline{BX}$ and $\overline{CY}$ are perpendicular to $\overline{AD}$ , it can be shown via symmetry that they are also perpendicular to $\overline{BC}$ . Therefore,

$\angle ABX$ and $\angle DCY$ both measure $60^{\circ }$

and $\Delta ABX$ and $\Delta DCY$ are $30^{\circ}-60^{\circ}-90^{\circ}$ triangles with long legs $\overline{AX}$ and $\overline{YD}$ . Since their hypotenuses are sides of the dodecagon and therefore have length 1,

$AX = YD = \frac{\sqrt{3}}{2}$ .

Also, since Quadrilateral BCXY is a rectangle, XY = BC = 1 .

The length of diagonal $\overline{AD}$ is $AD = AX + XY + YD = \frac{\sqrt{3}}{2}+ 1 + \frac{\sqrt{3}}{2} = 1 + \sqrt{3}$ .

Report an Error

Example Question #1 : How To Find The Perimeter Of A Polygon

Rectangle_3

Note: Figure NOT drawn to scale.

Refer to the above figure. The white trapezoid is isosceles. Give the perimeter of the blue polygon.

Possible Answers:

318

$264 + 18 \sqrt{3}$

$264 + 18 \sqrt{2}$

$264 + 36 \sqrt{2}$

336

Correct answer:

$264 + 36 \sqrt{2}$

Explanation:

The lower base of this trapezoid is 100 - 64 = 36 units longer than the upper base, and being isosceles, it is symmetrical. As a result, the lower leg of the right triangle at bottom is half this difference, or 18, which is the same as the upper leg. That makes the right triangle isosceles, and, therefore, a 45-45-90 triangle. 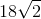Subsequently, the hypotenuse, which is one leg of the trapezoid, has length $\sqrt{2}$ times a leg of the triangle, or $18 \sqrt{2}$ .

The blue polygon has two sides that are the legs of this isosceles trapezoid, both of which have length $18 \sqrt{2}$ ; its other three sides are of length 64, 50, 100, and 50. Add them:

$P = 100 + 64 + 50 + 50 + 18 \sqrt{2}+ 18 \sqrt{2} = 264 + 36 \sqrt{2}$

Report an Error

Example Question #5 : Isosceles Triangles

Triangle ABC has angle measures as follows:

$\dpi{100} \small m\angle ABC=4x+3$

$\dpi{100} \small m\angle ACB=2x+6$

$\dpi{100} \small m\angle BAC=3x$

What is $\dpi{100} \small m\angle BAC$ ?

Possible Answers:

Correct answer:

Explanation:

The sum of the measures of the angles of a triangle is 180.

Thus we set up the equation $\dpi{100} \small 4x+3+2x+6+3x=180$

After combining like terms and cancelling, we have $\dpi{100} \small 9x=171\rightarrow x=19$

Thus $\dpi{100} \small m\angle BAC=3x=57$

Report an Error

Example Question #6 : Isosceles Triangles

The base angle of an isosceles triangle is five more than twice the vertex angle. What is the base angle?

Possible Answers:

$47$

$55$

$34$

$62$

$73$

Correct answer:

$73$

Explanation:

Every triangle has 180 degrees. An isosceles triangle has one vertex angle and two congruent base angles.

Let $x$ = the vertex angle and $2x+5$ = the base angle

So the equation to solve becomes $x+(2x+5)+(2x+5)=180$

Thus the vertex angle is 34 and the base angles are 73.

Report an Error

Example Question #6 : Isosceles Triangles

The base angle of an isosceles triangle is 15 less than three times the vertex angle. What is the vertex angle?

Possible Answers:

Correct answer:

Explanation:

Every triangle contains 180 degrees. An isosceles triangle has one vertex angle and two congruent base angles.

Let = vertex angle and 3x-15 = base angle

So the equation to solve becomes x+(3x-15)+(3x-15)=180 .

Report an Error

Example Question #1 : Isosceles Triangles

The base angle of an isosceles triangle is ten less than twice the vertex angle. What is the vertex angle?

Possible Answers:

$65^{\circ}$

$40^{\circ}$

$20^{\circ}$

$70^{\circ}$

$35^{\circ}$

Correct answer:

$40^{\circ}$

Explanation:

Every triangle has 180 degrees. An isosceles triangle has one vertex angle and two congruent base angles.

Let = vertex angle and 2x - 10 = base angle

So the equation to solve becomes x + (2x - 10) + (2x - 10) = 180

So the vertex angle is 40 and the base angles is 70

Report an Error

Example Question #2 : Isosceles Triangles

The base angle of an isosceles triangle is 10 more than twice the vertex angle. What is the vertex angle?

Possible Answers:

$74^{\circ}$

$32^{\circ}$

$50^{\circ}$

$60^{\circ}$

$45^{\circ}$

Correct answer:

$32^{\circ}$

Explanation:

Every triangle has 180 degrees. An isosceles triangle has one vertex angle and two congruent base angles.

Let = the vertex angle and 2x + 10 = the base angle

So the equation to solve becomes x + (2x +10) + (2x +10) = 180

The vertex angle is 32 degrees and the base angle is 74 degrees

Report an Error

Example Question #2 : Isosceles Triangles

In an isosceles triangle, the vertex angle is 15 less than the base angle. What is the base angle?

Possible Answers:

Correct answer:

Explanation:

Every triangle has 180 degrees. An isosceles triangle has one vertex angle and two congruent base angles.

Let = base angle and x - 15 = vertex angle

So the equation to solve becomes (x - 15) + x + x = 180

Thus, 65 is the base angle and 50 is the vertex angle.

Report an Error

Example Question #11 : Isosceles Triangles

In an isosceles triangle the vertex angle is half the base angle. What is the vertex angle?

Possible Answers:

$108$

$54$

$45$

$36$

$72$

Correct answer:

$36$

Explanation:

Every triangle has 180 degrees. An isosceles triangle has one vertex angle and two congruent base angles.

Let $x$ = base angle and $0.5x$ = vertex angle

So the equation to solve becomes $x+x+0.5x=180$ , thus $x=72$ is the base angle and $0.5x=36$ is the vertex angle.

Report an Error

Example Question #1 : Isosceles Triangles

If the average (arithmetic mean) of two noncongruent angles of an isosceles triangle is 55^o , which of the following is the measure of one of the angles of the triangle?

Possible Answers:

90^o

45^o

50^o

30^o

40^o

Correct answer:

40^o

Explanation:

Since the triangle is isosceles, we know that 2 of the angles (that sum up to 180) must be equal. The question states that the noncongruent angles average 55°, thus providing us with a system of two equations:

$\frac{x+y}{2}=55^o$

x+x+y=180^o

Solving for x and y by substitution, we get x = 70° and y = 40° (which average out to 55°).

70 + 70 + 40 equals 180 also checks out.

Since 70° is not an answer choice for us, we know that the 40° must be one of the angles.

Report an Error

View PSAT Mathematics Tutors

Michael
Certified Tutor

University of Massachusetts Amherst, Bachelor in Arts, Political Science and Government. University of Massachusetts Amherst,...

View PSAT Mathematics Tutors

Joy
Certified Tutor

Boston University, Bachelor in Arts, Conservation Biology.

View PSAT Mathematics Tutors

Yongmao
Certified Tutor

Nankai University, Bachelor of Science, Chemistry. Washington University in St Louis, Doctor of Philosophy, Organic Chemistry.

All PSAT Math Resources

10 Diagnostic Tests 421 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Chemistry Tutors in Dallas Fort Worth, Physics Tutors in Phoenix, SAT Tutors in San Diego, GRE Tutors in Chicago, French Tutors in Seattle, MCAT Tutors in Los Angeles, English Tutors in San Francisco-Bay Area, ACT Tutors in San Francisco-Bay Area, ACT Tutors in New York City, GRE Tutors in Denver

Popular Courses & Classes

GMAT Courses & Classes in New York City, ISEE Courses & Classes in Denver, LSAT Courses & Classes in Philadelphia, ISEE Courses & Classes in Miami, LSAT Courses & Classes in Boston, Spanish Courses & Classes in Chicago, Spanish Courses & Classes in Seattle, LSAT Courses & Classes in New York City, ACT Courses & Classes in Houston, ACT Courses & Classes in San Diego

Popular Test Prep

MCAT Test Prep in Boston, ISEE Test Prep in Houston, MCAT Test Prep in Philadelphia, ISEE Test Prep in Chicago, ISEE Test Prep in Philadelphia, SAT Test Prep in San Diego, SSAT Test Prep in Los Angeles, ISEE Test Prep in Dallas Fort Worth, GMAT Test Prep in Denver, SSAT Test Prep in Miami

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

PSAT Math : Plane Geometry

Study concepts, example questions & explanations for PSAT Math

All PSAT Math Resources

Example Questions

Example Question #1 : How To Find The Length Of A Diagonal Of A Polygon

Example Question #1 : How To Find The Perimeter Of A Polygon

Example Question #5 : Isosceles Triangles

Example Question #6 : Isosceles Triangles

Example Question #6 : Isosceles Triangles

Example Question #1 : Isosceles Triangles

Example Question #2 : Isosceles Triangles

Example Question #2 : Isosceles Triangles

Example Question #11 : Isosceles Triangles

Example Question #1 : Isosceles Triangles

All PSAT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: