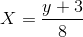First, we need to simplify what's inside the parentheses. 

Now we continue to evaluate the left hand side.

The right hand side does not need any reduction.

We set the two sides equal to each other.

In order to isolate the x-variable, we will need to multiply both sides by one third.

Simplify both sides.

The answer is:  

Add  on both sides.

Add one on both sides.

Divide by 16 on both sides.

The answer is:  

Upon solving for x, we find that x is less than or equal to 3.  The left-hand term of the interval is negative infinity since any number less than 3 is in our set, and infinity always has a parenthesis around it.  The right-hand term of the interval is 3 since it is the upper bound of our set.  There is a bracket around it because 3 is included in our set (3 is less than or equal to 3).  Remember when dividing or multiplying by a negative number in an inequality to reverse the direction of the inequality.

Step 1: Subtract 6 from both sides...

Step 2: Divide by 2.

Simplify:

Step 1: Subtract  from both sides

Step 2: Simplify:

Step 3: Divide..

Step 4: Take the square root of both sides...

Step 5: Simplify and get the answer...

Move  to the other side by subtracting it from both sides.

Simplify:

Divide by the coefficient, the number in front of x.

Reduce:

In , if we're solving for x, we first need to get the "x" term isolated. We do this by subtracting 3 from both sides so:

becomes

Now we divide both sides by 8

Re-written the answer becomes

Since this problems has 2 variables (D-dimes and Q-quarters) we need 2 equations. Because Larry has 14 coins, the first equation can be written as:

The value of those coins equals $2.60 or 260 cents. If Dimes are worth 10C and quarters are 25C, the next equation can be written as

To solve this write both equations on top of each other

Now we eliminate 1 variable by multiplying 1 equation by the lowest common denominator (as a negative) and adding the equations together.

  becomes

adding the equations

-----------------------------------

now we solve for Q.

Since we know Q, now we plug it back in to an equation and find D

Larry has 6 dimes and 8 quarters

There are two ways to solve for x and y in a pair of equations. One way is to add the two equations together and eliminate one of the variables. It may be necessary to multiply one equation by a positive or negative number in order to cancel out one of the variables. The second way is to pick one of the equations and solve for one of the variables. Let's pick the top equation and solve for x:

Now substitute x on the other (bottom) equation:

Now, since we know the value of y, use either equation and "plug in" the value of y:

To check your answers, you can plug in both answers to either equation, and since they are equations, if both sides are equal, your answers are correct.