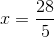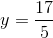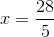Add the first two equations to eliminate the y and z-variable.

Using the value of x, with the first and third equations, we will need to eliminate the z-variable to solve for y.

Multiply the top equation by three.

Subtract both equations.

Divide both sides by two.

The answer is:  

The given system has as many variables as equations, which makes it possible to have exactly one solution.

One way to identify the solution set is to use Gauss-Jordan elimination on the augmented coefficient matrix

Perform operations on the rows, with the object of rendering this matrix in reduced row-echelon form.

First, a 1 is wanted in Row 1, Column 1. This is already the case, so 0's are wanted elsewhere in Column 1. Do this using the row operation:

Next, a 1 is wanted in Row 2, Column 2. This is already the case, so 0's are wanted elsewhere in Column 2. Do this using the row operations:

Next, a 1 is wanted in Row 3, Column 3.Perform the operation

Now, 0's are wanted in the rest of Column 3:

The matrix is in the desired form and can be interpreted to mean that the system has one and only one solution - . This makes the system consistent and independent.

To solve the system of linear equations, first isolate the equations with  and  to help solve for . This will include substitution.

Now that  is known it can be substituted back into the third equation to solve for .

Lastly, substitute  into the first equation and solve for .

Therefore, the solution to this system is

First step is to multiply Row 1 by , and substract Row 2, and put the resut in Row 2.

Now we have two equations.

, and 

Solve for y first, and then plug the result into the other equation.

1) Rewrite both the equations to tidy up and make sure all constant terms are isolated on one side of the equation. This will make it easier to write the augmented matrix. 

2) Write the augmented matrix corresponding to the system. 

Quick Refresher:

The identity matrix  has the form, 

We want to perform row operations until we have reduced the augmented matrix down to the form, 

where the column vector  represents what is left over from all the row operations performed to reduce the coefficient matrix down to the identity matrix. These will be the solution for  and . 

3) Now we can perform a succession of row operations to reduce to the desired form, 

We can start by cancelling out the  in the second row by subtracting the first row from the second row to obtain: 

Now we need a  to take the place of the  in the top row, so divide row 1 by :  

Now we need a  to take the place of the  in the second row, so divide the second row by , 

Now we need a  to replace the remaining  in the first row. Subtract 2-times the second row from the first row, 

4) Write the Solution Set:  

From the augmented matrix, we see that 

Now perform row operations to find x and y.

1. Switch Row 1 with Row 2:

2. Multiply Row 1 by-2 and add it to Row 2:

3. Multiply Row 3 by 13 and multiply Row 2 by -1. Add the two rows for a new Row 2:

4. Multiply Row 3 by -5 and add it to Row 1. Multiply Row 2 by -2 and add it to Row 1:

If, during the process of Gauss-Jordan elimination, any row becomes an all zero-row, it ceases to be a factor in determining the solution set. We must examine the top row of the matrix alone.

Remember that each entry to the left of the divider represents a coefficient and each entry to the right represents a constant. Therefore, the top row in the matrix 

represents the equation 

,

or, equivalently,

Therefore,  can assume any real value, and . There are therefore infinitely many solutions to this equation.

When applying the Gauss-Jordan method to solve a two-by-two linear system, the objective is to use row operations to form an augmented matrix of the form

  .

If this happens, then there is one and only one solution to the system, represented by the ordered pair . The augmented matrix given,

is in this form, and there is one solution, .

The red flag in this augmented matrix is the bottom row, which has 0's as all of its entries to the left of the divider and a nonzero entry to the right. Any time this happens during the process of Gauss-Jordan elimination, this signals that the system of equations has no solution.

If, during the process of Gauss-Jordan elimination, any row becomes an all zero-row, it ceases to be a factor in determining the solution set. We must examine the other rows of the matrix.

Remember that each entry to the left of the divider represents a coefficient and each entry to the right represents a constant. Therefore, the two nonzero rows in the matrix

represent the equations

and 

,

respectively.

The equations can be solved for  and  in terms of :

and 

Therefore,  can assume any real value, with  and  dependent on ; this is a dependent system, with infinitely many solutions.