Call Now to Set Up Tutoring:

(888) 888-0446

SAT Math : Circles

Study concepts, example questions & explanations for SAT Math

Create An Account

All SAT Math Resources

16 Diagnostic Tests 660 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #21 : Circles

What is the angle of a sector that has an arc length of 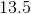13.5 on a circle of diameter ?

Possible Answers:

$194.14^{\circ}$

$10.74^{\circ}$

$128.92^{\circ}$

$35.81^{\circ}$

$14.24^{\circ}$

Correct answer:

$128.92^{\circ}$

Explanation:

The first thing to do for this problem is to compute the total circumference of the circle. Notice that you were given the diameter. The proper equation is therefore:

$C=\pi d$

For your data, this means,

$C=12\pi$

Now, to compute the angle, note that you have a percentage of the total circumference, based upon your arc length:

$\frac{13.5}{12\pi}*360=128.9155039044336$

Rounded to the nearest hundredth, this is $128.92^{\circ}$ .

Report an Error

Example Question #6 : How To Find The Angle Of A Sector

Inscribed angle

Figure NOT drawn to scale.

Refer to the above diagram. $\overarc {ABC}$ is a semicircle. Evaluate $m \overarc {AB}$ .

Possible Answers:

$55^{\circ }$

$77 ^{\circ }$

$66 ^{\circ }$

$88^{\circ }$

Insufficient information is given to answer the question.

Correct answer:

$66 ^{\circ }$

Explanation:

An inscribed angle of a circle that intercepts a semicircle is a right angle; therefore, $\angle B$ , which intercepts the semicircle $\overarc{ABC}$ , is such an angle. Consequently, $m \angle B = 90 ^{\circ }$ , and $\bigtriangleup ABC$ is a right triangle. The acute angles of $\bigtriangleup ABC$ are complementary, so

$m \angle A + m \angle C = 90 ^{\circ }$

(7t+1 )+ (4t+1) = 90

7t+4t+1+1 = 90

11t+2 = 90

11t+2 -2 = 90 - 2

11t = 88

$11t\div 11 = 88 \div 11$

t = 8

The measure of inscribed $\angle C$ is

$m \angle C = (4t+1 )^{\circ } = (4 \cdot 8+1 )^{\circ } = 33 ^{\circ }$ .

An inscribed angle of a circle intercepts an arc of twice its degree measure, so

$m \overarc {AB} = 2 \cdot m \angle C= 2 \cdot 33^{\circ } = 66 ^{\circ }$ .

Report an Error

Example Question #271 : Sat Mathematics

Secant 2 Figure NOT drawn to scale

Refer to the above figure. $\overline{AB}$ is a diameter of the circle. Evaluate .

Possible Answers:

t = 34

t = 56

t = 17

t = 68

t = 28

Correct answer:

t = 56

Explanation:

$\overline{AB}$ is a diameter, so $\overarc{BC A }$ is a semicircle, and

$m \overarc{BC A } = 180^{\circ }$ ,

or, equivalently,

$m \overarc{BC} + m \overarc {AC } = 180^{\circ }$

In terms of , since $m \overarc {AC } = t^{\circ }$ ,

$m \overarc{BC} + t ^{\circ }= 180^{\circ }$

$m \overarc{BC} + t^{\circ } - t ^{\circ }= 180 ^{\circ } - t^{\circ }$

$m \overarc{BC} =( 180 - t)^{\circ }$

$\overline{NB}$ and $\overline{NC }$ , being a secant segment and a tangent segment to a circle, respectively, intercept two arcs such that the measure of the angle that the segments form is equal to one-half the difference of the measures of the intercepted arcs - that is,

$\frac{1}{2} (m \overarc{BC} - m \overarc {AC }) = m \angle CNB$

Setting $m \overarc{BC} = \left (180 - t \right )^{\circ }$ , $m \overarc {AC } = t^{\circ }$ , and $m \angle CNB = 34^{\circ }$ :

$\frac{1}{2}\left [ (180 - t) - t \right ]= 34$

$\frac{1}{2} (180 - 2t) = 34$

$\frac{1}{2} \cdot 180 -\frac{1}{2} \cdot 2t = 34$

90 - t= 34

90-t-90 = 34 -90

-t =- 56

t = 56

Report an Error

Example Question #31 : Circles

Secant

Refer to the above diagram. Evaluate the measure of $\angle BND$ .

Possible Answers:

$37 \frac{1}{2}^{\circ }$

$52 \frac{1}{2}^{\circ }$

$60^{\circ }$

$30^{\circ }$

$45 ^{\circ }$

Correct answer:

$45 ^{\circ }$

Explanation:

The total measure of the arcs that comprise a circle is $360 ^{\circ }$ , so from the above diagram,

$m \overarc { AC}+m \overarc { CD}+ m \overarc { DB} + m \overarc {BA } = 360 ^{\circ }$

Substituting the appropriate expression for each arc measure:

t+t+3t+3t = 360

8t = 360

$8t \div 8 = 360 \div 8$

t = 45

Therefore,

$m \overarc { AC}= 45^{\circ }$

and

$m \overarc { DB} = 3 t^{\circ } = 3 \cdot 45^{\circ } = 135^{\circ }$

The measure of the angle formed by the tangent segments $\overline{NB}$ and $\overline{ND}$ , which is $\angle BND$ , is half the difference of the measures of the arcs they intercept, so

$m\angle BND = \frac{1}{2} (m \overarc {DB} - m \overarc {AC})$

Substituting:

$m\angle BND = \frac{1}{2} (135 ^{\circ }- 45^{\circ } )$

$m\angle BND = \frac{1}{2} (90^{\circ } )$

$m\angle BND = 45 ^{\circ }$

Report an Error

Example Question #11 : How To Find The Angle Of A Sector

Inscribed quad

Figure NOT drawn to scale.

The above figure shows a quadrilateral inscribed in a circle. Evaluate .

Possible Answers:

The question cannot be answered from the information given.

u= 102

u= 96

u= 88

u= 92

Correct answer:

u= 92

Explanation:

If a quadrilateral is inscribed in a circle, then each pair of its opposite angles are supplementary - that is, their degree measures total $180^{\circ }$ .

$\angle B$ and $\angle D$ are two such angles, so

$m \angle B + m \angle D = 180 ^{\circ }$

Setting $m \angle B = 88^{\circ }$ and $m \angle D =u ^{\circ }$ , and solving for :

88+ u = 180

88+ u - 88 = 180 - 88

u= 92 ,

the correct response.

Report an Error

Example Question #281 : Plane Geometry

Inscribed quad

Figure NOT drawn to scale.

The above figure shows a quadrilateral inscribed in a circle. Evaluate .

Possible Answers:

The question cannot be answered from the information given.

Correct answer:

The question cannot be answered from the information given.

Explanation:

If a quadrilateral is inscribed in a circle, then each pair of its opposite angles are supplementary - that is, their degree measures total $180^{\circ }$ .

$\angle A$ and $\angle C$ are two such angles, so

$m \angle A + m \angle C= 180 ^{\circ }$

Setting $m \angle A = t^{\circ }$ and $m \angle C = (180-t)^{\circ }$ , and solving for :

t + (180-t)= 180

180+t-t= 180

180= 180 ,

The statement turns out to be true regardless of the value of . Therefore, without further information, the value of cannot be determined.

Report an Error

Example Question #281 : Sat Mathematics

Inscribed quad

Figure NOT drawn to scale.

The above figure shows a quadrilateral inscribed in a circle. Evaluate .

Possible Answers:

t= 78

t= 84

t = 80

t = 82

t = 86

Correct answer:

t= 84

Explanation:

If a quadrilateral is inscribed in a circle, then each pair of its opposite angles are supplementary - that is, their degree measures total $180^{\circ }$ .

$\angle A$ and $\angle C$ are two such angles, so

$m \angle A + m \angle C= 180 ^{\circ }$

Setting $m \angle A = t^{\circ }$ and $m \angle C = (t+12)^{\circ }$ , and solving for :

t + (t+12 )= 180

2t +12 = 180

2t +12 - 12 = 180 - 12

2t = 168

$2t \div 2 = 168 \div 2$

t = 84 ,

the correct response.

Report an Error

Example Question #11 : How To Find The Angle Of A Sector

Secant 2

Figure NOT drawn to scale.

Refer to the above diagram. $\overline{AB}$ is a diameter. Evaluate $m \angle CBA$

Possible Answers:

$28^{\circ }$

$62^{\circ }$

$45^{\circ }$

$31^{\circ }$

$56^{\circ }$

Correct answer:

$31^{\circ }$

Explanation:

$\overline{AB}$ is a diameter, so $\overarc{ACB}$ is a semicircle - therefore, $m \overarc{ACB} = 180 ^{\circ }$ . By the Arc Addition Principle,

$m \overarc{AC}+ m \overarc{CB} = 180 ^{\circ }$

If we let $t ^{\circ }= m \overarc{AC}$ , then

$t ^{\circ }+ m \overarc{CB} = 180 ^{\circ }$ ,

and

$m \overarc{CB} = (180 -t )^{\circ }$

If a secant and a tangent are drawn from a point to a circle, the measure of the angle they form is half the difference of the measures of the intercepted arcs. Since $\overline{NC}$ and $\overline{NB}$ are such segments intercepting $\overarc{AC}$ and $\overarc{CB}$ , it holds that

$\frac{1}{2}\left ( m \overarc{CB}- m \overarc{AC} \right ) = m \angle CNB$

Setting $m \overarc{AC} = t ^{\circ }$ , $m \overarc{CB} = (180 -t )^{\circ }$ , and $m \angle CNB = 28 ^{\circ }$ :

$\frac{1}{2} [ \left ( 180-t \right ) - t ] = 28$

$\frac{1}{2} \left ( 180-2 t \right ) = 28$

90-t = 28

90-t + t - 28 = 28 + t - 28

62 = t

$m \overarc{AC} =62^{\circ }$

The inscribed angle that intercepts this arc, $\angle CBA$ , has half this measure:

$m \angle CBA = \frac{1}{2} \cdot m \overarc{AC} = \frac{1}{2} \cdot 62^{\circ } = 31^{\circ }$ .

This is the correct response.

Report an Error

Example Question #12 : How To Find The Angle Of A Sector

Secant 3 Figure NOT drawn to scale.

In the above figure, $\overline{AB}$ is a diameter. Also, the ratio of the length of $\overarc{BT}$ to that of $\overarc{AT}$ is 7 to 5. Give the measure of $\angle TNA$ .

Possible Answers:

$21 ^{\circ }$

$15^{\circ }$

$24 ^{\circ }$

$18^{\circ }$

The measure of $\angle TNA$ cannot be determine from the information given.

Correct answer:

$15^{\circ }$

Explanation:

$\overline{AB}$ is a diameter, so $\overarc {ATB}$ is a semicircle, which has measure $180 ^{\circ }$ . By the Arc Addition Principle,

$m \overarc {AT } + m \overarc {BT} = m \overarc {ATB}$

If we let $t ^{\circ }= m \overarc {AT }$ , then, substituting:

$t ^{\circ }+ m \overarc {BT} = 180 ^{\circ }$ ,

and

$m \overarc {BT} =( 180 - t ) ^{\circ }$

the ratio of the length of $\overarc{BT}$ to that of $\overarc{AT}$ is 7 to 5; this is also the ratio of their degree measures; that is,

$\frac{m \overarc{BT}}{m \overarc{AT}} = \frac{5}{3}$

Setting $m \overarc {AT } = t ^{\circ }$ and $m \overarc {BT} =( 180 - t ) ^{\circ }$ :

$\frac{180-t }{t} = \frac{7}{5}$

Cross-multiply, then solve for :

7t = 5 (180-t)

7t = 900 - 5t

7t + 5t = 900 - 5t + 5t

12t = 900

$\frac{12t }{12}= \frac{900}{12}$

t = 75

$m \overarc {AT } =75^{\circ }$ , and $m \overarc {BT} =( 180 -75 ) ^{\circ } = 105 ^{\circ }$

If a secant and a tangent are drawn from a point to a circle, the measure of the angle they form is half the difference of the measures of the intercepted arcs. Since $\overline{NB}$ and $\overline{NT}$ are such segments whose angle $\angle TNA$ intercepts $\overarc{AT}$ and $\overarc{BT}$ , it holds that:

$m \angle TNA = \frac{1}{2}\left ( m \overarc{BT}- m \overarc{AT} \right )$

$m \angle TNA = \frac{1}{2}\left ( 105 ^{\circ } - 75^{\circ } \right )$

$m \angle TNA = \frac{1}{2}\left ( 30 ^{\circ } \right )$

$m \angle TNA = 15^{\circ }$

Report an Error

Example Question #31 : Circles

If the area of a circle is four times larger than the circumference of that same circle, what is the diameter of the circle?

Possible Answers:

Correct answer:

Explanation:

Set the area of the circle equal to four times the circumference πr² = 4(2πr).

Cross out both π symbols and one r on each side leaves you with r = 4(2) so r = 8 and therefore d = 16.

Report an Error

View SAT Mathematics Tutors

Joy
Certified Tutor

Boston University, Bachelor in Arts, Conservation Biology.

View SAT Mathematics Tutors

Thomas
Certified Tutor

Michigan State University, Bachelor in Arts, History. Central Michigan University, Doctor of Philosophy, History.

View SAT Mathematics Tutors

Kelly
Certified Tutor

CUNY Hunter College, Bachelor in Arts, Economics.

All SAT Math Resources

16 Diagnostic Tests 660 Practice Tests Question of the Day Flashcards Learn by Concept

SAT Math Tutors in Top Cities:

Atlanta SAT Math Tutors, Austin SAT Math Tutors, Boston SAT Math Tutors, Chicago SAT Math Tutors, Dallas Fort Worth SAT Math Tutors, Denver SAT Math Tutors, Houston SAT Math Tutors, Kansas City SAT Math Tutors, Los Angeles SAT Math Tutors, Miami SAT Math Tutors, New York City SAT Math Tutors, Philadelphia SAT Math Tutors, Phoenix SAT Math Tutors, San Diego SAT Math Tutors, San Francisco-Bay Area SAT Math Tutors, Seattle SAT Math Tutors, St. Louis SAT Math Tutors, Tucson SAT Math Tutors, Washington DC SAT Math Tutors

Popular Courses & Classes

Spanish Courses & Classes in Miami, GRE Courses & Classes in Philadelphia, ACT Courses & Classes in Washington DC, GMAT Courses & Classes in Dallas Fort Worth, LSAT Courses & Classes in Chicago, SSAT Courses & Classes in San Diego, GMAT Courses & Classes in Atlanta, ACT Courses & Classes in Denver, GMAT Courses & Classes in Denver, LSAT Courses & Classes in Phoenix

Popular Test Prep

GRE Test Prep in Dallas Fort Worth, ACT Test Prep in Philadelphia, MCAT Test Prep in New York City, SAT Test Prep in New York City, ACT Test Prep in Denver, MCAT Test Prep in Houston, MCAT Test Prep in San Francisco-Bay Area, ACT Test Prep in San Diego, ACT Test Prep in Phoenix, GRE Test Prep in Houston

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

SAT Math : Circles

Study concepts, example questions & explanations for SAT Math

All SAT Math Resources

Example Questions

Example Question #21 : Circles

Example Question #6 : How To Find The Angle Of A Sector

Example Question #271 : Sat Mathematics

Example Question #31 : Circles

Example Question #11 : How To Find The Angle Of A Sector

Example Question #281 : Plane Geometry

Example Question #281 : Sat Mathematics

Example Question #11 : How To Find The Angle Of A Sector

Example Question #12 : How To Find The Angle Of A Sector

Example Question #31 : Circles

All SAT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: