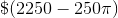Call Now to Set Up Tutoring:

(888) 888-0446

SAT Math : Circles

Study concepts, example questions & explanations for SAT Math

Create An Account

All SAT Math Resources

16 Diagnostic Tests 660 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #3 : How To Find The Angle Of Clock Hands

What is the measure, in degrees, of the acute angle formed by the hands of a 12-hour clock that reads exactly 3:10?

Possible Answers:

35°

60°

72°

65°

55°

Correct answer:

35°

Explanation:

The entire clock measures 360°. As the clock is divided into 12 sections, the distance between each number is equivalent to 30° (360/12). The distance between the 2 and the 3 on the clock is 30°. One has to account, however, for the 10 minutes that have passed. 10 minutes is 1/6 of an hour so the hour hand has also moved 1/6 of the distance between the 3 and the 4, which adds 5° (1/6 of 30°). The total measure of the angle, therefore, is 35°.

Report an Error

Example Question #1 : How To Find The Angle Of Clock Hands

If it is 4:00, what is the measure of the angle between the minute and hour hands of the clock?

Possible Answers:

120 degrees

45 degrees

100 degrees

90 degrees

125 degrees

Correct answer:

120 degrees

Explanation:

A clock takes the shape of a circle, which is composed of 360 degrees. There are 12 numbers on a clock that represent the hours. With this in mind, we can say that each number represents an angle. The measure of the angle between each number is given by $360\div12=30$ .

If it is 4:00, then the minute hand is pointing towards 12 while the hour hand points towards 4.

Therefore, we can say that the angle between the two hands is $30\cdot4=120$ degrees.

Another way to think of this is to imagine the clock at a nearby time. At 3:00, the hands of the clock form a right angle of 90 degrees. Since we know that each number on the clock is separated by 30 degrees, we can simply add 30 to 90 degrees and get 120 degrees for the angle at 4:00.

Report an Error

Example Question #1 : How To Find The Angle Of Clock Hands

If it is 2:00 PM, what is the measure of the angle between the minute and hour hands of the clock?

Possible Answers:

60 degrees

120 degrees

90 degrees

30 degrees

45 degrees

Correct answer:

60 degrees

Explanation:

First note that a clock is a circle made of 360 degrees, and that each number represents an angle and the separation between them is 360/12 = 30. And at 2:00, the minute hand is on the 12 and the hour hand is on the 2. The correct answer is 2 * 30 = 60 degrees.

Report an Error

Example Question #1 : Circles

Two pizzas are made to the same dimensions. The only difference is that Pizza 1 is cut into pieces at 30° angles and Pizza 2 is cut at 45° angles. They are sold by the piece, the first for $1.95 per slice and the second for $2.25 per slice. What is the difference in total revenue between Pizza 2 and Pizza 1?

Possible Answers:

–$2.70

–$5.40

$5.40

$2.70

Correct answer:

–$5.40

Explanation:

First, let's calculate how many slices there are per pizza. This is done by dividing 360° by the respective slice degrees:

Pizza 1: 360/30 = 12 slices

Pizza 2: 360/45 = 8 slices

Now, the total amount made per pizza is calculated by multiplying the number of slices by the respective cost per slice:

Pizza 1: 12 * 1.95 = $23.40

Pizza 2: 8 * 2.25 = $18.00

The difference between Pizza 2 and Pizza 1 is thus represented by: 18 – 23.40 = –$5.40

Report an Error

Example Question #1 : Plane Geometry

A circular, 8-slice pizza is placed in a square box that has dimensions four inches larger than the diameter of the pizza. If the box covers a surface area of 256 in², what is the surface area of one piece of pizza?

Possible Answers:

18π in²

144π in²

4.5π in²

36π in²

9π in²

Correct answer:

4.5π in²

Explanation:

The first thing to do is calculate the dimensions of the pizza box. Based on our data, we know 256 = s². Solving for s (by taking the square root of both sides), we get 16 = s (or s = 16).

Now, we know that the diameter of the pizza is four inches less than 16 inches. That is, it is 12 inches. Be careful! The area of the circle is given in terms of radius, which is half the diameter, or 6 inches. Therefore, the area of the pizza is π * 6² = 36π in². If the pizza is 8-slices, one slice is equal to 1/8 of the total pizza or (36π)/8 = 4.5π in².

Report an Error

Example Question #1 : How To Find The Area Of A Sector

If B is a circle with line AC = 12 and line BC = 16, then what is the area formed by DBE?

Possible Answers:

$200$

$256\pi$

$100\pi$

$144$

$5\pi$

Correct answer:

$100\pi$

Explanation:

Line AB is a radius of Circle B, which can be found using the Pythagorean Theorem:

$AB^2=AC^2+BC^2\rightarrow AB=\sqrt{AC^2+BC^2}=\sqrt{16^2+12^2}=\sqrt{400}=20$

Since AB is a radius of B, we can find the area of circle B via:

$Area=\pi R^2=\pi(20^2)=400\pi$

Angle DBE is a right angle, and therefore $\frac{1}{4}$ of the circle so it follows:

$Area(DBE)=\frac{400}{4}\pi=100\pi$

Report an Error

Example Question #1 : How To Find The Area Of A Sector

Circle

The radius of the circle above is and $\angle A=45^{\circ}$ . What is the area of the shaded section of the circle?

Possible Answers:

$4\pi$

$\pi$

$2\pi$

$8\pi$

$16\pi$

Correct answer:

$2\pi$

Explanation:

Area of Circle = πr² = π4²= 16π

Total degrees in a circle = 360

Therefore 45 degree slice = 45/360 fraction of circle = 1/8

Shaded Area = 1/8 * Total Area = 1/8 * 16π = 2π

Report an Error

Example Question #1 : How To Find The Area Of A Sector

Square-missing

SRAD is a square.

$\overline{AB}=\overline{BC}=\overline{CD}$

The arc from to is a semicircle with a center at the midpoint of $\overline{BC}$ .

All units are in feet.

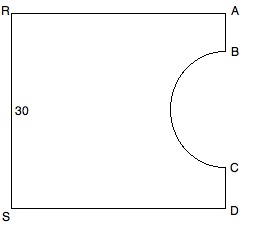
The diagram shows a plot of land.

The cost of summer upkeep is $2.50 per square foot.

In dollars, what is the total upkeep cost for the summer?

Possible Answers:

$\$(2250-31.25\pi )$

$\$900$

$\$(2250-250\pi )$

$\$(900-12.5\pi )$

$\$(900-25\pi )$

Correct answer:

$\$(2250-31.25\pi )$

Explanation:

To solve this, we must begin by finding the area of the diagram, which is the area of the square less the area of the semicircle.

The area of the square is straightforward:

30 * 30 = 900 square feet

Because each side is 30 feet long, AB + BC + CD = 30.

We can substitute BC for AB and CD since all three lengths are the same:

BC + BC + BC = 30

3BC = 30

BC = 10

Therefore the diameter of the semicircle is 10 feet, so the radius is 5 feet.

The area of the semi-circle is half the area of a circle with radius 5. The area of the full circle is 5²π = 25π, so the area of the semi-circle is half of that, or 12.5π.

The total area of the plot is the square less the semicircle: 900 - 12.5π square feet

The cost of upkeep is therefore 2.5 * (900 – 12.5π) = $(2250 – 31.25π).

Report an Error

Example Question #2 : How To Find The Area Of A Sector

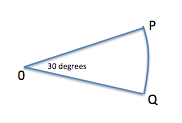
In the figure, PQ is the arc of a circle with center O. If the area of the sector is $3\pi$ what is the perimeter of sector?

Possible Answers:

$3 + 2\pi$

$12 + \pi$

$6 + \pi$

$12 + 2\pi$

$1 + \pi$

Correct answer:

$12 + \pi$

Explanation:

First, we figure out what fraction of the circle is contained in sector OPQ: $\frac{30^{\circ}}{360^{\circ}}= \frac{1}{12}$ , so the total area of the circle is $\dpi{100} \small 12\times 3\pi=36$ .

Using the formula for the area of a circle, ${\pi}r^{2}$ , we can see that $\dpi{100} \small r=6$ .

We can use this to solve for the circumference of the circle, $2{\pi}r$ , or $12{\pi}$ .

Now, OP and OQ are both equal to r, and PQ is equal to $\dpi{100} \small \frac{1}{12}$ of the circumference of the circle, or ${\pi}$ .

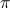
To get the perimeter, we add OP + OQ + PQ, which give us $12+{\pi}$ .

Report an Error

Example Question #1 : How To Find The Area Of A Sector

A central angle of a circle measures 60 degrees. If its corresponding arc measures 3 units, what is the area of the circle?

Possible Answers:

$18\pi$

$\frac{81}{\pi }$

$\frac{9}{\pi}$

$9\pi$

Correct answer:

$\frac{81}{\pi }$

Explanation:

If the central angle measures 60 degrees, divide the 360 total degrees in the circle by 60.

$\frac{360}{60}=6$

Multiply this by the measure of the corresponding arc to find the total circumference of the circle.

$3\times 6 =18$

Use the circumference to find the radius, then use the radius to find the area.

$C=2\pi r$

$18=2\pi r$

$\frac{18}{2\pi}=r$

$\frac{9}{\pi}=r$

$A=\pi r^2$

$A=\pi\times (\frac{9}{\pi})^2$

$A= \pi \times \frac{81}{\pi^2}$

$A = \frac{81\pi}{\pi^2}$

$A=\frac{81}{\pi}$

Report an Error

View SAT Mathematics Tutors

Joy
Certified Tutor

Boston University, Bachelor in Arts, Conservation Biology.

View SAT Mathematics Tutors

Sharif
Certified Tutor

NCCU, Bachelors, Physics and Mathematics.

View SAT Mathematics Tutors

Miriam
Certified Tutor

Washington University in St Louis, Bachelor in Arts, Biology, General.

All SAT Math Resources

16 Diagnostic Tests 660 Practice Tests Question of the Day Flashcards Learn by Concept

SAT Math Tutors in Top Cities:

Atlanta SAT Math Tutors, Austin SAT Math Tutors, Boston SAT Math Tutors, Chicago SAT Math Tutors, Dallas Fort Worth SAT Math Tutors, Denver SAT Math Tutors, Houston SAT Math Tutors, Kansas City SAT Math Tutors, Los Angeles SAT Math Tutors, Miami SAT Math Tutors, New York City SAT Math Tutors, Philadelphia SAT Math Tutors, Phoenix SAT Math Tutors, San Diego SAT Math Tutors, San Francisco-Bay Area SAT Math Tutors, Seattle SAT Math Tutors, St. Louis SAT Math Tutors, Tucson SAT Math Tutors, Washington DC SAT Math Tutors

Popular Courses & Classes

SSAT Courses & Classes in Houston, LSAT Courses & Classes in Los Angeles, LSAT Courses & Classes in Seattle, SSAT Courses & Classes in San Diego, ISEE Courses & Classes in Phoenix, ISEE Courses & Classes in Miami, GMAT Courses & Classes in Los Angeles, SSAT Courses & Classes in Phoenix, GMAT Courses & Classes in Phoenix, GRE Courses & Classes in Phoenix

Popular Test Prep

GMAT Test Prep in Seattle, MCAT Test Prep in Washington DC, ISEE Test Prep in Dallas Fort Worth, ISEE Test Prep in Philadelphia, SAT Test Prep in San Diego, GMAT Test Prep in Phoenix, ISEE Test Prep in Miami, ISEE Test Prep in Houston, GRE Test Prep in Washington DC, GRE Test Prep in New York City

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

SAT Math : Circles

Study concepts, example questions & explanations for SAT Math

All SAT Math Resources

Example Questions

Example Question #3 : How To Find The Angle Of Clock Hands

Example Question #1 : How To Find The Angle Of Clock Hands

Example Question #1 : How To Find The Angle Of Clock Hands

Example Question #1 : Circles

Example Question #1 : Plane Geometry

Example Question #1 : How To Find The Area Of A Sector

Example Question #1 : How To Find The Area Of A Sector

Example Question #1 : How To Find The Area Of A Sector

Example Question #2 : How To Find The Area Of A Sector

Example Question #1 : How To Find The Area Of A Sector

All SAT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: