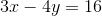Let and

The slope is geven by:    so

Then we use the slope-intercept form of an equation;   so

And we convert 

to standard form.

In order to find the equation of the line, we will first need to find the slope between the two points through which it passes. The slope, , of a line that passes through the points and is given by the formula below:

We are given our two points, (4,7) and (8,10), allowing us to calculate the slope.

Next, we can use point slope form to find the equation of a line with this slope that passes through one of the given points. We will use (4,7).

Multiply both sides by four to eliminate the fraction, and simplify by distribution.

Subtract from both sides and add twelve to both sides.

This gives our final answer:

First, solve for slope.

Then, substitute one of the points into the equation y=mx+b.

This leaves us with the equation

First, we need to find the slope of the above line. 

The slope of a line. given two points  can be calculated using the slope formula

Set :

Second, we note that the -intercept is the point .

Therefore, in the slope-intercept form of a line, we can set  and :

Since we are looking for standard form - that is,  - we do the following:

or 

First, we need to find the slope of the above line. 

The slope of a line. given two points  can be calculated using the slope formula

Set :

Second, we note that the -intercept is the point .

Therefore, in the slope-intercept form of a line, we can set  and :

First, we need to find the slope of the above line. 

The slope of a line. given two points  can be calculated using the slope formula

Set :

The slope of a line perpendicular to it has as its slope the opposite of the reciprocal of 3, which is .

Since we want the line to have the same -intercept as the above line, which is the point , we can use the slope-intercept form to help us.  We set 

, and solve for  in:

Substitute for  and  in the slope-intercept form, and the equation is

Based on the graph the y-intercept is 4. So we can eliminate choice y = x/2 - 4.

The graph is rising to the right which means our slope is positive, so we can eliminate choice y = -1/2x + 4.

Based on the line, if we start at (0,4) and go up 1 then 2 to the right we will be back on the line, meaning we have a slope of  (1/2).

Using the slope intercept formula we can plug in y= (1/2)x + 4.

The equation of line l relates x -values and y -values that lie along the line. The question is asking for the x -value of a point on the line whose y -value is 1, so we are looking for the x -value on the line when the y-value is 1. In the equation of the line, plug 1 in for y  and solve for x:

2x - 3(1) = 5

2x - 3 = 5

2x = 8

x = 4. So the missing x-value on line l is 4.

Test the difference combinations out starting with the most repeated number.  In this case, y = 7 appears most often in the answers.  Plug in y=7 and solve for x.  If the answer does not appear on the list, solve for the next most common coordinate.

2(x) + 9(7) = 71

2x + 63 = 71

2x = 8

x = 4

Therefore the answer is (4, 7)

In order to find out which of these lines is correct, we simply plug in the values  and  into each equation and see if it balances.

The only one for which this will work is