Call Now to Set Up Tutoring:

(888) 888-0446

PSAT Math : Algebra

Study concepts, example questions & explanations for PSAT Math

Create An Account

All PSAT Math Resources

10 Diagnostic Tests 421 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #434 : Algebra

If $x+1< 4$ and $y-2<-1$ , then which of the following could be the value of x+y ?

Possible Answers:

Correct answer:

Explanation:

To solve this problem, add the two equations together:

$x+1<4$

$y-2<-1$

$x+1+y-2<4-1$

$x+y-1<3$

$x+y<4$

The only answer choice that satisfies this equation is 0, because 0 is less than 4.

Report an Error

Example Question #1 : How To Find The Solution To An Inequality With Addition

If 17-x>19 , which of the following could be a value of ?

Possible Answers:

Correct answer:

Explanation:

In order to solve this inequality, you must isolate on one side of the equation.

17-x>19

17 > 19 +x

-2 > x

Therefore, the only option that solves the inequality is .

Report an Error

Example Question #141 : Algebra

What values of make the statement $\left |5x-9 \right |\geq6$ true?

Possible Answers:

$x\geq4,x\leq -\frac{1}{2}$

$x\geq3, x\leq \frac{3}{5}$

$x\geq5,x\leq \frac{1}{5}$

$x\geq15,x\leq \frac{2}{5}$

$x\geq6,x\leq \frac{1}{3}$

Correct answer:

$x\geq3, x\leq \frac{3}{5}$

Explanation:

First, solve the inequality $5x-9 \geq6$ :

$5x-9 \geq6$

$5x\geq15$

$x\geq3$

Since we are dealing with absolute value, $5x-9\leq-6$ must also be true; therefore:

$5x-9\leq-6$

$5x\leq3$

$x\leq \frac{3}{5}$

Report an Error

Example Question #52 : Equations / Inequalities

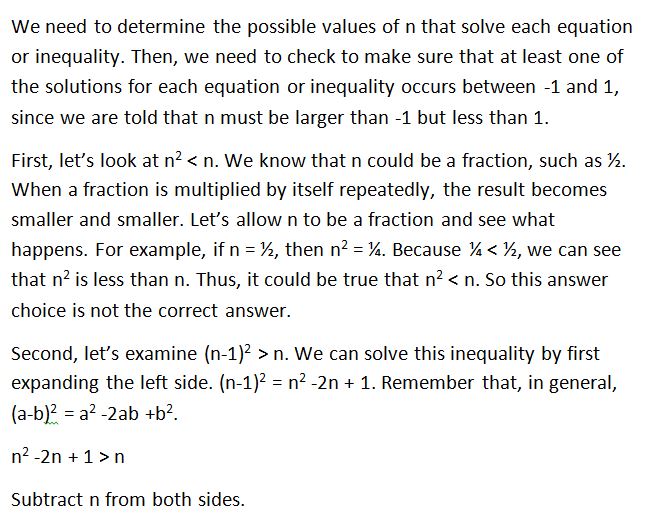
If –1 < n < 1, all of the following could be true EXCEPT:

Possible Answers:

(n-1)² > n

n² < 2n

16n² - 1 = 0

|n² - 1| > 1

n² < n

Correct answer:

|n² - 1| > 1

Explanation:

N_part_1

N_part_2

N_part_3

N_part_4

N_part_5

Report an Error

Example Question #53 : Equations / Inequalities

(√(8) / -x ) < 2. Which of the following values could be x?

Possible Answers:

-2

-1

-3

All of the answers choices are valid.

-4

Correct answer:

-1

Explanation:

The equation simplifies to x > -1.41. -1 is the answer.

Report an Error

Example Question #54 : Equations / Inequalities

Solve for x

$\small 3x+7 \geq -2x+4$

Possible Answers:

$\small x \geq -\frac{3}{5}$

$\small x \leq -\frac{3}{5}$

$\small x \geq \frac{3}{5}$

$\small x \leq \frac{3}{5}$

Correct answer:

$\small x \geq -\frac{3}{5}$

Explanation:

$\small 3x+7 \geq -2x+4$

$\small 3x \geq -2x-3$

$\small 5x \geq -3$

$\small x\geq -\frac{3}{5}$

Report an Error

Example Question #55 : Equations / Inequalities

We have x^2-4<0 , find the solution set for this inequality.

Possible Answers:

x<2

x>2, x<-2

x=0

x>-2

-2<x<2

Correct answer:

-2<x<2

Explanation:

$x^2-4<0 \Rightarrow x^2<4 \Rightarrow -2<x<2$

Report an Error

Example Question #25 : Inequalities

Fill in the circle with either $<$ , $>$ , or $=$ symbols:

$(x-3)\circ\frac{x^2-9}{x+3}$ for $x\geq 3$ .

Possible Answers:

None of the other answers are correct.

$(x-3)> \frac{x^2-9}{x+3}$

The rational expression is undefined.

$(x-3)=\frac{x^2-9}{x+3}$

$(x-3)< \frac{x^2-9}{x+3}$

Correct answer:

$(x-3)=\frac{x^2-9}{x+3}$

Explanation:

$(x-3)\circ\frac{x^2-9}{x+3}$

Let us simplify the second expression. We know that:

$(x^2-9)=(x+3)(x-3)$

So we can cancel out as follows:

$\frac{x^2-9}{x+3}=\frac{(x+3)(x-3)}{(x+3)}=x-3$

$(x-3)=\frac{x^2-9}{x+3}$

Report an Error

Example Question #21 : Inequalities

What is the greatest value of that makes

$-45 \leq \frac{x - 7}{- 6} \leq - 22$

a true statement?

Possible Answers:

228

277

Correct answer:

277

Explanation:

Find the solution set of the three-part inequality as follows:

$-45 \leq \frac{x - 7}{- 6} \leq - 22$

$-45 \cdot (-6) \geq \frac{x - 7}{- 6} \cdot (-6) \geq - 22 \cdot (-6)$

$270 \geq x-7 \geq 132$

$270 + 7 \geq x-7 + 7 \geq 132 + 7$

$27 7 \geq x \geq 139$

The greatest possible value of is the upper bound of the solution set, which is 277.

Report an Error

Example Question #5 : How To Find The Solution To An Inequality With Multiplication

What is the least value of that makes

$-45 \leq \frac{x - 7}{- 6} \leq - 22$

a true statement?

Possible Answers:

139

125

Correct answer:

139

Explanation:

Find the solution set of the three-part inequality as follows:

$-45 \leq \frac{x - 7}{- 6} \leq - 22$

$-45 \cdot (-6) \geq \frac{x - 7}{- 6} \cdot (-6) \geq - 22 \cdot (-6)$

$270 \geq x-7 \geq 132$

$270 + 7 \geq x-7 + 7 \geq 132 + 7$

$27 7 \geq x \geq 139$

The least possible value of is the lower bound of the solution set, which is 139.

Report an Error

View PSAT Mathematics Tutors

Troy
Certified Tutor

Rice University, Bachelor in Arts, Kinesiology.

View PSAT Mathematics Tutors

Mike Bahaa
Certified Tutor

Concordia University-Ann Arbor, Master of Arts Teaching, Mathematics Teacher Education.

View PSAT Mathematics Tutors

Darian
Certified Tutor

University of North Texas, Current Undergrad Student, English.

All PSAT Math Resources

10 Diagnostic Tests 421 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

ISEE Tutors in San Francisco-Bay Area, LSAT Tutors in San Diego, SAT Tutors in Washington DC, Chemistry Tutors in Houston, Calculus Tutors in San Diego, MCAT Tutors in Miami, LSAT Tutors in Chicago, French Tutors in Miami, French Tutors in Atlanta, Statistics Tutors in Washington DC

Popular Courses & Classes

Spanish Courses & Classes in Atlanta, GRE Courses & Classes in Washington DC, MCAT Courses & Classes in Los Angeles, SSAT Courses & Classes in Dallas Fort Worth, SAT Courses & Classes in Chicago, LSAT Courses & Classes in Los Angeles, LSAT Courses & Classes in Miami, ISEE Courses & Classes in San Francisco-Bay Area, Spanish Courses & Classes in Dallas Fort Worth, LSAT Courses & Classes in Houston

Popular Test Prep

MCAT Test Prep in Philadelphia, MCAT Test Prep in Los Angeles, SAT Test Prep in Houston, LSAT Test Prep in Chicago, LSAT Test Prep in Philadelphia, SAT Test Prep in New York City, GMAT Test Prep in Atlanta, ISEE Test Prep in Houston, ISEE Test Prep in Chicago, SAT Test Prep in Boston

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

PSAT Math : Algebra

Study concepts, example questions & explanations for PSAT Math

All PSAT Math Resources

Example Questions

Example Question #434 : Algebra

Example Question #1 : How To Find The Solution To An Inequality With Addition

Example Question #141 : Algebra

Example Question #52 : Equations / Inequalities

Example Question #53 : Equations / Inequalities

Example Question #54 : Equations / Inequalities

Example Question #55 : Equations / Inequalities

Example Question #25 : Inequalities

Example Question #21 : Inequalities

Example Question #5 : How To Find The Solution To An Inequality With Multiplication

All PSAT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: