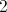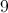To find the point where these two lines intersect, set the equations equal to each other, such that  is substituted with the  side of the second equation. Solving this new equation for  will give the -coordinate of the point of intersection.

Subtract from both sides.

Divide both sides by 2.

Now substitute  into either equation to find the -coordinate of the point of intersection.

With both coordinates, we know the point of intersection is . One can plug in  for  and  for  in both equations to verify that this is correct.

Add the equations together.

Put the terms together to see that .

Substitute this value into one of the original equaitons and solve for .

Now we know that , thus we can find the sum of and .

We add the two systems of equations:

For the Left Hand Side:

For the Right Hand Side:

So our resulting equation is:

Divide both sides by 10:

For the Left Hand Side:

For the Right Hand Side:

Our result is:

Reduce the second system by dividing by 3.

Second Equation:

     We this by 3.

Then we subtract the first equation from our new equation.

First Equation:

First Equation - Second Equation:

Left Hand Side:

Right Hand Side:

Our result is:

We first add both systems of equations.

Left Hand Side:

Right Hand Side:

Our resulting equation is:

We divide both sides by 3.

Left Hand Side:

Right Hand Side:

Our resulting equation is:

We first multiply the second equation by 4.

So our resulting equation is:

Then we subtract the first equation from the second new equation.

Left Hand Side:

Right Hand Side:

Resulting Equation:

We divide both sides by -15

Left Hand Side:

Right Hand Side:

Our result is:

If we multiply both sides of our bottom equation by , we get . We can now add our two equations, and eliminate , leaving only one variable. When we add the equations, we get . Therefore, . Finally, we go back to either of our equations, and plug in  so we can solve for .

Solve the second equation for , allowing us to solve using the substitution method.

Substitute for   in the first equation, and solve for .

Now, substitute for  in either equation; we will choose the second. This allows us to solve for .

Now we can write the solution in the notation , or .

Recall that with absolute values and "less than" inequalities, we have to hold the following:

12x + 3y < 15

AND

12x + 3y > –15

Otherwise written, this is:

–15 < 12x + 3y < 15

In this form, we can solve for y. First, we have to subtract x from all 3 parts of the inequality:

–15 – 12x < 3y < 15 – 12x

Now, we have to divide each element by 3:

(–15 – 12x)/3 < y < (15 – 12x)/3

This simplifies to:

–5 – 4x < y < 5 – 4x

This inequality could be rewritten as:

4x + 14 > 30  OR 4x + 14 < –30

Solve each for x:

4x + 14 > 30; 4x > 16; x > 4

4x + 14 < –30; 4x < –44; x < –11

Therefore, anything between –11 and 4 (inclusive) will not work. Hence, the answer is 7.