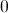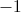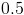We have a point at which . We know from the second derivative test that if the second derivative is negative, the function has a maximum at that point. If the second derivative is positive, the function has a minimum at that point. If the second derivative is zero, the function has an inflection point at that point.

Plug in 0 into the second derivative to obtain 

So the point is an inflection point.

Notice that on the interval , the term  is always less than or equal to . So the function is largest at the points when . This occurs at  and .

Plugging in either 1 or 0 into the original function  yields the correct answer of 0.

A cubic function will have at most one relative minimum and one relative maximum.  We can determine the zeros be factoring at .  From then we only need to determine if the graph is positive or negative in-between the zeros. 

The graph is positive between  and  (plug in ) and negative between 0 and 4 (plug in ).  This can also be seen from the graph.

The vertex form of a parabola is:

where  is the vertex of the parabola.

The function for this problem can be simplified into vetex form of a parabola: 

 ,

with a vertex at .

Since the parabola is concave up, the minimum will be at the vertex of the parabola, which is at .

To find discontinuity we need to look at where the denominator of the function is equal to zero. Looking at our function,

we need to set the denominator equal to zero and solve for :

When ,  which is undefined.

Therefore  is where the function is discontinuous.

Means to find the limit of the function as  approaches  from the left. We can see that  is a vertical asymptote therefore we need to look at numbers extremely close to zero on the left side. The value of  continues to decrease to the left of zero, with the value of  decreasing even more as  gets closer to . Thus, the limit is 

We first need to simplify the function, we can do this by factoring the numerator and denominator.

If we plug in 3 into the simplified function, we get:

Substituting  in for  we get the following:

When evaluating limits at infinity there are three rules to keep in mind:

1. If the degree of the highest exponent in the numerator is equal to the degree of the highest exponent in the denominator, then the limit is equal to the ratio of the coefficient of the highest exponent in the numerator over the coefficient of the highest exponent in the denominator. Make sure to include signs. 
2. If the degree of the highest exponent in the numerator is less than the degree of the highest exponent in the denominator, the limit = 0.
3. If the degree of the highest exponent in the numerator is greater than the degree of the higest exponent in the denominator, divide the highest power in the numerator by the highest power in the denominator and substitute for inifity. You will either subsitute for positive or negative infinity based on what the questions asks you to evaluate the limit at. 

In this case, the degree is higher in the numerator than the denominator (rule #3). Hence, you need to divide the highest powers and evaluate.

Evaluate  as x:

Answer: limit = 

When evaluating limits at infinity there are three rules to keep in mind:

1. If the degree of the highest exponent in the numerator is equal to the degree of the highest exponent in the denominator, then the limit is equal to the ratio of the coefficient of the highest exponent in the numerator over the coefficient of the highest exponent in the denominator. Make sure to include signs. 
2. If the degree of the highest exponent in the numerator is less than the degree of the highest exponent in the denominator, the limit = 0.
3. If the degree of the highest exponent in the numerator is greater than the degree of the higest exponent in the denominator, divide the highest power in the numerator by the highest power in the denominator and substitute for inifity. You will either subsitute for positive or negative infinity based on what the questions asks you to evaluate the limit at. 

In this case, the both the numerator and denominator have the highest degree of an exponent of 4 (rule #1). Hence, you need to compare the ratio of the coefficients. 

Answer: limit =