Call Now to Set Up Tutoring:

(888) 888-0446

ISEE Upper Level Math : Geometry

Study concepts, example questions & explanations for ISEE Upper Level Math

Create An Account

All ISEE Upper Level Math Resources

6 Diagnostic Tests 244 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #291 : Isee Upper Level (Grades 9 12) Mathematics Achievement

Find the area of a rhombus with one diagonal having a length of 12in and the other having a length two times the first diagonal.

Possible Answers:

$100\text{in}^2$

$288\text{in}^2$

$144\text{in}^2$

$121\text{in}^2$

$48\text{in}^2$

Correct answer:

$144\text{in}^2$

Explanation:

To find the area of a rhombus, we will use the following formula:

$A = \frac{pq}{2}$

where p and q are the lengths of the diagonals of the rhombus.

Now, we know one diagonal has a length of 12in. We also know the other diagonal is two times the first diagonal. Therefore, the second diagonal is 24in.

Knowing this, we can substitute into the formula. We get

$A = \frac{12\text{in} \cdot 24\text{in}}{2}$

$A = \frac{288\text{in}^2}{2}$

$A = 144\text{in}^2$

Report an Error

Example Question #1 : How To Find The Length Of The Side Of A Rhombus

A rhombus has a perimeter of $36\ in$ . Give the side length of the rhombus.

Possible Answers:

$9\ in$

$12\ in$

$6\ in$

$10\ in$

$8\ in$

Correct answer:

$9\ in$

Explanation:

Like any polygon, the perimeter of the rhombus is the total distance around the outside, which can be found by adding together the length of each side. In the case of a rhombus, all four sides have the same length, i.e.

Perimeter=4a ,

where is the length of each side.

$Perimeter=4a\Rightarrow 36=4a\Rightarrow a=9\ in$

Report an Error

Example Question #1 : Rhombuses

A rhombus has the area of $9\sqrt{2}\ cm^2$ . One of the interior angles is $45^{\circ}$ . Give the length of each side of the rhombus.

Possible Answers:

$2\sqrt{2}\ cm$

$3\ cm$

$3\sqrt{2}\ cm$

$2\ cm$

$3\sqrt{3}\ cm$

Correct answer:

$3\sqrt{2}\ cm$

Explanation:

The area of a rhombus can be determined by the following formula:

$Area=s^2sin\alpha$ ,

where is the length of any side and $\alpha$ is any interior angle.

$Area=s^2sin\alpha\Rightarrow 9\sqrt{2}=s^2\times sin45^{\circ}\Rightarrow 9\sqrt{2}=s^2 \times \frac{\sqrt{2}}{2}$

$\Rightarrow s^2=18\Rightarrow s=\sqrt{18}=\sqrt{9\times 2}=3\sqrt{2}\ cm$

Report an Error

Example Question #2 : Rhombuses

One side of a rhombus measures 2 yards. Which of the following is equal to the perimeter?

Possible Answers:

$18 \textrm{ ft}$

$\frac{1}{200} \textrm{ mi}$

All of the other choices give the correct perimeter.

$12 \textrm{ yd}$

$288 \textrm{ in }$

Correct answer:

$288 \textrm{ in }$

Explanation:

A rhombus has four sides of equal measure, so the perimeter of a rhombus with sidelength 2 yards is $2 \times 4 = 8$ yards.

$8 \textrm{ yd} = 8 \times 3 = 24 \textrm{ ft} = 24 \times 12 = 288 \textrm{ in}$

Also,

$8 \textrm{ yd} = 24 \textrm{ ft} =\frac{ 24}{5,280} = \frac{1}{220} \textrm{ mi}$

The only choice that agrees with any of these measures is 288 inches, the correct choice.

Report an Error

Example Question #2 : Rhombuses

One side of a rhombus measures y + 12 . Give its perimeter in terms of .

Possible Answers:

$y^{2}+ 12y + 144$

$y^{2}+ 24y + 144$

3y + 36

2y + 24

4y + 48

Correct answer:

4y + 48

Explanation:

A rhombus has four sides of equal measure, so the perimeter of a rhombus with sidelength y + 12 is 4 (y + 12) = 4y + 48 .

Report an Error

Example Question #1 : How To Find An Angle In A Rhombus

Consider the rhombus below. Solve for .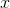

Problem_10

Possible Answers:

x=35

x=325

x=145

x=65

Correct answer:

x=145

Explanation:

The total sum of the interior angles of a quadrilateral is 360 degrees. In this problem, we are only considering half of the interior angles:

$\frac{360}{2}=180$

35+x=180

x=145

Report an Error

Example Question #2 : How To Find An Angle In A Rhombus

Rhombus

Note: Figure NOT drawn to scale.

The above depicts a rhombus and one of its diagonals. What is ?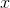

Possible Answers:

x = 58

x = 29

x = 48

x = 42

x = 32

Correct answer:

x = 29

Explanation:

The diagonals of a rhombus bisect the angles.

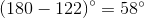
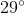
The angle bisected must be supplementary to the $122^{\circ }$ angle since they are consecutive angles of a parallelogram; therefore, that angle has measure $\left (180 - 122 \right ) ^{\circ } = 58^{\circ }$ , and is half that, or $29^{\circ }$ .

Report an Error

Example Question #1 : How To Find The Area Of A Rectangle

A rectangle on the coordinate plane has its vertices at the points (3,4), (-6,4), (-6,-2), (3,-2) .

What percent of the rectangle is located in Quadrant I?

Possible Answers:

$33 \frac{1}{3} \%$

$22 \frac{2}{9} \%$

$11 \frac{1}{9} \%$

$25 \%$

$44 \frac{4}{9} \%$

Correct answer:

$22 \frac{2}{9} \%$

Explanation:

The total area of the rectangle is

$\left [ 3- (-6) \right ] \times\left [ 4- (-2) \right ] = 9 \times 6 = 54$ .

The area of the portion of the rectangle in Quadrant I is

$\left ( 3- 0 \right ) \times\left ( 4- 0 \right) = 3 \times 4 = 12$ .

Therefore, the portion of the rectangle in Quadrant I is

$\frac{12}{54} = \frac{2}{9} = \frac{2}{9} \times 100 \% = 22 \frac{2}{9} \%$ .

Report an Error

Example Question #1 : How To Find The Area Of A Rectangle

A rectangle and a square have the same perimeter. The area of the square is 225 square centimeters; the length of the rectangle is centimeters. Give the width of the rectangle in centimeters.

Possible Answers:

$21 \frac{3}{7}\textrm{ cm}$

$18\textrm{ cm}$

$9\textrm{ cm}$

$10 \frac{5}{7}\textrm{ cm}$

$6\textrm{ cm}$

Correct answer:

$9\textrm{ cm}$

Explanation:

The sidelength of a square with area 225 square centimeters is $\sqrt{225} = 15$ centimeters; its perimeter, as well as that of the rectangle, is therefore $15 \times 4 = 60$ centimeters.

Using the formula for the perimeter of a rectangle, substitute L = 21 and solve for as follows:

2L + 2W = P

$2 \cdot 21 + 2W = 60$

42 + 2W = 60

42 -42 + 2W = 60-42

2W = 18

$2W \div 2 = 18\div 2$

W = 9

Report an Error

Example Question #1 : Rectangles

In a rectangle, the width is while the length is . If 3x=9 , what is the area of the rectangle?

Possible Answers:

Correct answer:

Explanation:

In a rectangle in which the width is x while the length is 4x, the first step is to solve for x. If 3x=9 , the value of x can be found by dividing each side of this equation by 3.

Doing so gives us the information that x is equal to 3.

Thus, the area is equal to:

$Area=x\cdot4x$

$Area=3\cdot4\cdot3$

Area=36

Report an Error

View Tutors

Tom
Certified Tutor

University of Wisconsin-Madison, Bachelor of Science, Atmospheric Sciences and Meteorology. Embry-Riddle Aeronautical Univers...

View Tutors

John
Certified Tutor

The University of Texas at Arlington, Bachelor of Science, Mathematics. Stanford University, Master of Science, Aerospace Eng...

View Tutors

Sundeep
Certified Tutor

Indian Institute of Technology, Engineering in Computer Science, Electrical Engineering. New York University, Masters in Busi...

All ISEE Upper Level Math Resources

6 Diagnostic Tests 244 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Physics Tutors in Houston, LSAT Tutors in Seattle, Algebra Tutors in Los Angeles, ACT Tutors in Miami, Physics Tutors in Dallas Fort Worth, ACT Tutors in Boston, GMAT Tutors in San Diego, MCAT Tutors in Denver, SSAT Tutors in Miami, Computer Science Tutors in Chicago

Popular Courses & Classes

SSAT Courses & Classes in San Diego, LSAT Courses & Classes in Dallas Fort Worth, MCAT Courses & Classes in Dallas Fort Worth, MCAT Courses & Classes in Chicago, LSAT Courses & Classes in Chicago, SSAT Courses & Classes in Los Angeles, MCAT Courses & Classes in Los Angeles, Spanish Courses & Classes in Phoenix, GRE Courses & Classes in Washington DC, SSAT Courses & Classes in Chicago

Popular Test Prep

MCAT Test Prep in Los Angeles, GRE Test Prep in Phoenix, ISEE Test Prep in Denver, GRE Test Prep in Philadelphia, LSAT Test Prep in Dallas Fort Worth, SSAT Test Prep in Houston, MCAT Test Prep in Seattle, ACT Test Prep in New York City, LSAT Test Prep in San Francisco-Bay Area, SSAT Test Prep in Denver

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

ISEE Upper Level Math : Geometry

Study concepts, example questions & explanations for ISEE Upper Level Math

All ISEE Upper Level Math Resources

Example Questions

Example Question #291 : Isee Upper Level (Grades 9 12) Mathematics Achievement

Example Question #1 : How To Find The Length Of The Side Of A Rhombus

Example Question #1 : Rhombuses

Example Question #2 : Rhombuses

Example Question #2 : Rhombuses

Example Question #1 : How To Find An Angle In A Rhombus

Example Question #2 : How To Find An Angle In A Rhombus

Example Question #1 : How To Find The Area Of A Rectangle

Example Question #1 : How To Find The Area Of A Rectangle

Example Question #1 : Rectangles

All ISEE Upper Level Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: