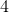We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

ISEE Lower Level Quantitative : Algebraic Concepts

Study concepts, example questions & explanations for ISEE Lower Level Quantitative

Create An Account

All ISEE Lower Level Quantitative Resources

20 Diagnostic Tests 114 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #21 : How To Find The Solution To An Equation

Which of the following expressions can be written as "the quotient of a number and twenty"?

Possible Answers:

x - 20

20 - x

$20 \div x$

$x \div 20$

Correct answer:

$x \div 20$

Explanation:

A quotient is defined as the result of a division; "the quotient of a number and twenty" is written as $x \div 20$ , as opposed to $20 \div x$ , which is "the quotient of twenty and a number".

Report an Error

Example Question #21 : Algebraic Concepts

Max and Sarah both drove at the same speed. It took Max 36 minutes to drive 3 miles. How many minutes did it take for Sarah to drive 6 miles?

Possible Answers:

108

Correct answer:

Explanation:

Since it took Max 36 minutes to drive 3 miles, it must take double the amount of time to drive 6 miles since they're both driving at the same speed. Thus, 72 is the correct answer.

Report an Error

Example Question #23 : How To Find The Solution To An Equation

Martin's family bought him 3 presents for his birthday. The mean cost of a present was $25. If the first present cost $20 and the second present cost $30, how much did the third present cost (in dollars)?

Possible Answers:

Correct answer:

Explanation:

The mean of a set is equal to the sum of the numbers divided by the number of items in the set.

Set up and equation and solve for the missing present:

$mean=25=\frac{20+30+x}{3}$

$25=\frac{50+x}{3}$

Multiply both sides of the equation by 3:

75=50+x

Subtract 50 from both sides:

x=25

Report an Error

Example Question #23 : Equations

4 puppies from a litter are adopted, and $\frac{1}{3}$ are not adopted. How many puppies are in the litter?

Possible Answers:

Correct answer:

Explanation:

If $\frac{1}{3}$ are not adopted, then $\frac{2}{3}$ are adopted. We also know that the number of adopted puppies is 4.

Set up a proportion and solve:

$\frac{4}{x}=\frac{2}{3}$

2x=12

x=6

Therefore, there are 6 puppies total in the litter.

Report an Error

Example Question #24 : Equations

What is the value of in the equation below?

x-4.65=7.23

Possible Answers:

1.88

11.78

11.98

11.88

Correct answer:

11.88

Explanation:

In order to solve for in x-4.65=7.23 , add 4.65 to each side of the equation.

This results in:

x=7.23+4.65

x=11.88

Report an Error

Example Question #22 : Algebraic Concepts

Solve for :

-3+2x=-9

Possible Answers:

x=-6

x=-3

x=-2

x=3

Correct answer:

x=-3

Explanation:

Add 3 to each side:

2x=-6

Divide by 2:

x=-3

Report an Error

Example Question #24 : How To Find The Solution To An Equation

Kiera bought packs of cards. The total she paid was $\$21$ . How much did each pack of cards cost?

Possible Answers:

Correct answer:

Explanation:

Kiera bought packs of cards. The total she paid was $\$21$ .

This problem can be represented with the equation: 7x=21

Remove the by dividing by .

$\frac{7x}{7} = \frac{21}{7} \rightarrow x=3$

The answer is .

Report an Error

Example Question #27 : How To Find The Solution To An Equation

Find the value of :

3x-5=4

Possible Answers:

Correct answer:

Explanation:

To solve an equation, first combine like terms. Move the over to the other side of the equation by adding .

3x-5=4

+5=+5

3x=9

Next, remove the from the variable by dividing by .

$\frac{3x}{3}=\frac{9}{3}\rightarrow x=3$

The answer is .

Report an Error

Example Question #28 : How To Find The Solution To An Equation

Find the value of :

3x+1=28

Possible Answers:

Correct answer:

Explanation:

To solve an equation, first combine like terms. Move the over to the other side of the equation by adding .

3x+1=28

-1=-1

3x=27

Next, remove the from the variable by dividing by .

$\frac{3x}{3}=\frac{27}{3}\rightarrow x=9$

The answer is .

Report an Error

Example Question #24 : Algebraic Concepts

Eight more than four times a number is . What is the number?

Possible Answers:

Correct answer:

Explanation:

Eight more than four times a number is can be expressed with the equation:

4x+8=56

To solve an equation, first combine like terms. Move the to the other side of the equation by adding .

4x+8=56

-8=-8

4x=56

Then, remove the from the variable by dividing by .

$\frac{4x}{4}=\frac{56}{4} \rightarrow x=14$

The answer is .

Report an Error

View Tutors

Gabby
Certified Tutor

University of Oregon, Bachelor in Arts, Journalism. University of Oregon, Current Grad Student, Communication, General.

View Tutors

Crystal
Certified Tutor

SUNY at Binghamton, Bachelor of Science, Mechanical Engineering.

View Tutors

Gregory
Certified Tutor

Pennsylvania State University-Main Campus, Bachelor of Science, Chemical Engineering. Carnegie Mellon University, Doctor of P...

All ISEE Lower Level Quantitative Resources

20 Diagnostic Tests 114 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Math Tutors in Houston, Algebra Tutors in Miami, SSAT Tutors in Miami, Biology Tutors in Denver, Algebra Tutors in Dallas Fort Worth, Math Tutors in Boston, English Tutors in San Diego, English Tutors in Chicago, SAT Tutors in Philadelphia, Physics Tutors in Chicago

Popular Courses & Classes

GRE Courses & Classes in Atlanta, Spanish Courses & Classes in Philadelphia, ISEE Courses & Classes in Los Angeles, GRE Courses & Classes in Miami, MCAT Courses & Classes in Phoenix, ACT Courses & Classes in Houston, ISEE Courses & Classes in Washington DC, ACT Courses & Classes in Dallas Fort Worth, GRE Courses & Classes in San Diego, SAT Courses & Classes in Miami

Popular Test Prep

GMAT Test Prep in Atlanta, ISEE Test Prep in Seattle, SAT Test Prep in Miami, GMAT Test Prep in Boston, MCAT Test Prep in Denver, SSAT Test Prep in Miami, SAT Test Prep in Houston, GRE Test Prep in Phoenix, LSAT Test Prep in Dallas Fort Worth, ISEE Test Prep in Chicago

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

ISEE Lower Level Quantitative : Algebraic Concepts

Study concepts, example questions & explanations for ISEE Lower Level Quantitative

All ISEE Lower Level Quantitative Resources

Example Questions

Example Question #21 : How To Find The Solution To An Equation

Example Question #21 : Algebraic Concepts

Example Question #23 : How To Find The Solution To An Equation

Example Question #23 : Equations

Example Question #24 : Equations

Example Question #22 : Algebraic Concepts

Example Question #24 : How To Find The Solution To An Equation

Example Question #27 : How To Find The Solution To An Equation

Example Question #28 : How To Find The Solution To An Equation

Example Question #24 : Algebraic Concepts

All ISEE Lower Level Quantitative Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: