All High School Math Resources
Example Questions
Example Question #2 : How To Find If Two Acute / Obtuse Triangles Are Similar
Are the triangles similar? If so, solve for 
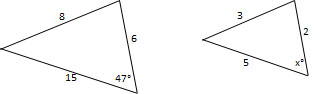
Yes;
Yes;
Yes;
The triangles are not similar
The triangles are not similar
The triangles are not similar, as proven by the side-side-side postulate.
The third side does not follow the same ratio of the other two, thus the triangles are not similar.
Example Question #52 : New Sat Math No Calculator


The perimeter is equal to the sum of the three sides. In similar triangles, each side is in proportion to its correlating side. The perimeters are also in equal proportion.
Perimeter A = 45” and perimeter B = 135”
The proportion of Perimeter A to Perimeter B is 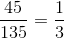
This applies to the sides of the triangle. Therefore to get the any side of Triangle B, just multiply the correlating side by 3.
15” x 3 = 45”
10” x 3 = 30“

Example Question #1 : How To Find If Two Acute / Obtuse Triangles Are Similar
Side-Side-Angle Postulate
Angle-Angle Postulate
Side-Angle-Side Postulate
Side-Side-Side Postulate
Angle-Angle Postulate
Angle-Angle Postulate: if two angles of one triangle are equal to two corresponding angles of another triangle, the triangles must be similar.
In this example, the triangles share one angle, which must be equal. Additionally, the triangles contain segments that are parallel to each other. When two parallel lines are crossed by another line, the corresponding angles must be equal. Each angle in one triangle is congruent with its corresponding angle in the other triangle, indicating that they are similar.
Example Question #3 : How To Find If Two Acute / Obtuse Triangles Are Similar
Side-Angle-Side Postulate
Side-Side-Side Postulate
Angle-Angle Postulate
Side-Side-Angle Postulate
Side-Angle-Side Postulate
The Side-Angle-Side Postulate considers two corresponding sides and the included angle. The included angles must be congruent, and the ratios of the two corresponding sides must be equal. If both criteria are satisfied, then the triangles are similar.
In this problem, there is a shared angle, making it equal for the two triangles. Now, consider the ratios of the sides.
Simplify the fractions.
because the ratios are equal, the triangles are similar by the Side-Angle-Side postulate.
Example Question #183 : Plane Geometry
The ratio of the side lengths of a triangle is 7:10:11. In a similar triangle, the middle side is 9 inches long. What is the length of the longest side of the second triangle?
9.9
10
7.7
12.1
9
9.9
Side lengths of similar triangles can be expressed in proportions. Establish a proportion comparing the middle and long sides of your triangles.
10/11 = 9/x
Cross multiply and solve for x.
10x = 99
x = 9.9
Example Question #184 : Plane Geometry
Two triangles are similar to each other. The bigger one has side lengths of 12, 3, and 14.
The smaller triangle's shortest side is 1 unit in length. What is the length of the smaller triangle's longest side?
Because the triangles are similar, a ratio can be set up between the triangles' longest sides and shortest sides as such: 14/3 = x/1. Solving for x, we obtain that the shortest side of the triangle is 14/3 units long.
Example Question #161 : Sat Mathematics
A triangle with two equal angles is called a(n) __________.
isosceles triangle
Pythagoras triangle
equilateral triangle
right triangle
disjoint triangle
isosceles triangle
An isoceles triangle is a triangle that has at least two congruent sides (and therefore, at least two congruent angles as well).
Example Question #114 : Triangles
Find the height of a triangle if the area of the triangle = 18 and the base = 4.
4
9
6
1
9
The area of a triangle = (1/2)bh where b is base and h is height. 18 = (1/2)4h which gives us 36 = 4h so h =9.
Example Question #85 : Triangles
You have two right triangles that are similar. The base of the first is 6 and the height is 9. If the base of the second triangle is 20, what is the height of the second triangle?
25
23
30
33
35
30
Similar triangles are proportional.
Base1 / Height1 = Base2 / Height2
6 / 9 = 20 / Height2
Cross multiply and solve for Height2
6 / 9 = 20 / Height2
6 * Height2= 20 * 9
Height2= 30
Example Question #1 : Right Triangles
In the figure above, line segments DC and AB are parallel. What is the perimeter of quadrilateral ABCD?
95
80
90
75
85
85
Because DC and AB are parallel, this means that angles CDB and ABD are equal. When two parallel lines are cut by a transversal line, alternate interior angles (such as CDB and ABD) are congruent.
Now, we can show that triangles ABD and BDC are similar. Both ABD and BDC are right triangles. This means that they have one angle that is the same—their right angle. Also, we just established that angles CDB and ABD are congruent. By the angle-angle similarity theorem, if two triangles have two angles that are congruent, they are similar. Thus triangles ABD and BDC are similar triangles.
We can use the similarity between triangles ABD and BDC to find the lengths of BC and CD. The length of BC is proportional to the length of AD, and the length of CD is proportional to the length of DB, because these sides correspond.
We don’t know the length of DB, but we can find it using the Pythagorean Theorem. Let a, b, and c represent the lengths of AD, AB, and BD respectively. According to the Pythagorean Theorem:
a2 + b2 = c2
152 + 202 = c2
625 = c2
c = 25
The length of BD is 25.
We now have what we need to find the perimeter of the quadrilateral.
Perimeter = sum of the lengths of AB, BC, CD, and DA.
Perimeter = 20 + 18.75 + 31.25 + 15 = 85
The answer is 85.
Certified Tutor
Certified Tutor
All High School Math Resources


























