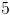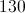We know that all the numbers are positive, so they are greater than zero.  We also know that the numbers are distinct, so they are all unique.

We can write this as {a + b + 8 + d + e}, so let a and b be 1 and 2 respectively, the smallest possible positive, distinct integers. Then let d and e be 9 and 10, the smallest positive, distinct integers larger than 8.

We add the set {1 + 2 + 8 + 9 + 10} to find it equals 30.

In order to multiply to 272, the units digits of the two integers will have to multiply to a number with a units digit of 2. For 17, you can see that 6 x 7 (the units digits of 16 and 17) = 42. The best strategy here is to plug in the choices using the units digit strategy. 

The units digit of any product depends on the units digits of the 2 numbers multiplied, which in this case is 3 and 4. Since , the units digit of  is 2.

The units digit of the product of any two numbers is the same as the units digit of the product of the two numbers' units digits. In this case, it would be the units digit of , which is .

When multiplying integers, if one of the integers is negative, your answer will be negative:

First multiply the ones digits together. 

Next multiply the ones digit of the smaller number with the tens digit of the larger number to get the tens digit of the product.

Combining these two and remembering the negative sign we get our final answer:

When multiplying integers, if both of the signs of the integers are the same (positive, or negative) then your result will be positive:

First multiply the ones digit of both numbers together. If the product is greater than ten remember to carry the one to the tens place.

Next multiply the ones digit of the smaller number with the tens digit of the larger number and add the number that was carried over.

Combine these two together to get:

Simply recognize that logically, the participation of either sport is non-exclusive, that is, just because people took track and field does not necessarily mean they did not take swimming as well. As such, those 51 who took swimming could have all potentially done track and field, which means all 51 students.

There are 120 students in the math club and 150 students in the chess club, for a total membership of 270. However, 100 students are in both clubs, which means they are counted twice. You simply subtract 100 from 270, which will give you a total of 170 different students participating in both clubs. This means that the remaining 130 students do not participate in either club.

When adding integers, one needs to pay close attention to the sign.  When you add a negative integer, it's the same thing as subtracting that integer.  Therefore:

The sum of any two negative numbers will be negative.  Remember, also, that adding a negative number is like subtracting it.  Therefore: