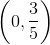We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

Common Core: 8th Grade Math : Expressions & Equations

Study concepts, example questions & explanations for Common Core: 8th Grade Math

Create An Account

All Common Core: 8th Grade Math Resources

7 Diagnostic Tests 75 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #2 : Use Similar Triangles To Show Equal Slopes: Ccss.Math.Content.8.Ee.B.6

The equation of a line is 12x-8y=6 . Find the slope of this line.

Possible Answers:

$-\frac{3}{2}$

$\frac{3}{2}$

$\frac{3}{4}$

$-\frac{3}{4}$

Correct answer:

$\frac{3}{2}$

Explanation:

To find the slope, you will need to put the equation in y=mx+b form. The value of will be the slope.

12x-8y=6

Subtract from either side:

8y=12x-6

Divide each side by :

$y=\frac{3}{2}x-\frac{3}{4}$

You can now easily identify the value of .

$m=\frac{3}{2}$

Report an Error

Example Question #491 : Ssat Upper Level Quantitative (Math)

What is the -intercept of the graph of the function $f \left ( x\right ) = 2x^{2} - 7x + 5$ ?

Possible Answers:

$\left (0,5 \right )$

$\left (0,0 \right )$

$\left ( 0, -3 \frac{1}{2}\right )$

$\left (0,2 \right )$

$\left (0,-7 \right )$

Correct answer:

$\left (0,5 \right )$

Explanation:

The -intercept of the graph of a function is the point at which it intersects the -axis - that is, at which x = 0 . This point is $\left (0, f(0) \right )$ , so evaluate f(0) :

$f \left ( x\right ) = 2x^{2} - 7x + 5$

$f \left (0\right ) = 2 \cdot 0^{2} - 7 \cdot 0 + 5$

$f \left (0\right ) = 0- 0 + 5 = 5$

The -intercept is $\left (0,5 \right )$ .

Report an Error

Example Question #91 : Expressions & Equations

Give the -intercept, if there is one, of the graph of the equation

$y = \frac{1}{x^{2}+ x} + 3$

Possible Answers:

(0,3)

$\left ( 0, \frac{3}{2}\right )$

(0,4)

(0,2)

The graph has no -intercept.

Correct answer:

The graph has no -intercept.

Explanation:

The -intercept is the point at which the graph crosses the -axis; at this point, the -coordinate is 0, so substitute for in the equation:

$y = \frac{1}{x^{2}+ x} + 3$

$y = \frac{1}{0^{2}+0} + 3$

$y = \frac{1}{0} + 3$

However, since this expression has 0 in a denominator, it is of undefined value. This means that there is no value of paired with -coordinate 0, and, subsequently, the graph of the equation has no -intercept.

Report an Error

Example Question #92 : Expressions & Equations

Give the -intercept, if there is one, of the graph of the equation

$y = \frac{3}{\left | 3x-8\right |}$

Possible Answers:

$\left ( 0, \frac{3}{5}\right )$

$\left ( 0,- \frac{3}{5}\right )$

$\left ( 0, \frac{3}{8}\right )$

The graph has no -intercept.

$\left ( 0, -\frac{3}{8}\right )$

Correct answer:

$\left ( 0, \frac{3}{8}\right )$

Explanation:

The -intercept is the point at which the graph crosses the -axis; at this point, the -coordinate is 0, so substitute for in the equation:

$y = \frac{3}{\left | 3x-8\right |}$

$y = \frac{3}{\left | 3 \cdot 0 -8\right |}$

$y = \frac{3}{\left | 0 -8\right |}$

$y = \frac{3}{\left | -8\right |}$

$y = \frac{3}{ 8}$

The -intercept is $\left ( 0, \frac{3}{8}\right )$ .

Report an Error

Example Question #93 : Expressions & Equations

Give the -intercept, if there is one, of the graph of the equation

$y = 3(x-2)^{2} + 4 (x-7)$ .

Possible Answers:

(0, 8)

(0, -64)

(0, -16)

The graph does not have a -intercept.

(0, -42)

Correct answer:

(0, -16)

Explanation:

The -intercept is the point at which the graph crosses the -axis; at this point, the -coordinate is 0, so substitute for in the equation:

$y = 3(x-2)^{2} + 4 (x-7)$

$y = 3(0-2)^{2} + 4 (0-7)$

$y = 3( -2)^{2} + 4 ( -7)$

$y = 3 \cdot 4 + 4 ( -7)$

y = 12+ 4 ( -7)

y = 12+ (-28)

y = -16

The -intercept is the point (0, -16) .

Report an Error

Example Question #2 : X And Y Intercept

A line passes through (5, 2) and is perpendicular to the line of the equation 3x-4y = 17 . Give the -intercept of this line.

Possible Answers:

$\left ( 8\frac{2}{3}, 0\right )$

The line has no -intercept.

$\left (6 \frac{1}{2}, 0 \right )$

$\left (2 \frac{1}{3}, 0 \right )$

$\left ( -1\frac{3}{4}, 0\right )$

Correct answer:

$\left (6 \frac{1}{2}, 0 \right )$

Explanation:

First, find the slope of the second line 3x-4y = 17 by solving for as follows:

3x-4y = 17

3x-4y +4y - 17 = 17+4y - 17

4y = 3x-17

$\frac{4y }{4}=\frac{ 3x-17 }{4}$

$y = \frac{3}{4}x- \frac{17}{4}$

The equation is now in the slope-intercept form y = mx+ b ; the slope of the second line is the -coefficient $m= \frac{3}{4}$ .

The first line, being perpendicular to the second, has as its slope the opposite of the reciprocal of $\frac{3}{4}$ , which is $m=- \frac{4}{3}$ .

Therefore, we are looking for a line through (5, 2) with slope $m=- \frac{4}{3}$ . Using point-slope form

$y - y_{1} = m(x -x_{1})$

with

$x _{1 } =5, y _{1 } = 2,m=- \frac{4}{3}$ ,

the equation becomes

$y - 2 =- \frac{4}{3}(x -5)$ .

To find the -intercept, substitute 0 for and solve for :

$0 - 2 =- \frac{4}{3}(x -5)$

$- 2 =- \frac{4}{3}x+ \frac{20}{3}$

$- 2- \frac{20}{3} =- \frac{4}{3}x+ \frac{20}{3} - \frac{20}{3}$

$- \frac{26}{3} =- \frac{4}{3}x$

$- \frac{3} {4}\cdot\left (- \frac{26}{3} \right )=- \frac{3} {4}\cdot \left (- \frac{4}{3}x \right )$

$x= \frac{26}{4} = \frac{13}{2} = 6\frac{1}{2}$

The -intercept is the point $\left (6 \frac{1}{2}, 0 \right )$ .

Report an Error

Example Question #271 : Geometry

A line passes through (5, 2) and is parallel to the line of the equation 3x-4y = 17 . Give the -intercept of this line.

Possible Answers:

$\left ( -1\frac{3}{4}, 0\right )$

The line has no -intercept.

$\left (2 \frac{1}{3}, 0 \right )$

$\left (6 \frac{1}{2}, 0 \right )$

$\left ( 8\frac{2}{3}, 0\right )$

Correct answer:

$\left (2 \frac{1}{3}, 0 \right )$

Explanation:

First, find the slope of the second line 3x-4y = 17 by solving for as follows:

3x-4y = 17

3x-4y +4y - 17 = 17+4y - 17

4y = 3x-17

$\frac{4y }{4}=\frac{ 3x-17 }{4}$

$y = \frac{3}{4}x- \frac{17}{4}$

The equation is now in the slope-intercept form y = mx+ b ; the slope of the second line is the -coefficient $m= \frac{3}{4}$ .

The first line, being parallel to the second, has the same slope.

Therefore, we are looking for a line through (5, 2) with slope $m= \frac{3}{4}$ . Using point-slope form

$y - y_{1} = m(x -x_{1})$

with

$x _{1 } =5, y _{1 } = 2,m= \frac{3}{4}$ ,

the equation becomes

$y - 2 = \frac{3}{4}(x -5)$ .

To find the -intercept, substitute 0 for and solve for :

$0 - 2 = \frac{3}{4}(x -5)$

$- 2 = \frac{3}{4} x - \frac{15}{4}$

$- 2 + \frac{15}{4}= \frac{3}{4} x - \frac{15}{4} + \frac{15}{4}$

$\frac{3}{4} x = \frac{7}{4}$

$\frac{4} {3}\cdot \frac{3}{4} x =\frac{4} {3}\cdot \frac{7}{4}$

$x = \frac{7}{3} = 2\frac{1}{3}$

The -intercept is the point $\left (2 \frac{1}{3}, 0 \right )$ .

Report an Error

Example Question #1 : How To Find X Or Y Intercept

Give the -intercept of the line with slope $\frac{2}{5}$ that passes through point (5, 9) .

Possible Answers:

$\left (0, -27 \frac{1}{2} \right )$

(0, -11)

The line has no -intercept.

$\left (0, -17 \frac{1}{2} \right )$

(0, 7)

Correct answer:

(0, 7)

Explanation:

By the point-slope formula, this line has the equation

$y - y_{1} = m(x -x_{1})$

where

$x_{1} = 5, y_{1} = 9 ,m = \frac{2}{5}$

By substitution, the equation becomes

$y -9 = \frac{2}{5}(x -5)$

To find the -intercept, substitute 0 for and solve for :

$y -9 = \frac{2}{5}(0 -5)$

$y -9 = \frac{2}{5}( -5)$

y - 9 = -2

y = 7

The -intercept is the point (0, 7) .

Report an Error

Example Question #495 : Ssat Upper Level Quantitative (Math)

Give the -intercept of the line that passes through points (-3, 4) and (2, -3) .

Possible Answers:

$\left (0, - 5\frac{6}{7} \right )$

$\left (0, - \frac{1}{7} \right )$

$\left (0, - 8 \frac{1}{5} \right )$

The line has no -intercept.

$\left (0, - \frac{1}{5} \right )$

Correct answer:

$\left (0, - \frac{1}{5} \right )$

Explanation:

First, find the slope of the line, using the slope formula

$m = \frac{y_{2} -y_{1}}{x_{2}-x_{1}}$

setting $x_{1} = -3, y_{1} = 4, x_{2} = 2, y_{2} = -3$ :

$m = \frac{-3 -4}{2-(-3)} = \frac{-7}{5} =- \frac{7}{5}$

By the point-slope formula, this line has the equation

$y - y_{1} = m(x -x_{1})$

where

$x_{1} = -3, y_{1} = 4, m = - \frac{7}{5}$ ; the line becomes

$y - 4 = - \frac{7}{5}(x -(-3))$

$y - 4 = - \frac{7}{5}(x +3)$

To find the -intercept, substitute 0 for and solve for :

$y - 4 = - \frac{7}{5}(0+3)$

$y - 4 = - \frac{7}{5}(3)$

$y - 4 = - \frac{21}{5}$

$y - 4+ 4 = - \frac{21}{5} + 4$

$y = - \frac{1}{5}$

The -intercept is $\left (0, - \frac{1}{5} \right )$ .

Report an Error

Example Question #8 : How To Find X Or Y Intercept

Give the -intercept of the line that passes through points (-3, 4) and (2, -3) .

Possible Answers:

$\left (- \frac{1}{7} , 0 \right )$

$\left ( - \frac{1}{5},0 \right )$

$\left ( - 8 \frac{1}{5},0 \right )$

$\left (- 5\frac{6}{7} , 0 \right )$

$\text{The line has no } x\text{-intercept.}$

Correct answer:

$\left (- \frac{1}{7} , 0 \right )$

Explanation:

First, find the slope of the line, using the slope formula

$m = \frac{y_{2} -y_{1}}{x_{2}-x_{1}}$

setting $x_{1} = -3, y_{1} = 4, x_{2} = 2, y_{2} = -3$ :

$m = \frac{-3 -4}{2-(-3)} = \frac{-7}{5} =- \frac{7}{5}$

By the point-slope formula, this line has the equation

$y - y_{1} = m(x -x_{1})$

where

$x_{1} = -3, y_{1} = 4, m = - \frac{7}{5}$ ; the line becomes

$y - 4 = - \frac{7}{5}(x -(-3))$

$y - 4 = - \frac{7}{5}(x +3)$

To find the -intercept, substitute 0 for and solve for :

$0 - 4 = - \frac{7}{5}(x +3)$

$- 4 = - \frac{7}{5} x - \frac{21}{5}$

$- 4+ \frac{21}{5} = - \frac{7}{5} x - \frac{21}{5}+ \frac{21}{5}$

$\frac{1}{5} = - \frac{7}{5} x$

$- \frac{5}{7} \cdot \frac{1}{5} = - \frac{5}{7}\left ( - \frac{7}{5} x \right )$

$- \frac{1}{7} =x$

The -intercept is $\left (- \frac{1}{7} , 0 \right )$ .

Report an Error

View Tutors

Amanda
Certified Tutor

Seattle University, Current Undergrad Student, Applied Mathematics.

View Tutors

David
Certified Tutor

Kent State University, Bachelors, Marketing. Kent State University at Kent, Masters in Education, Special Education.

View Tutors

Rainier
Certified Tutor

Norfolk State University, Bachelors, Physics.

All Common Core: 8th Grade Math Resources

7 Diagnostic Tests 75 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Reading Tutors in Miami, LSAT Tutors in San Francisco-Bay Area, GRE Tutors in San Diego, Chemistry Tutors in Miami, Chemistry Tutors in Philadelphia, French Tutors in Atlanta, Statistics Tutors in Atlanta, ACT Tutors in Atlanta, SAT Tutors in San Francisco-Bay Area, MCAT Tutors in Dallas Fort Worth

Popular Courses & Classes

LSAT Courses & Classes in Miami, ACT Courses & Classes in Denver, MCAT Courses & Classes in Chicago, ACT Courses & Classes in Atlanta, Spanish Courses & Classes in Boston, ISEE Courses & Classes in New York City, GRE Courses & Classes in Boston, MCAT Courses & Classes in Boston, LSAT Courses & Classes in Seattle, MCAT Courses & Classes in Los Angeles

Popular Test Prep

SSAT Test Prep in New York City, LSAT Test Prep in Miami, GRE Test Prep in Phoenix, GRE Test Prep in San Diego, MCAT Test Prep in Washington DC, GMAT Test Prep in Washington DC, GRE Test Prep in Denver, SSAT Test Prep in Houston, MCAT Test Prep in San Diego, ISEE Test Prep in Philadelphia

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Common Core: 8th Grade Math : Expressions & Equations

Study concepts, example questions & explanations for Common Core: 8th Grade Math

All Common Core: 8th Grade Math Resources

Example Questions

Example Question #2 : Use Similar Triangles To Show Equal Slopes: Ccss.Math.Content.8.Ee.B.6

Example Question #491 : Ssat Upper Level Quantitative (Math)

Example Question #91 : Expressions & Equations

Example Question #92 : Expressions & Equations

Example Question #93 : Expressions & Equations

Example Question #2 : X And Y Intercept

Example Question #271 : Geometry

Example Question #1 : How To Find X Or Y Intercept

Example Question #495 : Ssat Upper Level Quantitative (Math)

Example Question #8 : How To Find X Or Y Intercept

All Common Core: 8th Grade Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: