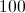All Common Core: 8th Grade Math Resources
Example Questions
Example Question #11 : Expressions & Equations
Evaluate:
A power raised to a power indicates that you multiply the two powers.
Example Question #7 : How To Find The Properties Of An Exponent
Evaluate:
The quantity is undefined.
Example Question #12 : Expressions & Equations
Evaluate:
The expression is undefined.
as 0 taken to any positive power is equal to 0.
Example Question #63 : Algebra
Evaluate:
The expression is undefined.
Example Question #12 : Generate Equivalent Numerical Expressions: Ccss.Math.Content.8.Ee.A.1
Evaluate:
The bases of all three terms are alike. Since the terms are of a specific power, the rule of exponents state that the powers can be added if the terms are multiplied.
When we have a negative exponent, we we put the number and the exponent as the denominator, over
Example Question #13 : Expressions & Equations
Simplify:
To solve this problem, we start with the parentheses and exponents in the denominator.
Next, we can bring the 
Finally, to get rid of the negative exponent we can bring it back down to the denominator.
Example Question #11 : Generate Equivalent Numerical Expressions: Ccss.Math.Content.8.Ee.A.1
Solve:
In order to solve this problem, we need to recall our exponent rules:
When our base numbers are equal to each other, like in this problem, we can add our exponents together using the following formula:
Let's apply this rule to our problem
Solve for the exponents
We cannot leave this problem in this format because we cannot have a negative exponent. Instead, we can move the base and the exponent to the denominator of a fraction:
Solve the problem
Example Question #16 : Expressions & Equations
Solve:
In order to solve this problem, we need to recall our exponent rules:
When our base numbers are equal to each other, like in this problem, we can add our exponents together using the following formula:
Let's apply this rule to our problem
Solve for the exponents
We cannot leave this problem in this format because we cannot have a negative exponent. Instead, we can move the base and the exponent to the denominator of a fraction:
Solve the problem
Example Question #17 : Expressions & Equations
Solve:
In order to solve this problem, we need to recall our exponent rules:
When our base numbers are equal to each other, like in this problem, we can add our exponents together using the following formula:
Let's apply this rule to our problem
Solve for the exponents
We cannot leave this problem in this format because we cannot have a negative exponent. Instead, we can move the base and the exponent to the denominator of a fraction:
Solve the problem
Example Question #18 : Expressions & Equations
Solve:
In order to solve this problem, we need to recall our exponent rules:
When our base numbers are equal to each other, like in this problem, we can add our exponents together using the following formula:
Let's apply this rule to our problem
Solve for the exponents
We cannot leave this problem in this format because we cannot have a negative exponent. Instead, we can move the base and the exponent to the denominator of a fraction:
Solve the problem
Certified Tutor
All Common Core: 8th Grade Math Resources