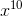Call Now to Set Up Tutoring:

(888) 888-0446

Common Core: 8th Grade Math : Expressions & Equations

Study concepts, example questions & explanations for Common Core: 8th Grade Math

Create An Account

All Common Core: 8th Grade Math Resources

7 Diagnostic Tests 75 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Simplifying Exponents

Simplify the expression:

$(3x^4y^2)(4xy^2)^{-3}$

Possible Answers:

$\frac{3x}{64y^3}$

$\frac{3x}{64y^4}$

Cannot be simplified

$\frac{3x}{16y^3}$

Correct answer:

$\frac{3x}{64y^4}$

Explanation:

Begin by distributing the exponent through the parentheses. The power rule dictates that an exponent raised to another exponent means that the two exponents are multiplied:

$(3x^{4}y^2)(4xy^2)^{-3}= (3xy^2)(4^{-3}x^{-3}y^{-6})$

Any negative exponents can be converted to positive exponents in the denominator of a fraction:

$\frac{3x^4y^2}{64x^3y^6}$

The like terms can be simplified by subtracting the power of the denominator from the power of the numerator:

$\frac{3x^4y^2}{64x^3y^6}=\frac{3}{64}x^{4-3}y^{2-6}=\frac{3}{64}xy^{-4}$

$\frac{3x}{64y^4}$

Report an Error

Example Question #1 : Integer Exponents

Simplify:

(4x^3)^4

Possible Answers:

512x

16x^7

4x^7

$256x^{12}$

$4x^{12}$

Correct answer:

$256x^{12}$

Explanation:

Use the power rule to distribute the exponent:

$(4x^3)^4\rightarrow 4^4\cdot x^{3\cdot 4}\rightarrow 256x^{12}$

Report an Error

Example Question #11 : Distributing Exponents (Power Rule)

Simplify: (2^3)^2-(2^2+ 2^3)^2

Possible Answers:

Correct answer:

Explanation:

To simplify this, we will need to use the power rule and order of operations.

Evaluate the first term. This will be done in two ways to show that the power rule will work for exponents outside of the parenthesis for a single term.

$(2^3)^2 = 2^3\times 2^3 =8\times 8 = 64$

$(2^3)^2 = 2^{3\times 2} = 2^6 =64$

For the second term, we cannot distribute 2^2 and 2^3 with the exponent outside the parentheses because it's not a single term. Instead, we must evaluate the terms inside the parentheses first.

Evaluate the second term.

(2^2+ 2^3)^2 = (4+8)^2 = (12)^2

Square the value inside the parentheses.

$(12)^2 = 12\times 12 = 144$

Subtract the value of the second term with the first term.

64-144 = -80

Report an Error

Example Question #1 : Expressions & Equations

Which of the following is equivalent to the expression (7x^5)^3 ?

Possible Answers:

$343x^{8}$

$49x^{2}$

$7x^{15}$

$343x^{15}$

Correct answer:

$343x^{15}$

Explanation:

Which of the following is equivalent to the expression (7x^5)^3 ?

We can rewrite the given expression by distributing the exponent on the outside.

(7x^5)^3=7^3(x^5)^3

Now, this may look a little messier, but we need to recall that when we distribute an exponent through parentheses as we are trying to do above, we need to multiple the exponent on the inside by the number on the outside.

In a general sense it looks like this:

$(a^b)^c=a^{b*c}$

For our specific problem, it looks like this:

$7^3(x^5)^3=7^3x^{15}=343x^{15}$

Report an Error

Example Question #2 : Generate Equivalent Numerical Expressions: Ccss.Math.Content.8.Ee.A.1

Simplify.

$(x^4)^{6}$

Possible Answers:

x^2

$x^{10}$

$x^{-2}$

$x^{46}$

$x^{24}$

Correct answer:

$x^{24}$

Explanation:

When an exponent is being raised by another exponent, we just multiply the powers of the exponents and keep the base the same.

$(x^4)^{6}=x^{4*6}=x^{24}$

Report an Error

Example Question #14 : Distributing Exponents (Power Rule)

Simplify.

$(x^{-3})^{-4}$

Possible Answers:

$x^{12}$

$x^{-7}$

$x^{7}$

$x^{34}$

$x^{-12}$

Correct answer:

$x^{12}$

Explanation:

When an exponent is being raised by another exponent, we just multiply the powers of the exponents and keep the base the same.

$(x^{-3})^{-4}=x^{-3*-4}=x^{12}$

Report an Error

Example Question #21 : Distributing Exponents (Power Rule)

Simplify.

$(\frac{x^5}{y^3})^3$

Possible Answers:

$\frac{x^{8}}{y^6}$

$\frac{x^{15}}{y^{13}}$

$\frac{x^{15}}{y^8}$

$\frac{x^{15}}{y^3}$

$\frac{x^{15}}{y^9}$

Correct answer:

$\frac{x^{15}}{y^9}$

Explanation:

When an exponent is being raised by another exponent, we just multiply the powers of the exponents and keep the base the same.

$(\frac{x^5}{y^3})^{3}=\frac{x^{5*3}}{y^{3*3}}=\frac{x^{15}}{y^{9}}$

Report an Error

Example Question #21 : Distributing Exponents (Power Rule)

Simplify the expression $\left ( 2x^3\right )^5$

Possible Answers:

$2x^{15}$

$32768x^{15}$

32x^8

$32x^{15}$

2x^8

Correct answer:

$32x^{15}$

Explanation:

$\left ( 2x^5\right )^3=\left ( 2x^5\right )\left ( 2x^5\right )\left ( 2x^5\right )$

$8x^{15}$

Report an Error

Example Question #1 : Generate Equivalent Numerical Expressions: Ccss.Math.Content.8.Ee.A.1

Evaluate $\dpi{100} \frac{2^{10}}{2^{8}}$

Possible Answers:

$\dpi{100} 2$

$\dpi{100} \frac{8}{5}$

$\dpi{100} 200$

$\dpi{100} 4$

Correct answer:

$\dpi{100} 4$

Explanation:

If you divide two exponential expressions with the same base, you can simply subtract the exponents. Here, both the top and the bottom have a base of 2 raised to a power.

So $\dpi{100} \frac{2^{10}}{2^{8}}=2^{10-8}=2^{2}=4$

Report an Error

Example Question #1 : Properties Of Exponents

$\dpi{100} 2^{3}\cdot 2^{2}$

Possible Answers:

$\dpi{100} 16$

$\dpi{100} 2^{6}$

$\dpi{100} 32$

Correct answer:

$\dpi{100} 32$

Explanation:

Since the two expressions have the same base, we just add the exponents.

$\dpi{100} 2^{3}\cdot 2^{2}=2^{3+2}=2^{5}=32$

Report an Error

View Tutors

Amanda
Certified Tutor

Seattle University, Current Undergrad Student, Applied Mathematics.

View Tutors

Ingy
Certified Tutor

University of Minnesota-Twin Cities, Bachelors, Mathematics with Actuarial Science Specialization.

View Tutors

Sarah
Certified Tutor

New York University, Bachelor of Fine Arts, Drama.

All Common Core: 8th Grade Math Resources

7 Diagnostic Tests 75 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

SSAT Tutors in San Diego, Reading Tutors in Denver, Spanish Tutors in Houston, SSAT Tutors in New York City, Spanish Tutors in Denver, SSAT Tutors in Dallas Fort Worth, English Tutors in Boston, Reading Tutors in Atlanta, LSAT Tutors in Dallas Fort Worth, Reading Tutors in Boston

Popular Courses & Classes

GMAT Courses & Classes in Phoenix, GRE Courses & Classes in Atlanta, GMAT Courses & Classes in Seattle, MCAT Courses & Classes in Washington DC, LSAT Courses & Classes in Dallas Fort Worth, MCAT Courses & Classes in New York City, ACT Courses & Classes in New York City, ISEE Courses & Classes in Seattle, SAT Courses & Classes in Houston, GMAT Courses & Classes in Denver

Popular Test Prep

ISEE Test Prep in Los Angeles, GRE Test Prep in San Francisco-Bay Area, ACT Test Prep in New York City, GRE Test Prep in Chicago, GRE Test Prep in Miami, MCAT Test Prep in Chicago, GMAT Test Prep in Dallas Fort Worth, GMAT Test Prep in San Francisco-Bay Area, LSAT Test Prep in Los Angeles, GMAT Test Prep in Miami

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Common Core: 8th Grade Math : Expressions & Equations

Study concepts, example questions & explanations for Common Core: 8th Grade Math

All Common Core: 8th Grade Math Resources

Example Questions

Example Question #1 : Simplifying Exponents

Example Question #1 : Integer Exponents

Example Question #11 : Distributing Exponents (Power Rule)

Example Question #1 : Expressions & Equations

Example Question #2 : Generate Equivalent Numerical Expressions: Ccss.Math.Content.8.Ee.A.1

Example Question #14 : Distributing Exponents (Power Rule)

Example Question #21 : Distributing Exponents (Power Rule)

Example Question #21 : Distributing Exponents (Power Rule)

Example Question #1 : Generate Equivalent Numerical Expressions: Ccss.Math.Content.8.Ee.A.1

Example Question #1 : Properties Of Exponents

All Common Core: 8th Grade Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: