Call Now to Set Up Tutoring:

(888) 888-0446

Basic Geometry : Triangles

Study concepts, example questions & explanations for Basic Geometry

Create An Account

All Basic Geometry Resources

9 Diagnostic Tests 164 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #5 : How To Find The Height Of A Right Triangle

If the area of a right triangle is , and the base of the triangle is , what is the height of the triangle?

Possible Answers:

Correct answer:

Explanation:

Recall how to find the area of a right triangle:

$\text{Area}=\frac{1}{2}(\text{base}\times\text{height})$

Now, we are going to manipulate the equation to solve for height.

$2(\text{Area})=\text{base}\times\text{height}$

$\text{Height}=\frac{2(\text{Area})}{\text{base}}$

Now, plug in the information given by the question about the values of the area and base of the triangle to find the height.

$\text{Height}=\frac{2(18)}{2}=\frac{36}{2}=18$

Report an Error

Example Question #1491 : Basic Geometry

If the area of a right triangle is , and the base of the triangle is , what is the height of the triangle?

Possible Answers:

Correct answer:

Explanation:

Recall how to find the area of a right triangle:

$\text{Area}=\frac{1}{2}(\text{base}\times\text{height})$

Now, we are going to manipulate the equation to solve for height.

$2(\text{Area})=\text{base}\times\text{height}$

$\text{Height}=\frac{2(\text{Area})}{\text{base}}$

Now, plug in the information given by the question about the values of the area and base of the triangle to find the height.

$\text{Height}=\frac{2(30)}{4}=\frac{60}{4}=15$

Report an Error

Example Question #1492 : Basic Geometry

If the area of a right triangle is , and the base of the triangle is , what is the height of the triangle?

Possible Answers:

Correct answer:

Explanation:

Recall how to find the area of a right triangle:

$\text{Area}=\frac{1}{2}(\text{base}\times\text{height})$

Now, we are going to manipulate the equation to solve for height.

$2(\text{Area})=\text{base}\times\text{height}$

$\text{Height}=\frac{2(\text{Area})}{\text{base}}$

Now, plug in the information given by the question about the values of the area and base of the triangle to find the height.

$\text{Height}=\frac{2(60)}{40}=\frac{120}{40}=3$

Report an Error

Example Question #1493 : Basic Geometry

If the area of a right triangle is , and the base of the triangle is , what is the height of the triangle?

Possible Answers:

Correct answer:

Explanation:

Recall how to find the area of a right triangle:

$\text{Area}=\frac{1}{2}(\text{base}\times\text{height})$

Now, we are going to manipulate the equation to solve for height.

$2(\text{Area})=\text{base}\times\text{height}$

$\text{Height}=\frac{2(\text{Area})}{\text{base}}$

Now, plug in the information given by the question about the values of the area and base of the triangle to find the height.

$\text{Height}=\frac{2(22)}{11}=\frac{44}{11}=4$

Report an Error

Example Question #1494 : Basic Geometry

If the area of a right triangle is , and the base of the triangle is , what is the height of the triangle?

Possible Answers:

$\frac{1}{8}$

$\frac{1}{2}$

$\frac{1}{4}$

Correct answer:

Explanation:

Recall how to find the area of a right triangle:

$\text{Area}=\frac{1}{2}(\text{base}\times\text{height})$

Now, we are going to manipulate the equation to solve for height.

$2(\text{Area})=\text{base}\times\text{height}$

$\text{Height}=\frac{2(\text{Area})}{\text{base}}$

Now, plug in the information given by the question about the values of the area and base of the triangle to find the height.

$\text{Height}=\frac{2(4)}{8}=\frac{8}{8}=1$

Report an Error

Example Question #1495 : Basic Geometry

If the area of a right triangle is , and the base of the triangle is , what is the height of the triangle?

Possible Answers:

Correct answer:

Explanation:

Recall how to find the area of a right triangle:

$\text{Area}=\frac{1}{2}(\text{base}\times\text{height})$

Now, we are going to manipulate the equation to solve for height.

$2(\text{Area})=\text{base}\times\text{height}$

$\text{Height}=\frac{2(\text{Area})}{\text{base}}$

Now, plug in the information given by the question about the values of the area and base of the triangle to find the height.

$\text{Height}=\frac{2(3)}{3}=\frac{6}{3}=2$

Report an Error

Example Question #1496 : Basic Geometry

Prblem 8 basic geometry

Find the missing length in triangle ABC.

Possible Answers:

$2\ in$

$5\ in$

$5/4\ in$

$3\ in$

$4\ in$

Correct answer:

$4\ in$

Explanation:

To find the missing length, use the Pythagorean Theorem.

a^2+b^2=c^2

Where "a" and "b" are the legs, and "c" is the hypotenuse.

a=3 inches

b=???

c= 5 inches

Plug in the values

3^2+b^2=5^2

9+b^2=25

Now, get the variable by itself.

b^2=16

Take the square root of both sides.

$\sqrt{b^2}=\sqrt{16}$

b=4

Report an Error

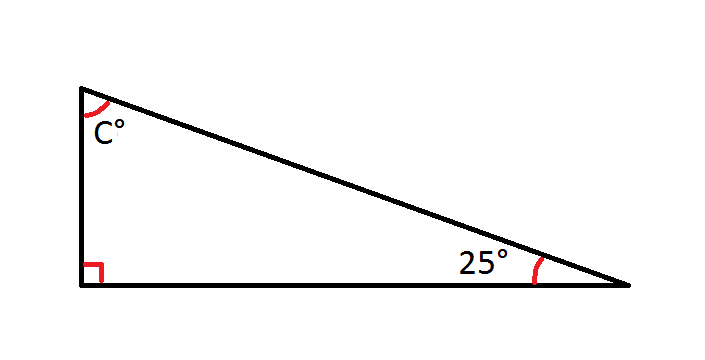
Example Question #1 : How To Find An Angle In A Right Triangle

Find angle C.

Triangle_90_25_c

Possible Answers:

C=53

C=72

C=65

C=70

None of these

Correct answer:

C=65

Explanation:

First, know that all the angles in a triangle add up to 180 degrees.

Each triangle has 3 angles. Thus, we have the sum of three angles as shown:

180=A+B+C

where we have angles A, B, and C. In our right triangle, one angle is 25 degree and we'll call that angle A. The other known angle is 90 degrees and we'll call this angle B. Thus, we have

180=25+90+C

Simplify and solve for C.

180=115+C

C=65

Report an Error

Example Question #1 : How To Find An Angle In A Right Triangle

Which of the following can be two angle measures of a right triangle?

Possible Answers:

$35^{\circ},55^{\circ}$

$100^{\circ},10^{\circ}$

$38^{\circ},42^{\circ}$

$60^{\circ},40^{\circ}$

$60^{\circ},60^{\circ}$

Correct answer:

$35^{\circ},55^{\circ}$

Explanation:

A right triangle cannot have an obtuse angle; this eliminates the choice of 100 and 10.

The acute angles of a right triangle must total 90 degrees. Three choices can be eliminated by this criterion:

60+40=100

38+42=80

60+60=120

The remaining choice is correct: 35+55=90

Report an Error

Example Question #2 : How To Find An Angle In A Right Triangle

A right triangle has an angle that is 15 more than twice the other. What is the smaller angle?

Possible Answers:

Correct answer:

Explanation:

The sum of the angles in a triangle is 180. A right triangle has one angle of 90. Thus, the sum of the other two angles will be 90.

Let = first angle and 2x + 15 = second angle

So the equation to solve becomes x+ 2x+15 = 90 or 3x=75

Thus, the first angle is and the second angle is .

So the smaller angle is

Report an Error

View Tutors

Karin
Certified Tutor

SUNY College at Cortland, Bachelor of Science, Mathematics Teacher Education. New York University, Master of Arts, Educationa...

View Tutors

Usman
Certified Tutor

New York University, Bachelor in Arts, Political Science and Government.

View Tutors

Filiberto
Certified Tutor

University of Arizona, Bachelor of Science, Electrical Engineering.

All Basic Geometry Resources

9 Diagnostic Tests 164 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Physics Tutors in Boston, Statistics Tutors in Miami, Chemistry Tutors in Miami, Statistics Tutors in Boston, ACT Tutors in Atlanta, Calculus Tutors in Los Angeles, Algebra Tutors in Houston, Algebra Tutors in Denver, SSAT Tutors in New York City, Algebra Tutors in Atlanta

Popular Courses & Classes

MCAT Courses & Classes in Phoenix, GRE Courses & Classes in New York City, GRE Courses & Classes in San Diego, GMAT Courses & Classes in New York City, MCAT Courses & Classes in San Francisco-Bay Area, LSAT Courses & Classes in Boston, ISEE Courses & Classes in San Francisco-Bay Area, MCAT Courses & Classes in Miami, LSAT Courses & Classes in Phoenix, LSAT Courses & Classes in Philadelphia

Popular Test Prep

ISEE Test Prep in Dallas Fort Worth, MCAT Test Prep in Dallas Fort Worth, MCAT Test Prep in Los Angeles, ACT Test Prep in Seattle, SAT Test Prep in Boston, LSAT Test Prep in Los Angeles, GMAT Test Prep in Los Angeles, SSAT Test Prep in Philadelphia, SSAT Test Prep in San Francisco-Bay Area, GRE Test Prep in Chicago

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Basic Geometry : Triangles

Study concepts, example questions & explanations for Basic Geometry

All Basic Geometry Resources

Example Questions

Example Question #5 : How To Find The Height Of A Right Triangle

Example Question #1491 : Basic Geometry

Example Question #1492 : Basic Geometry

Example Question #1493 : Basic Geometry

Example Question #1494 : Basic Geometry

Example Question #1495 : Basic Geometry

Example Question #1496 : Basic Geometry

Example Question #1 : How To Find An Angle In A Right Triangle

Example Question #1 : How To Find An Angle In A Right Triangle

Example Question #2 : How To Find An Angle In A Right Triangle

All Basic Geometry Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: