Call Now to Set Up Tutoring:

(888) 888-0446

Basic Geometry : Triangles

Study concepts, example questions & explanations for Basic Geometry

Create An Account

All Basic Geometry Resources

9 Diagnostic Tests 164 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #81 : How To Find The Area Of A Right Triangle

The diameter of the circle is , find the area of the shaded region.

Possible Answers:

13.38

9.98

12.41

10.99

Correct answer:

13.38

Explanation:

To find the area of the shaded region, we will first need to find the area of the right triangle and the area of the circle.

Recall how to find the area of a circle:

$\text{Area of Circle}=\pi\times\text{radius}^2$

Now, recall how to find the length of the radius from the length of the diameter.

$\text{Radius}=\frac{\text{Diameter}}{2}$

Substitute in the given diameter to find the radius.

$\text{Radius}=\frac{9}{2}$

Now, substitute in the radius to find the area of the circle.

$\text{Area of Circle}=\pi\times\left( \frac{9}{2}\right)^2$

$\text{Area of Circle}=\frac{81}{4}\pi$

Next, recall how to find the area of a right triangle.

$\text{Area of Triangle}=\frac{\text{base}\times\text{height}}{2}$

Substitute in the given base and height to find the area.

$\text{Area of Triangle}=\frac{11 \times 14}{2}$

$\text{Area of Triangle}=77$

We can now find the area of the shaded region:

$\text{Area of Shaded Region}=\text{Area of Triangle}-\text{Area of Circle}$

$\text{Area of Shaded Region}=77-\frac{81}{4}\pi$

Solve and round to two decimal places.

$\text{Area of Shaded Region}=13.38$

Report an Error

Example Question #82 : How To Find The Area Of A Right Triangle

The diameter of the circle is 2.2 , find the area of the shaded region.

Possible Answers:

17.78

15.98

16.20

14.28

Correct answer:

16.20

Explanation:

To find the area of the shaded region, we will first need to find the area of the right triangle and the area of the circle.

Recall how to find the area of a circle:

$\text{Area of Circle}=\pi\times\text{radius}^2$

Now, recall how to find the length of the radius from the length of the diameter.

$\text{Radius}=\frac{\text{Diameter}}{2}$

Substitute in the given diameter to find the radius.

$\text{Radius}=\frac{2.2}{2}$

$\text{Radius}=1.1$

Now, substitute in the radius to find the area of the circle.

$\text{Area of Circle}=\pi\times 1.1^2$

$\text{Area of Circle}=1.21\pi$

Next, recall how to find the area of a right triangle.

$\text{Area of Triangle}=\frac{\text{base}\times\text{height}}{2}$

Substitute in the given base and height to find the area.

$\text{Area of Triangle}=\frac{4 \times 10}{2}$

$\text{Area of Triangle}=20$

We can now find the area of the shaded region:

$\text{Area of Shaded Region}=\text{Area of Triangle}-\text{Area of Circle}$

$\text{Area of Shaded Region}=20-1.21\pi$

Solve and round to two decimal places.

$\text{Area of Shaded Region}=16.20$

Report an Error

Example Question #84 : How To Find The Area Of A Right Triangle

The diameter of the circle is , find the area of the shaded region.

Possible Answers:

89.89

93.44

90.90

91.28

Correct answer:

90.90

Explanation:

To find the area of the shaded region, we will first need to find the area of the right triangle and the area of the circle.

Recall how to find the area of a circle:

$\text{Area of Circle}=\pi\times\text{radius}^2$

Now, recall how to find the length of the radius from the length of the diameter.

$\text{Radius}=\frac{\text{Diameter}}{2}$

Substitute in the given diameter to find the radius.

$\text{Radius}=\frac{12}{2}$

$\text{Radius}=6$

Now, substitute in the radius to find the area of the circle.

$\text{Area of Circle}=\pi\times 6^2$

$\text{Area of Circle}=36\pi$

Next, recall how to find the area of a right triangle.

$\text{Area of Triangle}=\frac{\text{base}\times\text{height}}{2}$

Substitute in the given base and height to find the area.

$\text{Area of Triangle}=\frac{17 \times 24}{2}$

$\text{Area of Triangle}=204$

We can now find the area of the shaded region:

$\text{Area of Shaded Region}=\text{Area of Triangle}-\text{Area of Circle}$

$\text{Area of Shaded Region}=204-36\pi$

Solve and round to two decimal places.

$\text{Area of Shaded Region}=90.90$

Report an Error

Example Question #481 : Triangles

A triangle has a height of 5 inches and a base of 10 inches. What is the area of this triangle?

Possible Answers:

None of these.

A=25in^2

A=15in^2

A=65in^2

A=30in^2

Correct answer:

A=25in^2

Explanation:

The area of a right triangle can be found by A=.5bh .

$A=.5bh=.5(10)(5)=\mathbf{25in^2}$

Report an Error

Example Question #86 : How To Find The Area Of A Right Triangle

Prblem 6 basic geometry

Triangle ABC has the given side lengths. Find the area of triangle ABC.

Possible Answers:

$100\ inches^2$

$182\ inches^2$

$121\ inches^2$

$91\ inches^2$

$89\ inches^2$

Correct answer:

$91\ inches^2$

Explanation:

Imagine a right triangle as a square cut in half at a diagonal angle.

When figuring out the area, you figure it out the same way as finding the area of a square, but after multiplying length x width, divide the answer by 2.

$14 \cdot 13 = 182inches^2$

182/2=91 inches^2

Report an Error

Example Question #87 : How To Find The Area Of A Right Triangle

Find the area, , of a right triangle XYZ whose sides are X=12 , Y=5 , Z=13 .

Possible Answers:

A=35

A=50

A=30

A=40

Correct answer:

A=30

Explanation:

The formula for the area of a right triangle is

$A=\frac{1}{2}XY$ .

Plugging in the values given,

$A=\frac{1}{2}*5*12=30$ .

Report an Error

Example Question #84 : How To Find The Area Of A Right Triangle

Shape area right triangle

In the right triangle shown here, a=5 and b=12 . What is its area in square units?

Possible Answers:

Correct answer:

Explanation:

The area of a right triangle is given by $A=\frac{bh}{2}$ , where represents the length of the triangle's base and represents the length of the triangle's height. The base and the height of the triangle given in the problem are and units long, respectively. Hence, the area of the triangle can be calculated as follows:

$A=\frac{bh}{2}= \frac{(12)(5)}{2} = 30$ .

Hence, the area of a right triangle with base length units and height units is square units.

Report an Error

Example Question #1 : How To Find If Right Triangles Are Congruent

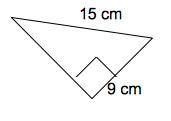
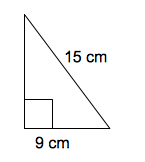
Are the two right triangles congruent?

Possible Answers:

Yes, by AAA

Yes, by HL

Yes, by AAS

Yes, by AAA

No, they are not congruent

Correct answer:

Yes, by HL

Explanation:

Right triangles are congruent if both the hypotenuse and one leg are the same length. These triangles are congruent by HL, or hypotenuse-leg.

Report an Error

Example Question #1 : How To Find If Right Triangles Are Congruent

Which of the following is not sufficient to show that two right triangles are congruent?

Possible Answers:

All the sides are congruent.

All the angles are congruent.

Both legs are congruent.

The hypotenuse and one leg are congruent.

Correct answer:

All the angles are congruent.

Explanation:

Two right triangles can have all the same angles and not be congruent, merely scaled larger or smaller. If all the side lengths are multiplied by the same number, the angles will remain unchanged, but the triangles will not be congruent.

Report an Error

Example Question #1 : How To Find If Right Triangles Are Congruent

Which of the following pieces of information would not allow the conclusion that

$\bigtriangleup ABD\cong \bigtriangleup CBD$

Possible Answers:

$m\angle ABC =100^o$

$\angle BAC\cong \angle BCA$

AB=10

AC=16

$\overrightarrow{BD}$ bisects $\angle ABC$

Correct answer:

$m\angle ABC =100^o$

Explanation:

To determine the answer choice that does not lead to congruence, we should simply use process of elimination.

If AC=16 , then subtracting tells us that DC=8 .; therefore $\overline{AD}\cong \overline{DC}$ . Given the fact that reflexively $\overline{BD}\cong\overline{BD}$ and that both $\angle BDA$ and $\angle BDC$ are both right angles and thus congruent, we can establish congruence by way of Side-Angle-Side.

Similarly, if AB=10 , then $\overline{AB}\cong \overline{BC}$ , and given the other information we determined with our last choice, we can establish conguence by way of Hypotenuse-Leg.

If $\angle BAC\cong \angle BCA$ , given what we already know we can establish congruence by Angle-Angle-Side

Finally, if $\overrightarrow{BD}$ is an angle bisector, then our two halves are congruent. $\angle ABD\cong \angle CBD$ . Given what we know, we can establish congruence by Angle-Side-Angle

The only remaining choice is the case where $m\angle ABC=100^o$ . This does not tell us how the two parts of this angle are related, we lack enough information for congruence.

Report an Error

View Tutors

Eleanor
Certified Tutor

Temple University, Bachelor in Arts, History. Drexel University, Master of Science, High School Teaching.

View Tutors

Momeena
Certified Tutor

State University of New York at New Paltz, Bachelors, Mathematics & Elementary Education. State University of New York at New...

View Tutors

Ranjeeta
Certified Tutor

University of Rajasthan, Bachelor of Science, Mathematics. University of Rajasthan, Master of Science, Mathematics.

All Basic Geometry Resources

9 Diagnostic Tests 164 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

LSAT Tutors in Washington DC, Physics Tutors in New York City, GMAT Tutors in Phoenix, Reading Tutors in Denver, Chemistry Tutors in San Francisco-Bay Area, Calculus Tutors in San Francisco-Bay Area, Statistics Tutors in New York City, GRE Tutors in Dallas Fort Worth, SAT Tutors in Seattle, English Tutors in Phoenix

Popular Courses & Classes

Spanish Courses & Classes in Atlanta, ACT Courses & Classes in Denver, GMAT Courses & Classes in San Diego, Spanish Courses & Classes in Philadelphia, GRE Courses & Classes in Boston, GMAT Courses & Classes in Philadelphia, ISEE Courses & Classes in Phoenix, ISEE Courses & Classes in Chicago, GRE Courses & Classes in Phoenix, GRE Courses & Classes in Atlanta

Popular Test Prep

MCAT Test Prep in Boston, LSAT Test Prep in Los Angeles, GRE Test Prep in Phoenix, SSAT Test Prep in Boston, ACT Test Prep in Atlanta, MCAT Test Prep in Houston, GMAT Test Prep in Denver, GRE Test Prep in New York City, GRE Test Prep in Atlanta, GMAT Test Prep in Philadelphia

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Basic Geometry : Triangles

Study concepts, example questions & explanations for Basic Geometry

All Basic Geometry Resources

Example Questions

Example Question #81 : How To Find The Area Of A Right Triangle

Example Question #82 : How To Find The Area Of A Right Triangle

Example Question #84 : How To Find The Area Of A Right Triangle

Example Question #481 : Triangles

Example Question #86 : How To Find The Area Of A Right Triangle

Example Question #87 : How To Find The Area Of A Right Triangle

Example Question #84 : How To Find The Area Of A Right Triangle

Example Question #1 : How To Find If Right Triangles Are Congruent

Example Question #1 : How To Find If Right Triangles Are Congruent

Example Question #1 : How To Find If Right Triangles Are Congruent

All Basic Geometry Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: