How do you find the perimeter of a right triangle?

There are three primary methods used to find the perimeter of a right triangle.

1. When side lengths are given, add them together.
2. Solve for a missing side using the Pythagorean theorem.
3. If we know side-angle-side information, solve for the missing side using the Law of Cosines.

Method 1:

This method will show you how to calculate the perimeter of a triangle when all sides lengths are known. Consider the following figure:

If we know the lengths of sides , , and , then we can simply add them together to find the perimeter of the triangle. It is important to note several things. First, we need to make sure that all the units given match one another. Second, when all the side lengths are known, then the perimeter formula may be used on all types of triangles (e.g. right, acute, obtuse, equilateral, isosceles, and scalene). The perimeter formula is written formally in the following format:

Method 2:

In right triangles, we can calculate the perimeter of a triangle when we are provided only two sides. We can do this by using the Pythagorean theorem. Let's first discuss right triangles in a general sense. A right triangle is a triangle that has one  angle. It is a special triangle and needs to be labeled accordingly. The legs of the triangle form the  angle and they are labeled  and . The side of the triangle that is opposite of the  angle and connects the two legs is known as the hypotenuse. The hypotenuse is the longest side of the triangle and is labeled as .

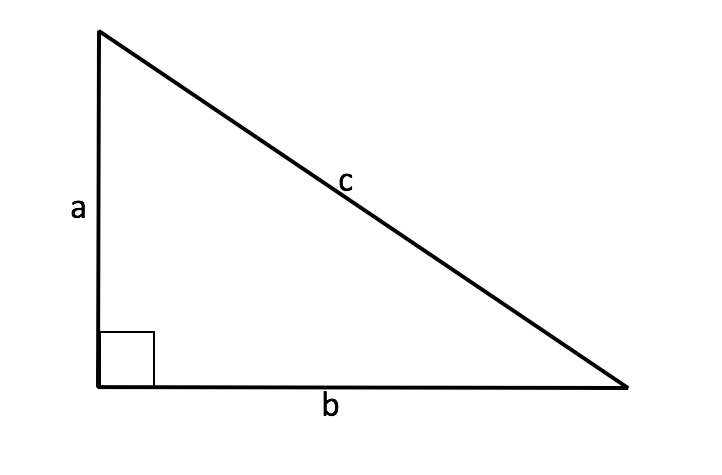
If a triangle appears in this format, then we can use the Pythagorean theorem to solve for any missing side. This formula is written in the following manner:

We can rearrange it in a number of ways to solve for each of the sides of the triangle. Let's rearrange it to solve for the hypotenuse, .

Rearrange and take the square root of both sides. 

Simplify.

Now, let's use the Pythagorean theorem to solve for one of the legs, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

Last, let's use the Pythagorean theorem to solve for the adjacent leg, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

It is important to note that we can only use the following formulas to solve for the missing side of a right triangle when two other sides are known:

After we find the missing side, we can use the perimeter formula to calculate the triangle's perimeter.  

Method 3:

This method is the most complicated method and can only be used when we know two side lengths of a triangle as well as the measure of the angle that is between them. When we know side-angle-side (SAS) information, we can use the Law of Cosines to find the missing side. In order for this formula to accurately calculate the missing side we need to label the triangle in the following manner:

When the triangle is labeled in this way each side directly corresponds to the angle directly opposite of it. If we label our triangle carefully, then we can use the following formulas to find missing sides in any triangle given SAS information:

After, we calculate the right side of the equation, we need to take the square root of both sides in order to obtain the final side length of the missing side. Last, we need to use the perimeter formula to obtain the distance of the side lengths of the polygon.

Solution:

Now, that we have discussed the three methods used to calculate the perimeter of a triangle, we can use this information to solve the problem.

The perimeter of a polygon is the sum of the lengths of its sides. We know the lengths of two sides of the triangle - the lengths of its legs, 9 and 12. We can find the length of the hypotenuse by way of the Pythagorean theorem:

Set , and solve for :

The perimeter is the sum of the lengths of the sides:

.

How do you find the perimeter of a right triangle?

There are three primary methods used to find the perimeter of a right triangle.

1. When side lengths are given, add them together.
2. Solve for a missing side using the Pythagorean theorem.
3. If we know side-angle-side information, solve for the missing side using the Law of Cosines.

Method 1:

This method will show you how to calculate the perimeter of a triangle when all sides lengths are known. Consider the following figure:

If we know the lengths of sides , , and , then we can simply add them together to find the perimeter of the triangle. It is important to note several things. First, we need to make sure that all the units given match one another. Second, when all the side lengths are known, then the perimeter formula may be used on all types of triangles (e.g. right, acute, obtuse, equilateral, isosceles, and scalene). The perimeter formula is written formally in the following format:

Method 2:

In right triangles, we can calculate the perimeter of a triangle when we are provided only two sides. We can do this by using the Pythagorean theorem. Let's first discuss right triangles in a general sense. A right triangle is a triangle that has one  angle. It is a special triangle and needs to be labeled accordingly. The legs of the triangle form the  angle and they are labeled  and . The side of the triangle that is opposite of the  angle and connects the two legs is known as the hypotenuse. The hypotenuse is the longest side of the triangle and is labeled as .

If a triangle appears in this format, then we can use the Pythagorean theorem to solve for any missing side. This formula is written in the following manner:

We can rearrange it in a number of ways to solve for each of the sides of the triangle. Let's rearrange it to solve for the hypotenuse, .

Rearrange and take the square root of both sides. 

Simplify.

Now, let's use the Pythagorean theorem to solve for one of the legs, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

Last, let's use the Pythagorean theorem to solve for the adjacent leg, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

It is important to note that we can only use the following formulas to solve for the missing side of a right triangle when two other sides are known:

After we find the missing side, we can use the perimeter formula to calculate the triangle's perimeter.  

Method 3:

This method is the most complicated method and can only be used when we know two side lengths of a triangle as well as the measure of the angle that is between them. When we know side-angle-side (SAS) information, we can use the Law of Cosines to find the missing side. In order for this formula to accurately calculate the missing side we need to label the triangle in the following manner:

When the triangle is labeled in this way each side directly corresponds to the angle directly opposite of it. If we label our triangle carefully, then we can use the following formulas to find missing sides in any triangle given SAS information:

After, we calculate the right side of the equation, we need to take the square root of both sides in order to obtain the final side length of the missing side. Last, we need to use the perimeter formula to obtain the distance of the side lengths of the polygon.

Solution:

Now, that we have discussed the three methods used to calculate the perimeter of a triangle, we can use this information to solve the problem.

First, we must determine the length of side B. To do this, we use the Pythagorean theorem, which shows that:

Through substitution, we know that:

Once we know the length of side B, we can find the perimeter by adding the lengths of each side:

Since the problem is given with the unit "inches" we know that the answer is ; converting this to feet gives us the correct answer:

How do you find the perimeter of a right triangle?

There are three primary methods used to find the perimeter of a right triangle.

1. When side lengths are given, add them together.
2. Solve for a missing side using the Pythagorean theorem.
3. If we know side-angle-side information, solve for the missing side using the Law of Cosines.

Method 1:

This method will show you how to calculate the perimeter of a triangle when all sides lengths are known. Consider the following figure:

If we know the lengths of sides , , and , then we can simply add them together to find the perimeter of the triangle. It is important to note several things. First, we need to make sure that all the units given match one another. Second, when all the side lengths are known, then the perimeter formula may be used on all types of triangles (e.g. right, acute, obtuse, equilateral, isosceles, and scalene). The perimeter formula is written formally in the following format:

Method 2:

In right triangles, we can calculate the perimeter of a triangle when we are provided only two sides. We can do this by using the Pythagorean theorem. Let's first discuss right triangles in a general sense. A right triangle is a triangle that has one  angle. It is a special triangle and needs to be labeled accordingly. The legs of the triangle form the  angle and they are labeled  and . The side of the triangle that is opposite of the  angle and connects the two legs is known as the hypotenuse. The hypotenuse is the longest side of the triangle and is labeled as .

If a triangle appears in this format, then we can use the Pythagorean theorem to solve for any missing side. This formula is written in the following manner:

We can rearrange it in a number of ways to solve for each of the sides of the triangle. Let's rearrange it to solve for the hypotenuse, .

Rearrange and take the square root of both sides. 

Simplify.

Now, let's use the Pythagorean theorem to solve for one of the legs, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

Last, let's use the Pythagorean theorem to solve for the adjacent leg, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

It is important to note that we can only use the following formulas to solve for the missing side of a right triangle when two other sides are known:

After we find the missing side, we can use the perimeter formula to calculate the triangle's perimeter.  

Method 3:

This method is the most complicated method and can only be used when we know two side lengths of a triangle as well as the measure of the angle that is between them. When we know side-angle-side (SAS) information, we can use the Law of Cosines to find the missing side. In order for this formula to accurately calculate the missing side we need to label the triangle in the following manner:

When the triangle is labeled in this way each side directly corresponds to the angle directly opposite of it. If we label our triangle carefully, then we can use the following formulas to find missing sides in any triangle given SAS information:

After, we calculate the right side of the equation, we need to take the square root of both sides in order to obtain the final side length of the missing side. Last, we need to use the perimeter formula to obtain the distance of the side lengths of the polygon.

Solution:

Now, that we have discussed the three methods used to calculate the perimeter of a triangle, we can use this information to solve the problem.

Before we can find the perimeter of this triangle, we need to find the length of side 'b'. Use the Pythagorean theorem and substitute.

Knowing that c=11 and a=9, we can find b.

Now that we know what b is, we can find the perimeter using this formula:

Basically, it is just adding the length of all sides.

How do you find the perimeter of a right triangle?

There are three primary methods used to find the perimeter of a right triangle.

1. When side lengths are given, add them together.
2. Solve for a missing side using the Pythagorean theorem.
3. If we know side-angle-side information, solve for the missing side using the Law of Cosines.

Method 1:

This method will show you how to calculate the perimeter of a triangle when all sides lengths are known. Consider the following figure:

If we know the lengths of sides , , and , then we can simply add them together to find the perimeter of the triangle. It is important to note several things. First, we need to make sure that all the units given match one another. Second, when all the side lengths are known, then the perimeter formula may be used on all types of triangles (e.g. right, acute, obtuse, equilateral, isosceles, and scalene). The perimeter formula is written formally in the following format:

Method 2:

In right triangles, we can calculate the perimeter of a triangle when we are provided only two sides. We can do this by using the Pythagorean theorem. Let's first discuss right triangles in a general sense. A right triangle is a triangle that has one  angle. It is a special triangle and needs to be labeled accordingly. The legs of the triangle form the  angle and they are labeled  and . The side of the triangle that is opposite of the  angle and connects the two legs is known as the hypotenuse. The hypotenuse is the longest side of the triangle and is labeled as .

If a triangle appears in this format, then we can use the Pythagorean theorem to solve for any missing side. This formula is written in the following manner:

We can rearrange it in a number of ways to solve for each of the sides of the triangle. Let's rearrange it to solve for the hypotenuse, .

Rearrange and take the square root of both sides. 

Simplify.

Now, let's use the Pythagorean theorem to solve for one of the legs, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

Last, let's use the Pythagorean theorem to solve for the adjacent leg, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

It is important to note that we can only use the following formulas to solve for the missing side of a right triangle when two other sides are known:

After we find the missing side, we can use the perimeter formula to calculate the triangle's perimeter.  

Method 3:

This method is the most complicated method and can only be used when we know two side lengths of a triangle as well as the measure of the angle that is between them. When we know side-angle-side (SAS) information, we can use the Law of Cosines to find the missing side. In order for this formula to accurately calculate the missing side we need to label the triangle in the following manner:

When the triangle is labeled in this way each side directly corresponds to the angle directly opposite of it. If we label our triangle carefully, then we can use the following formulas to find missing sides in any triangle given SAS information:

After, we calculate the right side of the equation, we need to take the square root of both sides in order to obtain the final side length of the missing side. Last, we need to use the perimeter formula to obtain the distance of the side lengths of the polygon.

Solution:

Now, that we have discussed the three methods used to calculate the perimeter of a triangle, we can use this information to solve the problem.

In order to calculate the perimeter we need to find the length of the hypotenuse using the Pythagorean theorem.

Rearrange.

Substitute in known values.

Now that we have found the missing side, we can substitute the values into the perimeter formula and solve.

How do you find the perimeter of a right triangle?

There are three primary methods used to find the perimeter of a right triangle.

1. When side lengths are given, add them together.
2. Solve for a missing side using the Pythagorean theorem.
3. If we know side-angle-side information, solve for the missing side using the Law of Cosines.

Method 1:

This method will show you how to calculate the perimeter of a triangle when all sides lengths are known. Consider the following figure:

If we know the lengths of sides , , and , then we can simply add them together to find the perimeter of the triangle. It is important to note several things. First, we need to make sure that all the units given match one another. Second, when all the side lengths are known, then the perimeter formula may be used on all types of triangles (e.g. right, acute, obtuse, equilateral, isosceles, and scalene). The perimeter formula is written formally in the following format:

Method 2:

In right triangles, we can calculate the perimeter of a triangle when we are provided only two sides. We can do this by using the Pythagorean theorem. Let's first discuss right triangles in a general sense. A right triangle is a triangle that has one  angle. It is a special triangle and needs to be labeled accordingly. The legs of the triangle form the  angle and they are labeled  and . The side of the triangle that is opposite of the  angle and connects the two legs is known as the hypotenuse. The hypotenuse is the longest side of the triangle and is labeled as .

If a triangle appears in this format, then we can use the Pythagorean theorem to solve for any missing side. This formula is written in the following manner:

We can rearrange it in a number of ways to solve for each of the sides of the triangle. Let's rearrange it to solve for the hypotenuse, .

Rearrange and take the square root of both sides. 

Simplify.

Now, let's use the Pythagorean theorem to solve for one of the legs, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

Last, let's use the Pythagorean theorem to solve for the adjacent leg, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

It is important to note that we can only use the following formulas to solve for the missing side of a right triangle when two other sides are known:

After we find the missing side, we can use the perimeter formula to calculate the triangle's perimeter.  

Method 3:

This method is the most complicated method and can only be used when we know two side lengths of a triangle as well as the measure of the angle that is between them. When we know side-angle-side (SAS) information, we can use the Law of Cosines to find the missing side. In order for this formula to accurately calculate the missing side we need to label the triangle in the following manner:

When the triangle is labeled in this way each side directly corresponds to the angle directly opposite of it. If we label our triangle carefully, then we can use the following formulas to find missing sides in any triangle given SAS information:

After, we calculate the right side of the equation, we need to take the square root of both sides in order to obtain the final side length of the missing side. Last, we need to use the perimeter formula to obtain the distance of the side lengths of the polygon.

Solution:

Now, that we have discussed the three methods used to calculate the perimeter of a triangle, we can use this information to solve the problem.

In order to calculate the perimeter we need to find the length of the hypotenuse using the Pythagorean theorem.

Rearrange.

Substitute in known values.

Now that we have found the missing side, we can substitute the values into the perimeter formula and solve.

How do you find the perimeter of a right triangle?

There are three primary methods used to find the perimeter of a right triangle.

1. When side lengths are given, add them together.
2. Solve for a missing side using the Pythagorean theorem.
3. If we know side-angle-side information, solve for the missing side using the Law of Cosines.

Method 1:

This method will show you how to calculate the perimeter of a triangle when all sides lengths are known. Consider the following figure:

If we know the lengths of sides , , and , then we can simply add them together to find the perimeter of the triangle. It is important to note several things. First, we need to make sure that all the units given match one another. Second, when all the side lengths are known, then the perimeter formula may be used on all types of triangles (e.g. right, acute, obtuse, equilateral, isosceles, and scalene). The perimeter formula is written formally in the following format:

Method 2:

In right triangles, we can calculate the perimeter of a triangle when we are provided only two sides. We can do this by using the Pythagorean theorem. Let's first discuss right triangles in a general sense. A right triangle is a triangle that has one  angle. It is a special triangle and needs to be labeled accordingly. The legs of the triangle form the  angle and they are labeled  and . The side of the triangle that is opposite of the  angle and connects the two legs is known as the hypotenuse. The hypotenuse is the longest side of the triangle and is labeled as .

If a triangle appears in this format, then we can use the Pythagorean theorem to solve for any missing side. This formula is written in the following manner:

We can rearrange it in a number of ways to solve for each of the sides of the triangle. Let's rearrange it to solve for the hypotenuse, .

Rearrange and take the square root of both sides. 

Simplify.

Now, let's use the Pythagorean theorem to solve for one of the legs, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

Last, let's use the Pythagorean theorem to solve for the adjacent leg, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

It is important to note that we can only use the following formulas to solve for the missing side of a right triangle when two other sides are known:

After we find the missing side, we can use the perimeter formula to calculate the triangle's perimeter.  

Method 3:

This method is the most complicated method and can only be used when we know two side lengths of a triangle as well as the measure of the angle that is between them. When we know side-angle-side (SAS) information, we can use the Law of Cosines to find the missing side. In order for this formula to accurately calculate the missing side we need to label the triangle in the following manner:

When the triangle is labeled in this way each side directly corresponds to the angle directly opposite of it. If we label our triangle carefully, then we can use the following formulas to find missing sides in any triangle given SAS information:

After, we calculate the right side of the equation, we need to take the square root of both sides in order to obtain the final side length of the missing side. Last, we need to use the perimeter formula to obtain the distance of the side lengths of the polygon.

Solution:

Now, that we have discussed the three methods used to calculate the perimeter of a triangle, we can use this information to solve the problem. In order to find the length of the hypotenuse, use the Pythagorean theorem:

, where  and  are the legs of the triangle, and  is the hypotenuse.

The hypotenuse is 10 inches long.

To find the perimeter, simply add up the three side lengths:

How do you find the perimeter of a right triangle?

There are three primary methods used to find the perimeter of a right triangle.

1. When side lengths are given, add them together.
2. Solve for a missing side using the Pythagorean theorem.
3. If we know side-angle-side information, solve for the missing side using the Law of Cosines.

Method 1:

This method will show you how to calculate the perimeter of a triangle when all sides lengths are known. Consider the following figure:

If we know the lengths of sides , , and , then we can simply add them together to find the perimeter of the triangle. It is important to note several things. First, we need to make sure that all the units given match one another. Second, when all the side lengths are known, then the perimeter formula may be used on all types of triangles (e.g. right, acute, obtuse, equilateral, isosceles, and scalene). The perimeter formula is written formally in the following format:

Method 2:

In right triangles, we can calculate the perimeter of a triangle when we are provided only two sides. We can do this by using the Pythagorean theorem. Let's first discuss right triangles in a general sense. A right triangle is a triangle that has one  angle. It is a special triangle and needs to be labeled accordingly. The legs of the triangle form the  angle and they are labeled  and . The side of the triangle that is opposite of the  angle and connects the two legs is known as the hypotenuse. The hypotenuse is the longest side of the triangle and is labeled as .

If a triangle appears in this format, then we can use the Pythagorean theorem to solve for any missing side. This formula is written in the following manner:

We can rearrange it in a number of ways to solve for each of the sides of the triangle. Let's rearrange it to solve for the hypotenuse, .

Rearrange and take the square root of both sides. 

Simplify.

Now, let's use the Pythagorean theorem to solve for one of the legs, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

Last, let's use the Pythagorean theorem to solve for the adjacent leg, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

It is important to note that we can only use the following formulas to solve for the missing side of a right triangle when two other sides are known:

After we find the missing side, we can use the perimeter formula to calculate the triangle's perimeter.  

Method 3:

This method is the most complicated method and can only be used when we know two side lengths of a triangle as well as the measure of the angle that is between them. When we know side-angle-side (SAS) information, we can use the Law of Cosines to find the missing side. In order for this formula to accurately calculate the missing side we need to label the triangle in the following manner:

When the triangle is labeled in this way each side directly corresponds to the angle directly opposite of it. If we label our triangle carefully, then we can use the following formulas to find missing sides in any triangle given SAS information:

After, we calculate the right side of the equation, we need to take the square root of both sides in order to obtain the final side length of the missing side. Last, we need to use the perimeter formula to obtain the distance of the side lengths of the polygon.

Solution:

Now, that we have discussed the three methods used to calculate the perimeter of a triangle, we can use this information to solve the problem.

In order to find the perimeter, we need to know all three side lengths. To discover the missing leg, we can use the Pythagorean theorem. Note that we actually know the hypotenuse, , so we're solving for either  or , one of the legs:

 subtract 16 from both sides

 take the square root

Now that we have our missing side, we can find the perimeter by adding all three sides together:

How do you find the perimeter of a right triangle?

There are three primary methods used to find the perimeter of a right triangle.

1. When side lengths are given, add them together.
2. Solve for a missing side using the Pythagorean theorem.
3. If we know side-angle-side information, solve for the missing side using the Law of Cosines.

Method 1:

This method will show you how to calculate the perimeter of a triangle when all sides lengths are known. Consider the following figure:

If we know the lengths of sides , , and , then we can simply add them together to find the perimeter of the triangle. It is important to note several things. First, we need to make sure that all the units given match one another. Second, when all the side lengths are known, then the perimeter formula may be used on all types of triangles (e.g. right, acute, obtuse, equilateral, isosceles, and scalene). The perimeter formula is written formally in the following format:

Method 2:

In right triangles, we can calculate the perimeter of a triangle when we are provided only two sides. We can do this by using the Pythagorean theorem. Let's first discuss right triangles in a general sense. A right triangle is a triangle that has one  angle. It is a special triangle and needs to be labeled accordingly. The legs of the triangle form the  angle and they are labeled  and . The side of the triangle that is opposite of the  angle and connects the two legs is known as the hypotenuse. The hypotenuse is the longest side of the triangle and is labeled as .

If a triangle appears in this format, then we can use the Pythagorean theorem to solve for any missing side. This formula is written in the following manner:

We can rearrange it in a number of ways to solve for each of the sides of the triangle. Let's rearrange it to solve for the hypotenuse, .

Rearrange and take the square root of both sides. 

Simplify.

Now, let's use the Pythagorean theorem to solve for one of the legs, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

Last, let's use the Pythagorean theorem to solve for the adjacent leg, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

It is important to note that we can only use the following formulas to solve for the missing side of a right triangle when two other sides are known:

After we find the missing side, we can use the perimeter formula to calculate the triangle's perimeter.  

Method 3:

This method is the most complicated method and can only be used when we know two side lengths of a triangle as well as the measure of the angle that is between them. When we know side-angle-side (SAS) information, we can use the Law of Cosines to find the missing side. In order for this formula to accurately calculate the missing side we need to label the triangle in the following manner:

When the triangle is labeled in this way each side directly corresponds to the angle directly opposite of it. If we label our triangle carefully, then we can use the following formulas to find missing sides in any triangle given SAS information:

After, we calculate the right side of the equation, we need to take the square root of both sides in order to obtain the final side length of the missing side. Last, we need to use the perimeter formula to obtain the distance of the side lengths of the polygon.

Solution:

Now, that we have discussed the three methods used to calculate the perimeter of a triangle, we can use this information to solve the problem. In this problem, we've been given the two legs of the right triangle. In order to find the perimeter, we have to know the hypotenuse as well. In order to figure out that length, use the Pythagorean theorem:

where a and b are the lengths of the legs and c is the length of the hypotenuse.

take the square root of both sides

To find the perimeter, just add

How do you find the perimeter of a right triangle?

There are three primary methods used to find the perimeter of a right triangle.

1. When side lengths are given, add them together.
2. Solve for a missing side using the Pythagorean theorem.
3. If we know side-angle-side information, solve for the missing side using the Law of Cosines.

Method 1:

This method will show you how to calculate the perimeter of a triangle when all sides lengths are known. Consider the following figure:

If we know the lengths of sides , , and , then we can simply add them together to find the perimeter of the triangle. It is important to note several things. First, we need to make sure that all the units given match one another. Second, when all the side lengths are known, then the perimeter formula may be used on all types of triangles (e.g. right, acute, obtuse, equilateral, isosceles, and scalene). The perimeter formula is written formally in the following format:

Method 2:

In right triangles, we can calculate the perimeter of a triangle when we are provided only two sides. We can do this by using the Pythagorean theorem. Let's first discuss right triangles in a general sense. A right triangle is a triangle that has one  angle. It is a special triangle and needs to be labeled accordingly. The legs of the triangle form the  angle and they are labeled  and . The side of the triangle that is opposite of the  angle and connects the two legs is known as the hypotenuse. The hypotenuse is the longest side of the triangle and is labeled as .

If a triangle appears in this format, then we can use the Pythagorean theorem to solve for any missing side. This formula is written in the following manner:

We can rearrange it in a number of ways to solve for each of the sides of the triangle. Let's rearrange it to solve for the hypotenuse, .

Rearrange and take the square root of both sides. 

Simplify.

Now, let's use the Pythagorean theorem to solve for one of the legs, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

Last, let's use the Pythagorean theorem to solve for the adjacent leg, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

It is important to note that we can only use the following formulas to solve for the missing side of a right triangle when two other sides are known:

After we find the missing side, we can use the perimeter formula to calculate the triangle's perimeter.  

Method 3:

This method is the most complicated method and can only be used when we know two side lengths of a triangle as well as the measure of the angle that is between them. When we know side-angle-side (SAS) information, we can use the Law of Cosines to find the missing side. In order for this formula to accurately calculate the missing side we need to label the triangle in the following manner:

When the triangle is labeled in this way each side directly corresponds to the angle directly opposite of it. If we label our triangle carefully, then we can use the following formulas to find missing sides in any triangle given SAS information:

After, we calculate the right side of the equation, we need to take the square root of both sides in order to obtain the final side length of the missing side. Last, we need to use the perimeter formula to obtain the distance of the side lengths of the polygon.

Solution:

Now, that we have discussed the three methods used to calculate the perimeter of a triangle, we can use this information to solve the problem.

To find the perimeter, first we need to know all three side lengths. To find the missing hypotenuse, use the Pythagorean theorem:

Now that we have all three side lengths, we can add them together to find the perimeter:

How do you find the perimeter of a right triangle?

There are three primary methods used to find the perimeter of a right triangle.

1. When side lengths are given, add them together.
2. Solve for a missing side using the Pythagorean theorem.
3. If we know side-angle-side information, solve for the missing side using the Law of Cosines.

Method 1:

This method will show you how to calculate the perimeter of a triangle when all sides lengths are known. Consider the following figure:

If we know the lengths of sides , , and , then we can simply add them together to find the perimeter of the triangle. It is important to note several things. First, we need to make sure that all the units given match one another. Second, when all the side lengths are known, then the perimeter formula may be used on all types of triangles (e.g. right, acute, obtuse, equilateral, isosceles, and scalene). The perimeter formula is written formally in the following format:

Method 2:

In right triangles, we can calculate the perimeter of a triangle when we are provided only two sides. We can do this by using the Pythagorean theorem. Let's first discuss right triangles in a general sense. A right triangle is a triangle that has one  angle. It is a special triangle and needs to be labeled accordingly. The legs of the triangle form the  angle and they are labeled  and . The side of the triangle that is opposite of the  angle and connects the two legs is known as the hypotenuse. The hypotenuse is the longest side of the triangle and is labeled as .

If a triangle appears in this format, then we can use the Pythagorean theorem to solve for any missing side. This formula is written in the following manner:

We can rearrange it in a number of ways to solve for each of the sides of the triangle. Let's rearrange it to solve for the hypotenuse, .

Rearrange and take the square root of both sides. 

Simplify.

Now, let's use the Pythagorean theorem to solve for one of the legs, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

Last, let's use the Pythagorean theorem to solve for the adjacent leg, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

It is important to note that we can only use the following formulas to solve for the missing side of a right triangle when two other sides are known:

After we find the missing side, we can use the perimeter formula to calculate the triangle's perimeter.  

Method 3:

This method is the most complicated method and can only be used when we know two side lengths of a triangle as well as the measure of the angle that is between them. When we know side-angle-side (SAS) information, we can use the Law of Cosines to find the missing side. In order for this formula to accurately calculate the missing side we need to label the triangle in the following manner:

When the triangle is labeled in this way each side directly corresponds to the angle directly opposite of it. If we label our triangle carefully, then we can use the following formulas to find missing sides in any triangle given SAS information:

After, we calculate the right side of the equation, we need to take the square root of both sides in order to obtain the final side length of the missing side. Last, we need to use the perimeter formula to obtain the distance of the side lengths of the polygon.

Solution:

Now, that we have discussed the three methods used to calculate the perimeter of a triangle, we can use this information to solve the problem. In order to find perimeter, you must first find the length of the hypotenuse. You can either use the Pythagorean theorem or realize this is one of the "special triangles", particularly a   triangle. The perimeter is the sum of these three lengths.

Therefore, the answer is .