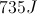All AP Physics C Electricity Resources
Example Questions
Example Question #111 : Ap Physics C
A satellite of mass 



Since the gravitational force cannot exert torque on the satellite about the Earth's center, angular momentum is conserved in this orbit:
Example Question #111 : Ap Physics C
A satellite is in an elliptical orbit about the Earth. If the satellite needs to enter a circular orbit at the apogee of the ellipse, in what direction will it need to accelerate?
This maneuver cannot be accomplished
In the direction of travel
Towards Earth
Opposite the direction of travel
Away from Earth
In the direction of travel
Since the speed of the satellite at apogee is too low for a circular orbit at that orbital radius, the satellite needs to speed up to circularize the orbit.
Example Question #1 : Understanding Newton's Laws
A 

Relevant equations:
Determine the clockwise torque caused by the bucket.
Write an expression for the counterclockwise torque caused by the rope.
Combine the torque of the rope and the torque of the bucket into the net torque equation.
Since the system has no angular acceleration, net torque must be zero, allowing us to solve for the force of the rope.
Example Question #111 : Mechanics Exam
An object at rest will remain at rest unless acted on by a(n) __________.
external force
internal force
negative force
positive force
external force
The correct answer is external force. External forces applied to an object will result in non-zero acceleration, causing the object to move. In contrast, internal forces contribute to the properties of the object and do not result in acceleration of the object.
Either positive or negative forces can result in the acceleration of an object. The sign on the force simply conveys information about its relative direction.
Example Question #112 : Ap Physics C
A 1000kg rocket has an engine capable of producing a force of 30000N. By the third law of motion, when the rocket launches it experiences a reaction force that pushes it upwards of equal magnitude to the force produced by the engine. What is the acceleration of the rocket?


When the rocket launches it produces a downward force of 30000N. Due to the third law of motion, the rocket experiences a 30000N reaction force that pushes it upwards.
In addition, the rocket experiences the downward force of its own weight. This is given by:

We know that 
We know the mass of the rocket is 1000kg, so we need only to find the net force to solve for acceleration.
We know that 


Finally we solve for acceleration:
Example Question #113 : Ap Physics C

Three boxes tied by two ropes move across a frictionless surface pulled by a force 
None of these
Since the boxes are all connected by ropes, we know that the acceleration of each box is exactly the same. They all move simultaneously, in tandem, with the same velocity and acceleration.
A quick analysis of each box will produce a very simple system of equations. Each rope experiences some tension, so we will label the tension experienced by the rope between 





For the box on the right (of mass 



We get the equation: 


For the middle box (of mass 



So we get the equation: 


Finally, for the box on the left (of mass 


So we get the equation: 


Now it is just a matter of simple substitution. We have three equations:
From them we can get an expression for the force. Isolate 
Substitute the expression of 
Use this value of 
So we have:
Solve for acceleration.
Example Question #114 : Ap Physics C

A box is being pushed against a wall by a force 

None of these
This question requires a 2-dimensional analysis. First identify all the forces acting on the box. Since the box is being pushed against a surface it automatically experiences a normal force 



The key is that the box should NOT move.
For the horizontal axis, net force must be zero. The two horizontal forces are the applied force and the normal force.
Now, on the vertical axis we have fiction and the object's weight:
Finally, we use the equation for friction force to solve the problem.
Substitute the weight, since it is equal to the force of friction.
Isolate the normal force.
Since the normal force is equal to the applied force, this is our final expression.
Example Question #42 : Forces

A box of mass 10kg is pulled by a force as shown in the diagram. The surface is frictionless. How much force is the box experiencing along the horizontal axis?
You need only to obtain the horizontal component of the force. To do this you must use trigonometric properties.
We see that the force makes an angle of 60º with the horizontal axis. This means that the horizontal component of the force is adjacent to this angle. We can view the diagram as a triangle. From trigonometry we know that to calculate the adjacent side of a triangle we need to multiply the hypotenuse by the cosine of the angle.
We can solve for the horizontal component of the force using the applied force as the hypotenuse:
Example Question #111 : Ap Physics C
A 25kg child climbs up a tree. How much work is required for him to climb up this tree to a height of three meters?
The forces acting on the child are the force of gravity and the upward force provided by the child himself. Choosing the upward direction as positive, Newton's second law applied to the child gives the following equation.
To calculate the work done by the child to bring himself three meters up the tree, we use the work equation below.
Example Question #112 : Ap Physics C
A force of 27N is applied horizontally to a box resting on a level surface. The surface exerts a friction force of 2N as the box moves. If it moves a total of 3.7m, how much work was done on the box?
The net force on the box is the applied force minus the friction force, since friction acts in the direction opposite motion.
Consequently, the net force is:
The formula for work is:
Substituting in our net force and distance, we find that the work done on the box is:
Certified Tutor
All AP Physics C Electricity Resources