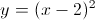We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

Algebra 1 : Graphing

Study concepts, example questions & explanations for Algebra 1

Create An Account

All Algebra 1 Resources

10 Diagnostic Tests 557 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : How To Graph A Quadratic Function

What is the equation of a parabola with vertex (4,1) and -intercept (0,-7) ?

Possible Answers:

$y = \frac{1}{2} x^{2} -4x - 7$

$y = -\frac{1}{2} x^{2} +4x - 7$

$y = \frac{1}{2} x^{2} +8x - 7$

$y = \frac{1}{2} x^{2} -8x - 7$

$y = -\frac{1}{2} x^{2} -4x - 7$

Correct answer:

$y = -\frac{1}{2} x^{2} +4x - 7$

Explanation:

From the vertex, we know that the equation of the parabola will take the form $y = a (x - 4) ^{2}+ 1$ for some .

To calculate that , we plug in the values from the other point we are given, x = 0, y=-7 , and solve for :

$y = a (x - 4)^{2} + 1$

$-7= a \cdot (0 - 4)^{2} + 1$

$-7= a \cdot (- 4)^{2} + 1$

-7= 16a + 1

16a = -8

$a = -\frac{1}{2}$

Now the equation is $y = -\frac{1}{2} (x - 4)^{2} + 1$ . This is not an answer choice, so we need to rewrite it in some way.

Expand the squared term:

$y = -\frac{1}{2} (x^{2} -8x + 16) + 1$

Distribute the fraction through the parentheses:

$y = -\frac{1}{2} x^{2} +4x - 8 + 1$

Combine like terms:

$y = -\frac{1}{2} x^{2} +4x - 7$

Report an Error

Example Question #1 : How To Graph A Quadratic Function

Possible Answers:

$y = x^{2}$

$y = (x-2)^{2}$

$y = -(x-2)^{2} - 2$

$y = (x+3)^{2}$

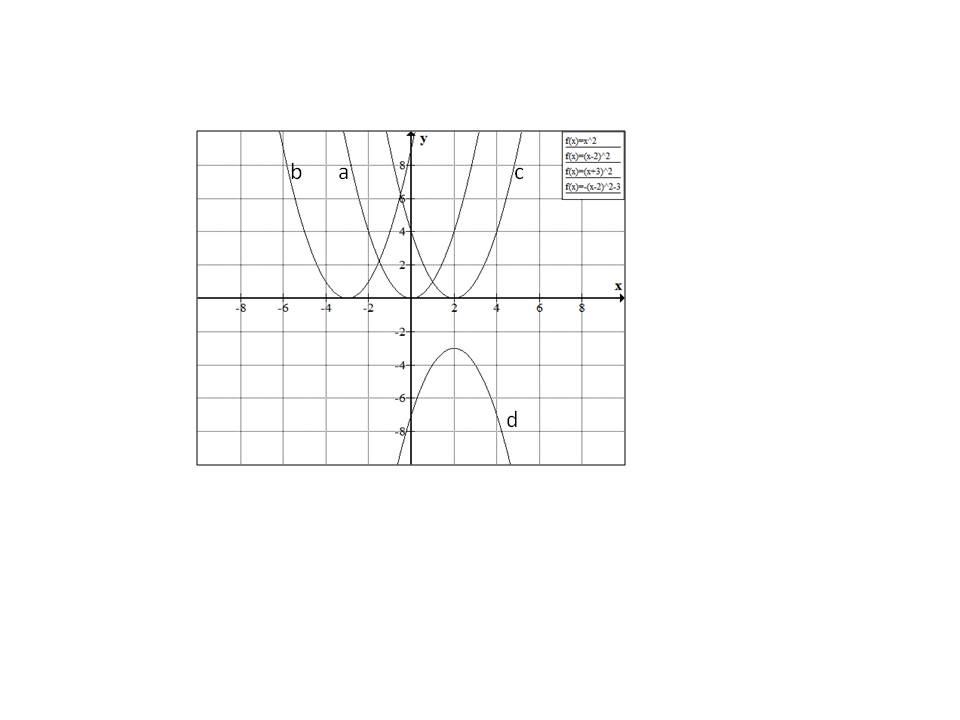
None of the above

Correct answer:

$y = (x+3)^{2}$

Explanation:

Starting with $y = x^{2}$

$(x-2)^{2}$ moves the parabola $x^{2}$ by units to the right.

Similarly $(x+3)^{2}$ moves the parabola by units to the left.

Hence the correct answer is option .

Report an Error

Example Question #41 : Graphing

Which of the following graphs matches the function y=(x-1)^2-2 ?

Possible Answers:

Graph4

Graph3

Graph1

Graph

Graph2

Correct answer:

Graph

Explanation:

Start by visualizing the graph associated with the function :

Graph5

Terms within the parentheses associated with the squared x-variable will shift the parabola horizontally, while terms outside of the parentheses will shift the parabola vertically. In the provided equation, 2 is located outside of the parentheses and is subtracted from the terms located within the parentheses; therefore, the parabola in the graph will shift down by 2 units. A simplified graph of looks like this:

Graph6

Remember that there is also a term within the parentheses. Within the parentheses, 1 is subtracted from the x-variable; thus, the parabola in the graph will shift to the right by 1 unit. As a result, the following graph matches the given function :

Graph

Report an Error

Example Question #1 : How To Graph An Absolute Value Function

Absolute_value

Which of these would most likely be the equation corresponding to the above graph?

Possible Answers:

y = |x + 2 | +1

y = |x + 3 |

y = |x + 2 | -1

y = |x - 2 | +1

y = |x - 2 | -1

Correct answer:

y = |x - 2 | -1

Explanation:

This is an absolute value graph. Its equation takes the form y = |x-h|+k , in which h,k represent the number of units that the base graph y = |x| is translated right and up respectively.

Since the graph of y = |x| is translated two units right and one unit down, h = 2 and k = -1 , so the equation would be:

y = |x-2|+(-1)

y = |x - 2 | -1

Report an Error

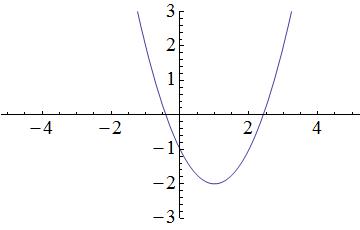
Example Question #2 : How To Graph An Absolute Value Function

Give the -intercept(s) of the graph of the function $f(x) = 7 - \left | 2x-3 \right |$

Possible Answers:

(-2,0) , (5,0)

The graph has no -intercepts.

(-2,0) , (2,0)

(-5,0) , (2,0)

(-5,0) , (5,0)

Correct answer:

(-2,0) , (5,0)

Explanation:

To find the -intercept(s) of the graph, set f(x) = 0 and solve for .

$f(x) = 7 - \left | 2x-3 \right |$

$0= 7 - \left | 2x-3 \right |$

$\left | 2x-3 \right |= 7$

Rewrite this as the compound equation:

2x-3= -7 or 2x-3= 7

Solve each separately:

2x-3= -7

2x-3 + 3 = -7+ 3

2x = -4

$2x \div 2 = -4\div 2$

x = -2

2x-3= 7

2x-3 + 3 = 7+ 3

2x = 10

$2x \div 2 = 10\div 2$

x = 5

There are two -intercepts: (-2,0) , (5,0)

Report an Error

Example Question #1 : How To Graph An Absolute Value Function

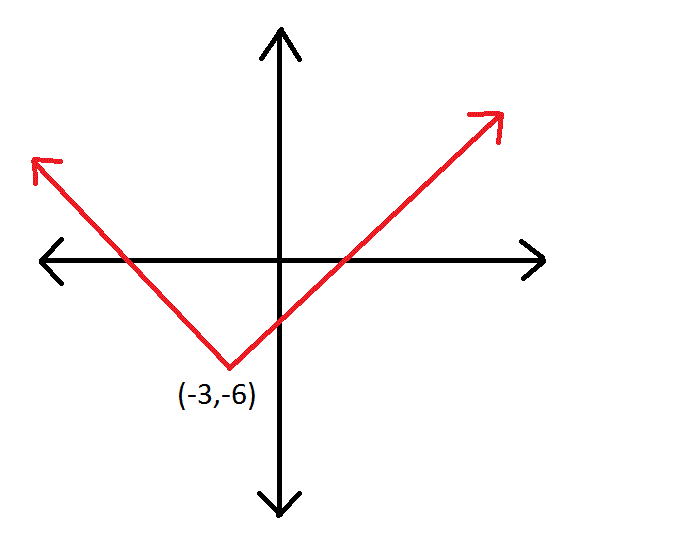
Absolute_value

Which of these would most likely be the equation corresponding to the above graph?

Possible Answers:

y = | x - 6 | - 3

y = | x - 3 | - 6

y = | x - 6 | + 3

y = | x + 3 | - 6

y = | x + 6 | - 3

Correct answer:

y = | x + 3 | - 6

Explanation:

This is an absolute value graph. Its equation takes the form y = |x-h|+k , in which h,k represent the number of units that the base graph y = |x| is translated right and up respectively.

Since the graph of y = |x| is translated three units left and six units down, h = -3 and k = -6 .

Plug these values into the general form of the equation:

y = |x-(-3)|+(-6)

Simplify:

y = |x+3|-6

Report an Error

Example Question #1 : How To Graph An Absolute Value Function

$\textup{What is the slope of } y=-4\left | x-2\right | \textup{ when } x=1?$

Possible Answers:

$\textup{It does not exist.}$

Correct answer:

Explanation:

$\textup{When }x=1\textup{ the expression inside the absolute value is negative,} \\\textup{so the function can be written as } y=-4(2-x)=4x-8,\textup{ with a slope of 4.}\\\textup{Plus, the slope changes to -4 when } x>2. \textup{ When } x=2 \textup{ it does not exist.}$

Report an Error

Example Question #2 : How To Graph An Absolute Value Function

$\textup{Find the lowest attainable value of }y \textup{ in } y=-4+ \left |4x^2-25 \right |.$

Possible Answers:

2.5

-2.5

Correct answer:

Explanation:

$\\ \left | 4x^2-25\right | \textup{cannot get any lower than zero,}\\\textup{so\textit{ y} cannot get any lower than} -4.$

Report an Error

Example Question #2 : How To Graph An Absolute Value Function

$\textup{Which is an \textit{x}-intercept of the function } y=\left | -x^2-8x-16\right |-4.$

Possible Answers:

$\textup{None of the above.}$

Correct answer:

Explanation:

$\\ \textup{The function can be simplified as } \\y=\left | -(x+4)^2\right |-4=\left |(x+4)^2\right |-4=(x+4)^2-4.\\\textup{In order to find the \textit{x}-intercecpt, we solve the equation}\\0=(x+4)^2-4 \textup{ which becomes }\\(x+4)=\pm2\textup{ and has solution } -2 \textup{ and } -6.$

Report an Error

View Algebra Tutors

Dejuan
Certified Tutor

University of Illinois at Urbana-Champaign, Bachelor of Science, Electrical Engineering.

View Algebra Tutors

Yucheng
Certified Tutor

The University of Texas at Austin, Bachelor of Science, Mechanical Engineering.

View Algebra Tutors

Dilip
Certified Tutor

University of Calcutta, Bachelor of Science, Physics. Indiana Institute of Technology, Master of Science, Physics.

All Algebra 1 Resources

10 Diagnostic Tests 557 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

SSAT Tutors in San Diego, French Tutors in Phoenix, ISEE Tutors in Philadelphia, GRE Tutors in Denver, Calculus Tutors in San Francisco-Bay Area, LSAT Tutors in San Diego, Math Tutors in Chicago, ACT Tutors in Phoenix, Spanish Tutors in Los Angeles, Spanish Tutors in Phoenix

Popular Courses & Classes

Spanish Courses & Classes in Chicago, SAT Courses & Classes in San Diego, SAT Courses & Classes in Seattle, Spanish Courses & Classes in Philadelphia, LSAT Courses & Classes in Houston, ACT Courses & Classes in Denver, SSAT Courses & Classes in Houston, GMAT Courses & Classes in Washington DC, SAT Courses & Classes in San Francisco-Bay Area, GRE Courses & Classes in San Diego

Popular Test Prep

SAT Test Prep in Dallas Fort Worth, GRE Test Prep in Chicago, GMAT Test Prep in Los Angeles, SAT Test Prep in San Francisco-Bay Area, GMAT Test Prep in Boston, GRE Test Prep in Seattle, GMAT Test Prep in Miami, ISEE Test Prep in Dallas Fort Worth, GRE Test Prep in Atlanta, GRE Test Prep in Los Angeles

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Algebra 1 : Graphing

Study concepts, example questions & explanations for Algebra 1

All Algebra 1 Resources

Example Questions

Example Question #1 : How To Graph A Quadratic Function

Example Question #1 : How To Graph A Quadratic Function

Example Question #41 : Graphing

Example Question #1 : How To Graph An Absolute Value Function

Example Question #2 : How To Graph An Absolute Value Function

Example Question #1 : How To Graph An Absolute Value Function

Example Question #1 : How To Graph An Absolute Value Function

Example Question #2 : How To Graph An Absolute Value Function

Example Question #2 : How To Graph An Absolute Value Function

All Algebra 1 Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: