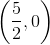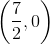Call Now to Set Up Tutoring:

(888) 888-0446

SSAT Upper Level Math : SSAT Upper Level Quantitative (Math)

Study concepts, example questions & explanations for SSAT Upper Level Math

Create An Account

All SSAT Upper Level Math Resources

6 Diagnostic Tests 311 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #631 : Ssat Upper Level Quantitative (Math)

Add 4+ 7i to its complex conjugate. What is the result?

Possible Answers:

11-3i

11 + 11i

14i

Correct answer:

Explanation:

The complex conjugate of a complex number a + bi is a - bi , so 4+7i has 4-7i as its complex conjugate; the sum of the two numbers is

(4+7i)+ (4-7i) = 4+4 + 7i - 7i = 8

Report an Error

Example Question #94 : Graphing

Multiply the complex conjugate of 3 + 7i by . What is the result?

Possible Answers:

-12 + 28i

-28+12i

12 + 28 i

28 + 12i

12 - 28 i

Correct answer:

28 + 12i

Explanation:

The complex conjugate of a complex number a + bi is a - bi , so the complex conjugate of 3 + 7i is 3 - 7i . Multiply this by :

$(3-7i) \cdot 4i = 3 \cdot 4i - 7 i \cdot 4i$

$= 12i - 7 \cdot 4 \cdot i \cdot i$

$= 12i -28 \cdot (-1)$

= 12i + 28

= 28 + 12i

Report an Error

Example Question #95 : Graphing

Multiply the complex conjugate of 8 by 5 i . What is the result?

Possible Answers:

40i

None of the other responses gives the correct product.

-40i

Correct answer:

40i

Explanation:

The complex conjugate of a complex number a + bi is a - bi . Since 8=8+0i , its complex conjugate is 8-0i = 8 itself. Multiply this by :

$8 \cdot 5i = 40 i$

Report an Error

Example Question #96 : Graphing

Multiply the complex conjugate of by 3 i . What is the result?

Possible Answers:

21i

-21i

None of the other responses gives the correct product.

Correct answer:

Explanation:

The complex conjugate of a complex number a + bi is a - bi . Since 7i = 0+7i , its complex conjugate is 0-7i = -7i .

Multiply this by :

Recall that by definition i^2=-1 .

$-7i \cdot 3i = -7 \cdot 3 \cdot i \cdot i = -21 (-1) = 21$

Report an Error

Example Question #21 : How To Graph Complex Numbers

Multiply the following complex numbers:

$\left (5 + i \right )\left (5 + 2i \right )$

Possible Answers:

27 - 15i

23 + 5i

27 + 5i

23 - 15i

23 + 15i

Correct answer:

23 + 15i

Explanation:

FOIL the product out:

$\left (5 + i \right )\left (5 + 2i \right )$

To FOIL multiply the first terms from each binomial together, multiply the outer terms of both terms together, multiply the inner terms from both binomials together, and finally multiply the last terms from each binomial together.

$=5 \cdot 5 + 5 \cdot 2i + 5 \cdot i + i\cdot 2i$

$=25 + 10i + 5 i + 2i^{2}$

Recall that i is an imaginary number and by definition i^2=-1 . Substituting this into the function is as follows.

=25 + 10i + 5 i + 2 (-1)

=25 -2 + 10i + 5 i

=23 + 15i

Report an Error

Example Question #1 : How To Graph Inverse Variation

Give the equation of the vertical asymptote of the graph of the equation $y = \frac{7}{9x+4}$ .

Possible Answers:

$x= \frac{7}{9}$

$x= - \frac{7}{4}$

x = 9

$x= -\frac{4}{9}$

x=-4

Correct answer:

$x= -\frac{4}{9}$

Explanation:

The vertical asymptote of an inverse variation function is the vertical line of the equation x = a , where is the value for which the expression is not defined. To find , set the denominator to and solve for :

9x+4 = 0

9x= -4

$x= -\frac{4}{9}$ is the equation of the asymptote.

Report an Error

Example Question #1 : How To Graph Inverse Variation

Give the -intercept of the graph of the equation $y = \frac{5}{2x-7}$ .

Possible Answers:

$\left ( \frac{5}{2}, 0 \right )$

(5,0)

(7,0)

The graph has no -intercept.

$\left ( \frac{7}{2}, 0 \right )$

Correct answer:

The graph has no -intercept.

Explanation:

The -intercept of the graph of an equation is the point at which it intersects the -axis. Its -coordinate is 0, so set y = 0 and solve for :

$y = \frac{5}{2x-7}$

$0= \frac{5}{2x-7}$

$0\cdot (2x-7) = \frac{5}{2x-7} \cdot (2x-7)$

0 = 5

This is identically false, so the graph has no -intercept.

Report an Error

Example Question #3 : How To Graph Inverse Variation

Give the -intercept of the graph of the equation $y = \frac{3}{5x-8}$ .

Possible Answers:

$\left ( 0, -\frac{3}{5}\right )$

The graph has no -intercept.

$\left ( 0, \frac{8}{5}\right )$

$\left ( 0, -\frac{3}{8}\right )$

(0,8)

Correct answer:

$\left ( 0, -\frac{3}{8}\right )$

Explanation:

The -intercept of the graph of an equation is the point at which it intersects the -axis. Its -coordinate is 0, so set x = 0 and solve for :

$y = \frac{3}{5x-8}$

$y = \frac{3}{5 (0)-8}$

$y = \frac{3}{0-8}$

$y =- \frac{3}{8}$

$\left ( 0, -\frac{3}{8}\right )$ is the -intercept.

Report an Error

Example Question #1 : How To Graph Inverse Variation

Give the slope of the line that passes through the - and -intercepts of the graph of the equation $y = \frac{6}{8x-2}$ .

Possible Answers:

$-\frac{1}{3}$

The line cannot exist as described.

$\frac{4}{3}$

$\frac{3}{4}$

Correct answer:

The line cannot exist as described.

Explanation:

The graph of $y = \frac{6}{8x-2}$ does not have an -intercept. If it did, then it would be the point on the graph with -coordinate 0. If we were to make this substitution, the equation would be

$0= \frac{6}{8x-2}$

and

$0 \cdot (8x-2)= \frac{6}{8x-2} \cdot (8x-2)$

0=6

This is identically false, so the graph has no -intercept. Therefore, the line cannot exist as described.

Report an Error

Example Question #5 : How To Graph Inverse Variation

Give the -coordinate of a point with a positive -coordinate at which the graphs of the equations $y = \frac{4}{x}$ and x+3y = 4 intersect.

Possible Answers:

$\frac{2}{3}$

$\frac{4}{3}$

The graphs of the equations do not intersect.

Correct answer:

The graphs of the equations do not intersect.

Explanation:

Substitute $\frac{4}{x}$ for in the second equation:

$x+3\cdot \frac{4}{x} = 4$

$x+ \frac{12}{x} = 4$

$x+ \frac{12}{x}- 4 = 4 - 4$

$x- 4 + \frac{12}{x}= 0$

$\left (x- 4 + \frac{12}{x} \right )\cdot x = 0 \cdot x$

$x^{2}- 4 x + 12 = 0$

The discriminant of this quadratic expression is $b^{2} - 4ac$ , where a=1, b= -4, c=12 ; this is

$b^{2} - 4ac = (-4)^{2}-4 \cdot 1 \cdot 12 = 16 -48 = -32$ .

The discriminant being negative, there are no real solutions to this quadratic equation. Consequently, there are no points of intersection of the graphs of the two equations on the coordinate plane.

Report an Error

View SSAT- Upper Level Tutors

Sundeep
Certified Tutor

Indian Institute of Technology, Engineering in Computer Science, Electrical Engineering. New York University, Masters in Busi...

View SSAT- Upper Level Tutors

Colin
Certified Tutor

Cooper Union for the Advancement of Science and Art, Bachelor of Fine Arts, Fine and Studio Arts Management. The University o...

View SSAT- Upper Level Tutors

Keith
Certified Tutor

Xavier University, Bachelor in Business Administration, Accounting. Ohio University-Main Campus, Masters in Education, Mathem...

All SSAT Upper Level Math Resources

6 Diagnostic Tests 311 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

MCAT Tutors in Dallas Fort Worth, Physics Tutors in Chicago, Computer Science Tutors in Philadelphia, MCAT Tutors in New York City, SAT Tutors in Atlanta, LSAT Tutors in Philadelphia, SSAT Tutors in Atlanta, Computer Science Tutors in Atlanta, ISEE Tutors in Houston, MCAT Tutors in Chicago

Popular Courses & Classes

LSAT Courses & Classes in Washington DC, Spanish Courses & Classes in Denver, ISEE Courses & Classes in Atlanta, SAT Courses & Classes in Dallas Fort Worth, ISEE Courses & Classes in Los Angeles, ISEE Courses & Classes in Denver, SAT Courses & Classes in Los Angeles, SSAT Courses & Classes in Atlanta, ACT Courses & Classes in New York City, LSAT Courses & Classes in San Diego

Popular Test Prep

ACT Test Prep in New York City, SSAT Test Prep in Seattle, SSAT Test Prep in San Diego, ISEE Test Prep in New York City, GRE Test Prep in Dallas Fort Worth, GRE Test Prep in Philadelphia, SAT Test Prep in Denver, SAT Test Prep in Chicago, ISEE Test Prep in Phoenix, MCAT Test Prep in Atlanta

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

SSAT Upper Level Math : SSAT Upper Level Quantitative (Math)

Study concepts, example questions & explanations for SSAT Upper Level Math

All SSAT Upper Level Math Resources

Example Questions

Example Question #631 : Ssat Upper Level Quantitative (Math)

Example Question #94 : Graphing

Example Question #95 : Graphing

Example Question #96 : Graphing

Example Question #21 : How To Graph Complex Numbers

Example Question #1 : How To Graph Inverse Variation

Example Question #1 : How To Graph Inverse Variation

Example Question #3 : How To Graph Inverse Variation

Example Question #1 : How To Graph Inverse Variation

Example Question #5 : How To Graph Inverse Variation

All SSAT Upper Level Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: