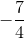This is a two-step problem. First, the slope of the original line needs to be found. The slope will be represented by "" when the line is in -intercept form .

So the slope of the original line is . A line with perpendicular slope will have a slope that is the inverse reciprocal of the original. So in this case, the slope would be . The second step is finding which line will give you that slope. For the correct answer, we find the following:

So, the slope is , and this line is perpendicular to the original.

For lines to be parallel, they must have the same slope. The slope of the line we are looking for then must be .

The point that's given in the equation is also the y-intercept.

Using these two pieces of information, we know that the equation for the line must be 

In this kind of problem, it's important to keep track of information given about your line of interest. In this case, the coordinates given set up the stage for us to be able to get to our line of focus - the line perpendicular to the tangent line. In order to determine the perpendicular line's slope, the tangent line's slope must be calculated. Keeping in mind that:

where y2,x2 and y1,x1 are assigned arbitrarily as long as the order of assignment is maintained. 

  which is the slope of the tangent line.

To calculate the perpendicular line, we have to remember that the product of the tangent slope and the perpendicular slope will equal -1.

, the perpendicular slope can then be calculated as 

Rewrite the linear equation in standard form to slope-intercept form, .

Since the slope of every point of this line is , the slope of the tangent line at the given point should also be .

To determine the slope of the function, , use the power rule to find the derivative function.

The slope at every point of the function  has a slope of .  To find the slope at the given point, substitute the x-value of the given point, , into the derivative function to find the slope.

The line must be perpendicular to the radius at the point (–3,4). The slope of the radius is given by  

The radius has endpoints (–3,4) and the center of the circle (0,0), so its slope is –4/3.

The slope of the tangent line must be perpendicular to the slope of the radius, so the slope of the line is ¾.

The equation of the line is y – 4 = (3/4)(x – (–3))

Rearranging gives us: 3x – 4y = -25

To find the slope of the tangent line, it is necessary to determine the slope of the function.

The function  is already in the slope-intercept form, , and .

Substitute the slope and the given point  into the slope-intercept equation.

Substitute the known slope and the y-intercept to the slope-intercept form.

The graph below is the graph of the absolute value function , which pairs each -coordinate with its absolute value.

The given graph is the same as the above graph, except that each -coordinate is paired with the -coordinate three times that with which it is paired in the above graph. Therefore, the equation graphed is 

or

The graph of a function  shifted right three units is the graph of . In this graph, , so the graph formed by the transformation is

The correct equation is .

The reflection of the graph of the equation  about the origin is the graph of the equation , so replace  with  and  with :

becomes