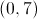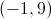Call Now to Set Up Tutoring:

(888) 888-0446

SSAT Upper Level Math : Coordinate Geometry

Study concepts, example questions & explanations for SSAT Upper Level Math

Create An Account

All SSAT Upper Level Math Resources

6 Diagnostic Tests 311 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #3 : How To Graph Inverse Variation

Give the -intercept of the graph of the equation $y = \frac{3}{5x-8}$ .

Possible Answers:

$\left ( 0, -\frac{3}{5}\right )$

The graph has no -intercept.

$\left ( 0, \frac{8}{5}\right )$

$\left ( 0, -\frac{3}{8}\right )$

(0,8)

Correct answer:

$\left ( 0, -\frac{3}{8}\right )$

Explanation:

The -intercept of the graph of an equation is the point at which it intersects the -axis. Its -coordinate is 0, so set x = 0 and solve for :

$y = \frac{3}{5x-8}$

$y = \frac{3}{5 (0)-8}$

$y = \frac{3}{0-8}$

$y =- \frac{3}{8}$

$\left ( 0, -\frac{3}{8}\right )$ is the -intercept.

Report an Error

Example Question #4 : How To Graph Inverse Variation

Give the slope of the line that passes through the - and -intercepts of the graph of the equation $y = \frac{6}{8x-2}$ .

Possible Answers:

The line cannot exist as described.

$-\frac{1}{3}$

$\frac{3}{4}$

$\frac{4}{3}$

Correct answer:

The line cannot exist as described.

Explanation:

The graph of $y = \frac{6}{8x-2}$ does not have an -intercept. If it did, then it would be the point on the graph with -coordinate 0. If we were to make this substitution, the equation would be

$0= \frac{6}{8x-2}$

and

$0 \cdot (8x-2)= \frac{6}{8x-2} \cdot (8x-2)$

0=6

This is identically false, so the graph has no -intercept. Therefore, the line cannot exist as described.

Report an Error

Example Question #5 : How To Graph Inverse Variation

Give the -coordinate of a point with a positive -coordinate at which the graphs of the equations $y = \frac{4}{x}$ and x+3y = 4 intersect.

Possible Answers:

$\frac{2}{3}$

$\frac{4}{3}$

The graphs of the equations do not intersect.

Correct answer:

The graphs of the equations do not intersect.

Explanation:

Substitute $\frac{4}{x}$ for in the second equation:

$x+3\cdot \frac{4}{x} = 4$

$x+ \frac{12}{x} = 4$

$x+ \frac{12}{x}- 4 = 4 - 4$

$x- 4 + \frac{12}{x}= 0$

$\left (x- 4 + \frac{12}{x} \right )\cdot x = 0 \cdot x$

$x^{2}- 4 x + 12 = 0$

The discriminant of this quadratic expression is $b^{2} - 4ac$ , where a=1, b= -4, c=12 ; this is

$b^{2} - 4ac = (-4)^{2}-4 \cdot 1 \cdot 12 = 16 -48 = -32$ .

The discriminant being negative, there are no real solutions to this quadratic equation. Consequently, there are no points of intersection of the graphs of the two equations on the coordinate plane.

Report an Error

Example Question #6 : How To Graph Inverse Variation

The graphs of the equations $y = \frac{3}{x}$ and 4x-2y = -10 intersect in two points; one has a positive -coordinate. and one has a negative -coordinate. Give the -coordinate of the point of intersection that has a positive -coordinate.

Possible Answers:

Correct answer:

Explanation:

Substitute $\frac{3}{x}$ for in the second equation:

4x-2y = -10

$4x-2 \left ( \frac{3}{x} \right )= -10$

$4x- \frac{6}{x} = -10$

$4x- \frac{6}{x} + 10 = -10+ 10$

$4x- \frac{6}{x} + 10 = 0$

$\left (4x- \frac{6}{x} + 10 \right ) \cdot \frac{x}{2} = 0 \cdot \frac{x}{2}$

$2x^{2}-3+5x = 0$

$2x^{2}+5x -3= 0$

This quadratic equation can be solved using the method; the integers with product $2 \cdot (-3) = -6$ and sum 5 are and 6, so continue as follows:

$2x^{2}-x+6x -3= 0$

x (2x -1) +3(2x -1)= 0

(x+3 )(2x -1)= 0

Either x+3 = 0 , in which case x = -3 , or

2x-1 = 0 , in which case

2x = 1

x = 0.5

The desired -coordinate is paired with the positive -coordinate, so we substitute 0.5 for in the first equation:

$y = \frac{3}{x} = \frac{3}{0.5} = 6$

Report an Error

Example Question #5 : How To Graph Inverse Variation

Give the -coordinate of the point at which the graphs of the equations $y = \frac{2}{x}$ and $y = -\frac{3}{x+1}$ intersect.

Possible Answers:

The graphs of the equations do not intersect.

Correct answer:

Explanation:

Using the substitution method, set the values of equal to each other.

$\frac{2}{x} = -\frac{3}{x+1}$

Multiply both sides by $\frac{x(x+1)}{1}$ :

$\frac{2}{x} \cdot \frac{x(x+1)}{1}= -\frac{3}{x+1}\cdot \frac{x(x+1)}{1}$

2(x+1)= -3x

2x+2= -3x

2x+2 + 3x -2 = -3x + 3x -2

5x = -2

$5x \div 5 = -2 \div 5$

x = -0.4

Substitute in either equation:

$y = \frac{2}{x} = \frac{2}{-0.4} = -5$

Report an Error

Example Question #6 : How To Graph Inverse Variation

A line with slope 4 shares its -intercept with that of the graph of the equation $y = \frac{3}{2x-7}$ . Which of the following is the equation of that line?

Possible Answers:

This line does not exist, since the graph of $y = \frac{3}{2x-7}$ has no -intercept.

8x-2y =- 7

28x-7y=2

8x-2y=-3

28x-7y=3

Correct answer:

28x-7y=3

Explanation:

The -intercept of the graph of $y = \frac{3}{2x-7}$ —the point at which it crosses the -axis—is the point at which x = 0 , so substitute accordingly and solve for :

$y = \frac{3}{2 \cdot 0-7}= \frac{3}{ 0-7}=- \frac{3}{ 7}$

The -intercept of this graph, and that of the line, is $\left (0, - \frac{3}{ 7} \right )$ . Since the slope is 4, the slope-intercept form of the equation of the line is

$y = 4x-\frac{3}{7}$

To put it in standard form:

$y-y + \frac{3}{7} = 4x-\frac{3}{7} -y + \frac{3}{7}$

$\frac{3}{7} = 4x -y$

$\frac{3}{7} \cdot 7 = (4x -y) \cdot 7$

28x-7y=3

Report an Error

Example Question #7 : How To Graph Inverse Variation

$\bigtriangleup ABC \cong \bigtriangleup DEF$ , where $\angle B$ is a right angle, $m \angle C = 30 ^{\circ }$ , and AC = 30 . Which of the following cannot be true?

Possible Answers:

All of the statements given in the other choices are true.

$\angle F$ is a right angle

$m \angle D = 60^{\circ }$

$\bigtriangleup DEF$ has perimeter $45+15 \sqrt{3}$

DE= 15

Correct answer:

$\angle F$ is a right angle

Explanation:

$\angle B$ is a right angle and $m \angle C = 30 ^{\circ }$ , so

$m \angle A = 90 ^{\circ } - m \angle C = 90 ^{\circ } - 30 ^{\circ }= 60 ^{\circ }$ ,

making $\bigtriangleup ABC$ a 30-60-90 triangle. By the 30-60-90 Triangle Theorem, the length of the short leg $\overline{AB}$ is half that of hypotenuse $\overline{AC}$ :

$AB= \frac{1}{2}\cdot AC = \frac{1}{2}\cdot 30 = 15$

and the length of long leg $\overline{BC}$ is $\sqrt {3}$ times that of $\overline{AB}$ :

$BC = AB \cdot \sqrt{3}= 15 \cdot \sqrt{3}$

Corresponding sides of congruent triangles are congruent, so DE = AB ; since AB=15 , it follows that DE = 15 .

Also, DE = AB = 15 , $EF= BC = 15 \sqrt{3}$ , and DF = AC = 30 , so the perimeter of $\bigtriangleup DEF$ is the sum of these, or

$DE+EF+DF = 15+15 \sqrt{3}+30=45+15 \sqrt{3}$ .

Corresponding angles are congruent, so $m \angle D = m \angle A$ and $m \angle F = m \angle C$ . By substitution, $m \angle D = 60 ^{\circ }$ and $m \angle F = 30 ^{\circ }$ .

The false statement among the choices is that $\angle F$ is a right angle.

Report an Error

Example Question #3 : How To Graph A Quadratic Function

What is the vertex of the function $f(x)=-2x^{2}-4x+7$ ?

Possible Answers:

(0,7)

(2,-9)

(-2,7)

(-1,9)

(1,1)

Correct answer:

(-1,9)

Explanation:

f(x)=ax^2+bx+c

$f(x)=-2x^{2}-4x+7$

The -coordinate of the vertex is $x=-\frac{b}{2a}$ , where a = -2, b=-4 .

$x=-\frac{b}{2a} = -\frac{-4}{2 \left (-2 \right )} = -1$

To get the -coordinate, evaluate f (-1) .

$f(x)=-2x^{2}-4x+7$

$f(1)=-2 \left (-1 \right )^{2}-4\left (-1 \right )+7 =-2+4+7=9$

The vertex is (-1,9) .

Report an Error

View SSAT- Upper Level Tutors

Carneta
Certified Tutor

Langston University, Bachelor in Arts, Theater Arts.

View SSAT- Upper Level Tutors

Gabby
Certified Tutor

University of Oregon, Bachelor in Arts, Journalism. University of Oregon, Current Grad Student, Communication, General.

View SSAT- Upper Level Tutors

Liban
Certified Tutor

Emory University, Bachelor of Science, Mathematics/Economics.

All SSAT Upper Level Math Resources

6 Diagnostic Tests 311 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

English Tutors in Boston, GMAT Tutors in Miami, Spanish Tutors in Atlanta, Reading Tutors in Chicago, Biology Tutors in San Francisco-Bay Area, MCAT Tutors in Philadelphia, English Tutors in Washington DC, GRE Tutors in Washington DC, Reading Tutors in San Francisco-Bay Area, Spanish Tutors in Miami

Popular Courses & Classes

SAT Courses & Classes in Philadelphia, ACT Courses & Classes in Seattle, GRE Courses & Classes in Washington DC, ACT Courses & Classes in Houston, ACT Courses & Classes in Dallas Fort Worth, ACT Courses & Classes in Denver, LSAT Courses & Classes in Boston, ACT Courses & Classes in San Diego, Spanish Courses & Classes in Philadelphia, SAT Courses & Classes in Los Angeles

Popular Test Prep

SAT Test Prep in Philadelphia, GRE Test Prep in Washington DC, ACT Test Prep in Houston, ACT Test Prep in Los Angeles, ACT Test Prep in San Diego, ACT Test Prep in Denver, GRE Test Prep in Chicago, LSAT Test Prep in San Francisco-Bay Area, ACT Test Prep in Washington DC, ACT Test Prep in Seattle

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

SSAT Upper Level Math : Coordinate Geometry

Study concepts, example questions & explanations for SSAT Upper Level Math

All SSAT Upper Level Math Resources

Example Questions

Example Question #3 : How To Graph Inverse Variation

Example Question #4 : How To Graph Inverse Variation

Example Question #5 : How To Graph Inverse Variation

Example Question #6 : How To Graph Inverse Variation

Example Question #5 : How To Graph Inverse Variation

Example Question #6 : How To Graph Inverse Variation

Example Question #7 : How To Graph Inverse Variation

Example Question #3 : How To Graph A Quadratic Function

All SSAT Upper Level Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: