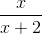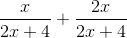We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

SAT Math : SAT Mathematics

Study concepts, example questions & explanations for SAT Math

Create An Account

All SAT Math Resources

16 Diagnostic Tests 660 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #685 : Algebra

$3 ^{x}= 9 ^{y+1}$ .

Express in terms of .

Possible Answers:

y = 2x-1

$y = \frac{1}{2}x- 1$

$y = x- \frac{1}{2}$

$y = \frac{1}{2}x- \frac{1}{2}$

y = 2x-2

Correct answer:

$y = \frac{1}{2}x- 1$

Explanation:

$3 ^{x}= 9 ^{y+1}$

$3 ^{x}= (3 ^{2} )^{y+1}$

$3 ^{x}= 3 ^{2(y+1)} = 3 ^{2y+2}$

x = 2y + 2

2y = x-2

$y = \frac{x-2}{2} = \frac{1}{2}x- 1$

Report an Error

Example Question #686 : Algebra

$10,000^{3x+7} = 10 ^{y- 2}$

Express in terms of .

Possible Answers:

y = 3x+9

y = 12x+ 30

$y = \frac{1}{12}x+ 26$

y = 12x+ 36

$y = \frac{1}{12}x+ 30$

Correct answer:

y = 12x+ 30

Explanation:

$10,000^{3x+7} = 10 ^{y- 2}$

$\left ( 10 ^{4} \right )^{3x+7} = 10 ^{y- 2}$

$10 ^{4 \left ( 3x+7 \right )} = 10 ^{y- 2}$

$10 ^{12x+28} = 10 ^{y- 2}$

y - 2 = 12x + 28

y = 12x + 28+ 2 =12x+ 30

Report an Error

Example Question #25 : Pattern Behaviors In Exponents

$16^{5x-17} = 8^{2y + 1}$

Express in terms of .

Possible Answers:

y = 10x-71

$y = \frac{10}{3}x- \frac{65}{6}$

y = 10x-65

$y = \frac{10}{3}x- 10$

$y = \frac{10}{3}x- \frac{71}{6}$

Correct answer:

$y = \frac{10}{3}x- \frac{71}{6}$

Explanation:

$16^{5x-17} = 8^{2y + 1}$

$\left (2^{4} \right )^{5x-17} =( 2 ^{3})^{2y + 1}$

$2^{4(5x-17) }= 2 ^{3(2y + 1)}$

$2^{20x-68}= 2 ^{6y + 3}$

6y+3 = 20x-68

6y+3 -3= 20x-68 - 3

6y = 20x-71

$\frac{6y}{6} = \frac{20x-71}{6}$

$y = \frac{10}{3}x- \frac{71}{6}$

Report an Error

Example Question #688 : Algebra

$5^{x} \cdot 25 ^{y} = 5 ^{y} \cdot 125 ^{x}$

Express in terms of .

Possible Answers:

y = x+ 1

y = x+2

y = 3x

y = 2x

y =x+ 3

Correct answer:

y = 2x

Explanation:

To solve this problem, first convert all numbers to have the like base of five.

$5^{x} \cdot 25 ^{y} = 5 ^{y} \cdot 125 ^{x}$

$5^{x} \cdot\left ( 5 ^{2} \right ) ^{y} = 5 ^{y} \cdot\left ( 5 ^{3} \right ) ^{x}$

$5^{x} \cdot 5 ^{2y} = 5 ^{y} \cdot 5 ^{3x}$

Recall the rule of exponents which states, when multiplying like bases we add their exponents.

$5^{x+2y} = 5 ^{3x+ y}$

Since we have the same base we can set the exponents equal to each other and solve.

x+2y = 3x+y

x+2y - x-y = 3x+y - x-y

y = 2x

Report an Error

Example Question #689 : Algebra

Solve for :

$4^{3t+ 8} = 8 ^{2t- 11}$

Possible Answers:

t = 3

t = 19

t = 6

$t = \frac{9}{2}$

This statement has no solution.

Correct answer:

This statement has no solution.

Explanation:

To solve this problem, first convert all numbers to have the like base of two

$4^{3t+ 8} = 8 ^{2t- 11}$

$\left (2^{2} \right )^{3t+ 8} = \left (2^{3} \right ) ^{2t- 11}$

Recall that when exponents are raised to another power the exponents are multiplied together.

$2^{2(3t+ 8)} = 2^{3(2t- 11)}$

Since we have the same base we can set the exponents equal to each other and solve.

2(3t+ 8) =3(2t- 11)

6t+16 =6t-33

6t+16 - 6t =6t-33 - 6t

16 = -33

This statement is identically false, so the original statement has no solution.

Report an Error

Example Question #21 : How To Find Patterns In Exponents

Solve for :

$3^{4y-2 } = 27 ^{y}$

Possible Answers:

The equation has no solution.

$y =- \frac{1}{2}$

y = -2

$y = \frac{1}{2}$

y = 2

Correct answer:

y = 2

Explanation:

To solve this problem, first convert all numbers to have the like base of three.

$3^{4y-2 } = 27 ^{y}$

$3^{4y-2 } = \left ( 3^{3} \right ) ^{y}$

$3^{4y-2 } = 3^{3y}$

Since we have the same base we can set the exponents equal to each other and solve.

3y= 4y-2

3y - 3y + 2 = 4y-2 - 3y + 2

y = 2

Report an Error

Example Question #2461 : Sat Mathematics

What is the unit's digit of $2^{357}$ ?

Possible Answers:

Correct answer:

Explanation:

Notice that there is a repeating pattern in the unit's digit of the powers of 2:

2^1=2

2^2=4

2^3=8

2^4=16

2^5=32

2^6=64

and so on. The pattern is four numbers long, which means we need to count how many complete blocks of 4 numbers are in the 357-number pattern. That is,

$357\div4=89$ with a remainder of 1.

The remainder is all we need, since it tells us where in the pattern we need to be: the first place, with a unit's digit of 2.

Report an Error

Example Question #2461 : Sat Mathematics

Simplify the expression.

$\frac{x}{2x+4}+\frac{x}{x+2}$

Possible Answers:

$\frac{2x}{3x+6}$

$\frac{1}{2x+4}$

$\frac{x+1}{2x+4}$

$\frac{3x}{2x+4}$

$\frac{x}{x+2}$

Correct answer:

$\frac{3x}{2x+4}$

Explanation:

To add rational expressions, first find the least common denominator. Because the denominator of the first fraction factors to 2(x+2), it is clear that this is the common denominator. Therefore, multiply the numerator and denominator of the second fraction by 2.

$\frac{x}{2x+4}+\frac{x}{x+2}$

$\frac{x}{2x+4}+\frac{(2)x}{(2)(x+2)}$

$\frac{x}{2x+4}+\frac{2x}{2x+4}$

$\frac{3x}{2x+4}$

This is the most simplified version of the rational expression.

Report an Error

Example Question #2 : Expressions

Simplify the following:

$\frac{x+3}{x-2}+\frac{x-2}{x+3}$

Possible Answers:

2x^2-4x+4

$\frac{2(x+3)(x-2)}{(x+3)(x-2)}$

$\frac{(x+3)^2+(x-2)^2}{(x+3)(x-2)}$

Correct answer:

$\frac{(x+3)^2+(x-2)^2}{(x+3)(x-2)}$

Explanation:

To simplify the following, a common denominator must be achieved. In this case, the first term must be multiplied by (x+2) in both the numerator and denominator and likewise with the second term with (x-3).

$\frac{(x+3)(x+3)}{(x-2)(x+3)}+\frac{(x-2)(x-2)}{(x+3)(x-2)}$

$\frac{(x+3)^2+(x-2)^2}{(x+3)(x-2)}$

Report an Error

Example Question #2462 : Sat Mathematics

If √(ab) = 8, and a²= b, what is a?

Possible Answers:

Correct answer:

Explanation:

If we plug in a² for b in the radical expression, we get √(a³) = 8. This can be rewritten as a^3/2 = 8. Thus, log_a8 = 3/2. Plugging in the answer choices gives 4 as the correct answer.

Report an Error

View SAT Mathematics Tutors

Paulkey
Certified Tutor

National Chiao Tung University, Bachelors, Computer Science & Information Engineering. University of Maryland Eastern Shore, ...

View SAT Mathematics Tutors

Sarah
Certified Tutor

New York University, Bachelor of Fine Arts, Drama.

View SAT Mathematics Tutors

Thomas
Certified Tutor

Michigan State University, Bachelor in Arts, History. Central Michigan University, Doctor of Philosophy, History.

All SAT Math Resources

16 Diagnostic Tests 660 Practice Tests Question of the Day Flashcards Learn by Concept

SAT Math Tutors in Top Cities:

Atlanta SAT Math Tutors, Austin SAT Math Tutors, Boston SAT Math Tutors, Chicago SAT Math Tutors, Dallas Fort Worth SAT Math Tutors, Denver SAT Math Tutors, Houston SAT Math Tutors, Kansas City SAT Math Tutors, Los Angeles SAT Math Tutors, Miami SAT Math Tutors, New York City SAT Math Tutors, Philadelphia SAT Math Tutors, Phoenix SAT Math Tutors, San Diego SAT Math Tutors, San Francisco-Bay Area SAT Math Tutors, Seattle SAT Math Tutors, St. Louis SAT Math Tutors, Tucson SAT Math Tutors, Washington DC SAT Math Tutors

Popular Courses & Classes

SSAT Courses & Classes in Denver, MCAT Courses & Classes in Chicago, SAT Courses & Classes in Houston, LSAT Courses & Classes in Boston, ACT Courses & Classes in Washington DC, LSAT Courses & Classes in San Francisco-Bay Area, ACT Courses & Classes in Atlanta, LSAT Courses & Classes in Seattle, SSAT Courses & Classes in Chicago, ACT Courses & Classes in Philadelphia

Popular Test Prep

SSAT Test Prep in Miami, SSAT Test Prep in Los Angeles, ISEE Test Prep in Philadelphia, SAT Test Prep in Los Angeles, ACT Test Prep in Atlanta, MCAT Test Prep in New York City, ACT Test Prep in Seattle, GRE Test Prep in Boston, MCAT Test Prep in Los Angeles, GMAT Test Prep in Los Angeles

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

SAT Math : SAT Mathematics

Study concepts, example questions & explanations for SAT Math

All SAT Math Resources

Example Questions

Example Question #685 : Algebra

Example Question #686 : Algebra

Example Question #25 : Pattern Behaviors In Exponents

Example Question #688 : Algebra

Example Question #689 : Algebra

Example Question #21 : How To Find Patterns In Exponents

Example Question #2461 : Sat Mathematics

Example Question #2461 : Sat Mathematics

Example Question #2 : Expressions

Example Question #2462 : Sat Mathematics

All SAT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: