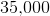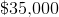All PSAT Math Resources
Example Questions
Example Question #1 : Drawing Conclusions From Graphs & Tables
Matt conducted a statistical experiment to determine the relationship between yearly salary earned and age. In this study, he assigned age (in years) as the independent variable, and yearly salary as the dependent variable. He drew a line of best fit and found a slope of 
Each month, a person's salary decreases by
Each year, a person's salary decreases by
Each year, a person's salary increases by
Each month, a person's salary increases by
Each year, a person's salary increases by
The slope of a line is the rate that a line increases or decreases. The question tells us that Matt looked at the relationship between age, in years; thus, the correct answer should include "each year", which eliminates the answer choices that include "each month". Finally the slope is 


Example Question #1 : Drawing Conclusions From Graphs & Tables
Mrs. Frame conducted a statistical experiment to determine the relationship between test grades and the number of hours her students spent studying. In this study, she assigned the number of hours spent studying as the independent variable, and the test grades (in percentages) were assigned as the dependent variable. She plotted the data on a scatter plot and drew a line of best fit. If the slope of the best fit line was 



Yes ,the 
No, the 
Yes, the 
Yes ,the 
Yes ,the 
The equation of the best fit line will be in slope intercept form:
The question tells us that the slope is 

We had to convert our percentage into a decimal in order to multiple, but we need to change it back to a percent before we subtract so that we arrive at the correct answer because 

Example Question #2 : Drawing Conclusions From Graphs & Tables
Mr. Miller conducted a statistical experiment to determine the relationship between final grades and the number of school days that his students missed. In this study, he assigned the number of missed school days as the independent variable, and the final grade was assigned as the dependent variable. He plotted his results on a scatter plot. If the results follow a linear relationship, what is a reasonable conclusion that could be found based these results?
A negative slope
A slope of
A positive slope
An undefined slope
A negative slope
We know, from attending school ourselves, that every day we learn something new in school. When a day of school is missed, there is a lot of catch up that needs to be done, but the teacher's instructions and lesson given each day can't be repeated the day you return, because the teacher has to move on with the rest of the class. If you missed a week of school, that's five days of lessons that were missed. Wouldn't it be challenging to catch up on what was missed, as well as learning what the teacher is currently teaching when you return? This would likely be challenging; thus, we can conclude that the more days of school missed, the lower a students final grade will be. As the number of days missed increases, the final grade will decrease; thus, the best fit line will have a negative slope.
Example Question #31 : Statistics & Probability
Matt conducted a statistical experiment to determine the relationship between yearly salary earned and age. In this study, he assigned age (in years) as the independent variable, and yearly salary as the dependent variable. He plotted his results on a scatter plot. If the results follow a linear relationship, what is a reasonable conclusion that could be found based these results?
A slope of
An undefined slope
A positive slope
A negative slope
A positive slope
To help us answer this question, let's think about what we know about jobs:
If you were to get a part time job when you turn 

Example Question #1 : Drawing Conclusions From Graphs & Tables
A school principal conducted a statistical experiment to determine the relationship between 

A positive slope
A negative slope
An undefined slope
A slope of
A positive slope
We know, from attending school ourselves, that completing homework assignments and studying for quizzes and tests means that we will do better in school than if we didn't do those things. Completing homework and studying for tests takes time outside of school. Normally, the harder you study and the more time you spend studying, the more likely you are to do well in school. If you don't study at all, nor spend anytime completing homework assignments, your school grade will likely be lower than if you had spent time preparing and completing assignments; thus, we can conclude that the more time we spend studying, the higher our 

Example Question #551 : Grade 8
Mrs. Frame conducted a statistical experiment to determine the relationship between test grades and the number of hours her students spent studying. In this study, she assigned the number of hours spent studying as the independent variable, and the test grades (in percentages) were assigned as the dependent variable. She plotted her results on a scatter plot. If the results follow a linear relationship, what is a reasonable conclusion that could be found based these results?
A negative slope
An undefined slope
A slope of
A positive slope
A positive slope
We know, from attending school ourselves, that when we have a test coming up that we want to do well on, we'll study for the test. Normally, the harder you study and the more time you spend studying, the more likely you are to do well on the test. If you don't study at all, and don't know the material that's being covered on a test, you'll like do poorly on a test; thus, we can conclude that the more time we spend studying, the higher our test grade will be. As the number of hours studied increases, the score on the test will increase; thus, the best fit line will have a positive slope.
Example Question #3 : Drawing Conclusions From Graphs & Tables
A doctor conducted a statistical experiment to determine the relationship between age and height. In this study, she assigned age as the independent variable, and height (in inches) as the dependent variable. She plotted the data on a scatter plot. The doctor drew a line of best fit and found the 

The average person has a height of
Every month a person grows by
Every year a person grows by
A newborn baby will have an average height of
A newborn baby will have an average height of
The question tells us that age is the independent variable, or the 
The 


Example Question #1 : Use The Equation Of A Linear Model To Solve Problems: Ccss.Math.Content.8.Sp.A.3
A used car dealership conducted a statistical experiment to determine the relationship between the age of a car and the cost. In this study, he assigned age as the independent variable, and price as the dependent variable. He plotted the data on a scatter plot and drew a line of best fit. The 

The slope for the best fit line of this data set is
The price of a brand new car is
AS a car ages, the price decreases by
As a car ages, the price increases by
The price of a brand new car is
The question tells us that age is the independent variable, or the 
The 


Example Question #7 : Use The Equation Of A Linear Model To Solve Problems: Ccss.Math.Content.8.Sp.A.3
A used car dealership conducted a statistical experiment to determine the relationship between the age of a car and the cost. In this study, he assigned age as the independent variable, and price as the dependent variable. He plotted the data on a scatter plot and drew a line of best fit. If the results follow a linear relationship, what is a reasonable conclusion that could be found based these results?
A positive slope
An undefined slope
A negative slope
A slope of
A negative slope
Imagine that you were buying a car. You have two options: a brand new car for 

Example Question #32 : Statistics & Probability
A doctor conducted a statistical experiment to determine the relationship between weight and height. In this study, she assigned weight as the independent variable, and height (in inches) as the dependent variable. She plotted the data on a scatter plot. If the results follow a linear relationship, what is a reasonable conclusion that could be found based these results?
A negative slope
An undefined slope
A positive slope
A slope of
A positive slope
Let's think about ourselves in this scenario, as you've gotten taller, has your weight increased or decreased? Most likely, as your height has increased your weight has also increased; thus the slope of the best fit line for this data would be positive.
Certified Tutor
All PSAT Math Resources