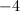We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

PSAT Math : Equations / Inequalities

Study concepts, example questions & explanations for PSAT Math

Create An Account

All PSAT Math Resources

10 Diagnostic Tests 421 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

← Previous 1 2 3 4 5 6 7 8 9 10 11 12 … 22 23 Next →

Example Question #3 : How To Find The Solution To An Inequality With Division

Solve for :

7x+12>3x-13

Possible Answers:

$x>-\frac{35}{8}$

$x<-\frac{1}{4}$

$x>-\frac{25}{4}$

$x>-\frac{12}{25}$

$x<-\frac{25}{4}$

Correct answer:

$x>-\frac{25}{4}$

Explanation:

Begin by moving all of the values to the left side of the inequality:

7x+12>3x-13

becomes

4x+12>-13

Next, move the to the right side:

4x>-25

Finally, divide both sides by :

$x>-\frac{25}{4}$

Report an Error

Example Question #1 : How To Find The Solution To An Inequality With Division

Solve for :

4x-23<7x+5

Possible Answers:

$x<\frac{18}{3}$

$x>\frac{18}{3}$

$x>-\frac{28}{3}$

$x<-\frac{28}{3}$

$x<\frac{31}{4}$

Correct answer:

$x>-\frac{28}{3}$

Explanation:

First, move the values to the left side of the inequality:

4x-23<7x+5

becomes

-3x-23<5

Next, move the to the right side:

-3x<28

Finally, divide by . Remember: you must flip the inequality sign when you multiply or divide by a negative number.

$x>-\frac{28}{3}$

Report an Error

Example Question #5 : How To Find The Solution To An Inequality With Division

Solve for :

17x +122>20x-22

Possible Answers:

$x < \frac{137}{3}$

x < 48

$x > \frac{130}{4}$

x < 92

x > 48

Correct answer:

x < 48

Explanation:

First, get the factors on the left side of the inequality:

17x +122>20x-22

becomes

-3x +122>-22

Next, subtract 122 from both sides:

-3x>-144

Now, divide by . Remember: Dividing or multiplying by a negative number requires you to flip the inequality sign:

x < 48

Report an Error

Example Question #1 : How To Find The Solution To An Inequality With Division

Solve the inequality

$\frac{3x}{5} > 6$

Possible Answers:

x < 10

x > 9

x > 10

x > 3

Correct answer:

x > 10

Explanation:

First, multiplying each side of the equality by gives 3x > 30 . Next, dividing each side of the inequality by will solve for ; x > 10 .

Report an Error

Example Question #7 : Inequalities

What is the solution set of the inequality $\dpi{100} \small 3x+8<35$ ?

Possible Answers:

$\dpi{100} \small x<35$

$\dpi{100} \small x<27$

$\dpi{100} \small x<9$

$\dpi{100} \small x>27$

$\dpi{100} \small x>9$

Correct answer:

$\dpi{100} \small x<9$

Explanation:

We simplify this inequality similarly to how we would simplify an equation

$\dpi{100} \small 3x+8-8<35-8$

$\dpi{100} \small \frac{3x}{3}<\frac{27}{3}$

Thus $\dpi{100} \small x<9$

Report an Error

Example Question #8 : Inequalities

What is a solution set of the inequality 2x+12>42 ?

Possible Answers:

x>4

x<15

$x>\frac{3}{2}$

x<9

x>15

Correct answer:

x>15

Explanation:

In order to find the solution set, we solve 2x+12>42 as we would an equation:

2x+12>42

2x>30

x>15

Therefore, the solution set is any value of x>15 .

Report an Error

Example Question #3 : How To Find Out When An Equation Has No Solution

Find the solution to the following equation if x = 3:

y = (4x² - 2)/(9 - x²)

Possible Answers:

no possible solution

Correct answer:

no possible solution

Explanation:

Substituting 3 in for x, you will get 0 in the denominator of the fraction. It is not possible to have 0 be the denominator for a fraction so there is no possible solution to this equation.

Report an Error

Example Question #1 : How To Find Out When An Equation Has No Solution

Undefined_denom3

I. x = 0

II. x = –1

III. x = 1

Possible Answers:

I only

III only

I, II, and III

II and III only

II only

Correct answer:

I only

Explanation:

Undefined_denom2

Report an Error

Example Question #1 : Linear / Rational / Variable Equations

Nosol1

Possible Answers:

–3

–1/2

There is no solution

Correct answer:

There is no solution

Explanation:

Nosol2

Report an Error

Example Question #6 : How To Find Out When An Equation Has No Solution

$\small h\left ( x\right )=\frac{28}{x+4}$

$\small \textup{For which of the following values of}\,x\,\textup{is the above function undefined?}$

Possible Answers:

None of the other answers

$\small 4$

$\small 0$

$\small 28$

$\small -4$

Correct answer:

$\small -4$

Explanation:

A fraction is considered undefined when the denominator equals 0. Set the denominator equal to zero and solve for the variable.

$\small x+4=0$

$\small x=-4$

Report an Error

← Previous 1 2 3 4 5 6 7 8 9 10 11 12 … 22 23 Next →

View PSAT Mathematics Tutors

Eklavya
Certified Tutor

Indira Gandhi National Open University, Bachelor, Physics.

View PSAT Mathematics Tutors

Becca
Certified Tutor

Brigham Young University-Provo, Bachelor of Science, Electrical Engineering.

View PSAT Mathematics Tutors

Antonia
Certified Tutor

University of Michigan-Ann Arbor, Bachelor of Engineering, Nuclear Engineering. University of Wisconsin-Madison, Current Grad...

All PSAT Math Resources

10 Diagnostic Tests 421 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Statistics Tutors in Houston, Statistics Tutors in San Francisco-Bay Area, English Tutors in Boston, MCAT Tutors in Dallas Fort Worth, LSAT Tutors in Los Angeles, Reading Tutors in San Francisco-Bay Area, GRE Tutors in Dallas Fort Worth, Statistics Tutors in Miami, GMAT Tutors in Dallas Fort Worth, Statistics Tutors in San Diego

Popular Courses & Classes

GMAT Courses & Classes in Dallas Fort Worth, MCAT Courses & Classes in Boston, ACT Courses & Classes in Dallas Fort Worth, MCAT Courses & Classes in Dallas Fort Worth, MCAT Courses & Classes in Phoenix, SSAT Courses & Classes in Dallas Fort Worth, SSAT Courses & Classes in Phoenix, SAT Courses & Classes in San Diego, GRE Courses & Classes in Miami, GRE Courses & Classes in Denver

Popular Test Prep

LSAT Test Prep in Washington DC, MCAT Test Prep in Denver, ACT Test Prep in Dallas Fort Worth, ISEE Test Prep in San Diego, ISEE Test Prep in Los Angeles, SAT Test Prep in Miami, SAT Test Prep in Chicago, GMAT Test Prep in Dallas Fort Worth, LSAT Test Prep in Seattle, GMAT Test Prep in Boston

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

PSAT Math : Equations / Inequalities

Study concepts, example questions & explanations for PSAT Math

All PSAT Math Resources

Example Questions

Example Question #3 : How To Find The Solution To An Inequality With Division

Example Question #1 : How To Find The Solution To An Inequality With Division

Example Question #5 : How To Find The Solution To An Inequality With Division

Example Question #1 : How To Find The Solution To An Inequality With Division

Example Question #7 : Inequalities

Example Question #8 : Inequalities

Example Question #3 : How To Find Out When An Equation Has No Solution

Example Question #1 : How To Find Out When An Equation Has No Solution

Example Question #1 : Linear / Rational / Variable Equations

Example Question #6 : How To Find Out When An Equation Has No Solution

All PSAT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: