All Precalculus Resources
Example Questions
Example Question #1 : Graphing Tangent, Cosecant, Secant, And Cotangent Functions
Given the function 

For the function 
Recall that the parent function 


Set the inner quantity of 
This indicates that there is a zero at 



Example Question #2 : Find The Equations Of Vertical Asymptotes Of Tangent, Cosecant, Secant, And Cotangent Functions
Which of the choices represents asymptote(s), if any?
Factor the numerator and denominator.
Notice that the 

The denominator cannot be equal to zero. Set the denominator to find the location where the x-variable cannot exist.
The asymptote is located at 
Example Question #11 : Understanding Asymptotes
Find the vertical asymptote of the equation.
There are no vertical asymptotes.
To find the vertical asymptotes, we set the denominator of the function equal to zero and solve.
Example Question #1 : Find The Equations Of Vertical Asymptotes Of Tangent, Cosecant, Secant, And Cotangent Functions
Given the function 

Example Question #5 : Find The Equations Of Vertical Asymptotes Of Tangent, Cosecant, Secant, And Cotangent Functions
Given the function 

Example Question #6 : Find The Equations Of Vertical Asymptotes Of Tangent, Cosecant, Secant, And Cotangent Functions
Which of the following represents the asymptotes for the general parent function 
If you do not have these asymptotes memorized, they can be easily derived. Write 

Now we need to solve for 





Example Question #1 : Find The Equations Of Vertical Asymptotes Of Tangent, Cosecant, Secant, And Cotangent Functions
Assume that there is a vertical asymptote for the function 



We know that the parent function 


Now we will add this to the parent function equation for vertical asymptotes
Now we will set this equation for the given vertical asymptote at
Example Question #8 : Find The Equations Of Vertical Asymptotes Of Tangent, Cosecant, Secant, And Cotangent Functions
True or false: There is a vertical asymptote for 
The statement is true.
The statement is false.
There is insufficient information to answer the question.
The statement is true.
We know that the parent function of 






So when 

Example Question #891 : Pre Calculus
If the graph 
Do not confuse the movement of an equation of a line with the movement of a point. Moving 

Example Question #2 : Transformations
Which of the following expressions corresponds to a vertical translation of f(x)
Which of the following expressions corresponds to a vertical translation of f(x)
In my experience, vertical translations are the easiest way of changing a graph. A vertical translation will be of the form:
What this basically means is that we simply add our vertical translation onto the end of our function. This will simply make our function +c higher. In this case we need a vertical translation of positive 5. Do the following:
So our answer is:
Certified Tutor
Certified Tutor
All Precalculus Resources




















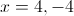











 has vertical asymptotes at
has vertical asymptotes at  where
where  is any integer. We will set the quantity inside the
is any integer. We will set the quantity inside the  function equal to zero to solve for the shift of the asymptote.
function equal to zero to solve for the shift of the asymptote.







 has asymptotes at
has asymptotes at  where
where  is any integer. We will set the quantity within the
is any integer. We will set the quantity within the  function equal to zero in order to find the shift of the asymptote.
function equal to zero in order to find the shift of the asymptote. 








































