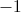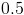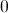Call Now to Set Up Tutoring:

(888) 888-0446

Precalculus : Pre-Calculus

Study concepts, example questions & explanations for Precalculus

Create An Account

All Precalculus Resources

12 Diagnostic Tests 380 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Conic Sections

Using the information below, determine the equation of the hyperbola.

Foci: (-5,4) and (7,4)

Eccentricity: $\frac{3}{2}$

Possible Answers:

$\small \small \small \small \small \frac{(x-4)^{2}}{20} - \frac{(y-1)^{2}}{36}=1$

$\small \small \small \small \frac{(x-1)^{2}}{16} - \frac{(y-4)^{2}}{36}=1$

$\small \small \small \frac{(x-4)^{2}}{16} - \frac{(y-1)^{2}}{20}=1$

$\small \small \frac{(x-1)^{2}}{16} - \frac{(y-4)^{2}}{20}=1$

$\small \small \small \frac{(x-1)^{2}}{20} - \frac{(y-4)^{2}}{16}=1$

Correct answer:

$\small \small \frac{(x-1)^{2}}{16} - \frac{(y-4)^{2}}{20}=1$

Explanation:

General Information for Hyperbola:

Equation for horizontal transverse hyperbola:

$\small \frac{(x-h)^{2}}{a^{2}} - \frac{(y-k)^{2}}{b^{2}}=1$

Distance between foci =

Distance between vertices =

Eccentricity = c/a

$\small a^{2}+b^{2}=c^{2}$

Center: (h, k)

First determine the value of c. Since we know the distance between the two foci is 12, we can set that equal to .

12=2c

$\small c= 6$

Next, use the eccentricity equation and the value of the eccentricity provided in the question to determine the value of a.

Eccentricity = $\small \small c/a=3/2$

$\small \frac{c}{a}=\frac{3}{2}=\frac{6}{a}$

$\small 12=3a$

$\small a=4$

$\small \small a^{2}=16$

Determine the value of $\small b^{2}$

$\small a^{2}+b^{2}=c^{2}$

$\small \small 4^{2}+b^{2}=6^{2}$

$\small \small b^{2}=20$

Determine the center point to identify the values of h and k. Since the y coordinate of the foci are 4, the center point will be on the same line. Hence, $\small k = 4$ .

Since center point is equal distance from both foci, and we know that the distance between the foci is 12, we can conclude that $\small h=1$

Center point: $\small \small (h, k)= (1,4)$

Thus, the equation of the hyperbola is:

$\small \small \frac{(x-1)^{2}}{16} - \frac{(y-4)^{2}}{20}=1$

Report an Error

Example Question #2 : Conic Sections

Using the information below, determine the equation of the hyperbola.

Foci: (1, 8) and (9, 8)

Eccentricity:

Possible Answers:

$\small \small \small \small \small \small \small \small \frac{(x-8)^{2}}{12} - \frac{(y-5)^{2}}{16}=1$

$\small \small \small \small \small \small \small \frac{(x-5)^{2}}{4} - \frac{(y-8)^{2}}{16}=1$

$\small \small \small \small \small \small \small \frac{(x-8)^{2}}{4} - \frac{(y-5)^{2}}{12}=1$

$\small \small \small \small \small \small \small \frac{(x-5)^{2}}{12} - \frac{(y-8)^{2}}{4}=1$

$\small \small \small \small \small \small \frac{(x-5)^{2}}{4} - \frac{(y-8)^{2}}{12}=1$

Correct answer:

$\small \small \small \small \small \small \frac{(x-5)^{2}}{4} - \frac{(y-8)^{2}}{12}=1$

Explanation:

General Information for Hyperbola:

Equation for horizontal transverse hyperbola:

$\small \frac{(x-h)^{2}}{a^{2}} - \frac{(y-k)^{2}}{b^{2}}=1$

Distance between foci =

Distance between vertices =

Eccentricity = c/a

$\small a^{2}+b^{2}=c^{2}$

Center: (h, k)

First determine the value of c. Since we know the distance between the two foci is 8, we can set that equal to .

$\small 8=2c$

$\small \small c= 4$

Next, use the eccentricity equation and the value of the eccentricity provided in the question to determine the value of a.

Eccentricity = $\small \small \small \small c/a=2$

$\small \small \frac{c}{a}=\frac{2}{1}=\frac{4}{a}$

$\small \small 4=2a$

$\small \small a=2$

$\small \small \small a^{2}=4$

Determine the value of $\small b^{2}$

$\small a^{2}+b^{2}=c^{2}$

$\small \small \small 2^{2}+b^{2}=4^{2}$

$\small \small \small b^{2}=12$

Determine the center point to identify the values of h and k. Since the y coordinate of the foci are 8, the center point will be on the same line. Hence, $\small \small k = 8$ .

Since center point is equal distance from both foci, and we know that the distance between the foci is 8, we can conclude that $\small \small h=5$

Center point: $\small \small \small (h, k)= (5, 8 )$

Thus, the equation of the hyperbola is:

$\small \small \small \small \small \small \frac{(x-5)^{2}}{4} - \frac{(y-8)^{2}}{12}=1$

Report an Error

Example Question #2 : Pre Calculus

The function f(x) is such that

f'(0) = 0

When you take the second derivative of the function f(x) , you obtain

f''(x) = x^2

What can you conclude about the function at x=0 ?

Possible Answers:

The point is an absolute maximum.

The point is a local maximum.

The point is an inflection point.

The point is an absolute minimum.

The point is a local minimum.

Correct answer:

The point is an inflection point.

Explanation:

We have a point at which $f^{'}(x)=0$ . We know from the second derivative test that if the second derivative is negative, the function has a maximum at that point. If the second derivative is positive, the function has a minimum at that point. If the second derivative is zero, the function has an inflection point at that point.

Plug in 0 into the second derivative to obtain

f''(0)=0

So the point is an inflection point.

Report an Error

Example Question #1 : Pre Calculus

Consider the function

f(x)=x^2-x

Find the maximum of the function on the interval $\left [ 0,1 \right ]$ .

Possible Answers:

-0.5

0.5

Correct answer:

Explanation:

Notice that on the interval $\left [ 0,1 \right ]$ , the term $\ x^{2}$ is always less than or equal to . So the function is largest at the points when x^2=x . This occurs at x=0 and x=1 .

Plugging in either 1 or 0 into the original function f(x) yields the correct answer of 0.

Report an Error

Example Question #1 : Introductory Calculus

In what -intervals are the relative minimum and relative maximum for the function below?

f(x)=x^3-3x^2-4x

Possible Answers:

$\textup{There are no relative extrema}$

$\textup{Relative minimum in}\ \left ( -1,0\right )\textup{and a relative maximum in}\ \left ( 0,4\right )$

$\textup{Relative maximum in}\ \left ( -4,0\right )\textup{and a relative minimum in}\ \left ( 0,1\right )$

$\textup{Relative maximum in}\ \left ( -1,0\right )\textup{and a relative minimum in}\ \left ( 0,4\right )$

$\textup{Relative minimum in}\ \left ( -4,0\right )\textup{and a relative maximum in}\ \left ( 0,1\right )$

Correct answer:

$\textup{Relative maximum in}\ \left ( -1,0\right )\textup{and a relative minimum in}\ \left ( 0,4\right )$

Explanation:

A cubic function will have at most one relative minimum and one relative maximum. We can determine the zeros be factoring at x=-1,0,4 . From then we only need to determine if the graph is positive or negative in-between the zeros.

The graph is positive between and (plug in x=-0.5 ) and negative between 0 and 4 (plug in x=1 ). This can also be seen from the graph.

Wolframalpha--graph_of_y__x3-3x2-4x_from_x__-5_to_x__5_y__-15_to_y15--2014-12-19_0045

Report an Error

Example Question #1 : Derivatives

What is the minimum of the function f(x)=x^2-10x+26 ?

Possible Answers:

(7,3)

(5,1)

(4,0)

(6,2)

Correct answer:

(5,1)

Explanation:

The vertex form of a parabola is:

f(x)=a(x-h)^2+k

where {}(h,k) is the vertex of the parabola.

The function for this problem can be simplified into vetex form of a parabola:

f(x)=(x-5)^2+1 ,

with a vertex at (5,1) .

Since the parabola is concave up, the minimum will be at the vertex of the parabola, which is at (5,1) .

Report an Error

Example Question #11 : Pre Calculus

What is the y-intercept of the following equation?

$f(x)=4\sin{3x}+2$

Possible Answers:

(0,3)

(0,2)

(4,0)

(0,4)

(2,0)

Correct answer:

(0,2)

Explanation:

The y-intercept can by found by solving the equation when x=0. Thus,

$f(0)=4\sin(3*0)+2$

f(0)=4*0+2

f(0)=2

Report an Error

Example Question #12 : Pre Calculus

Determine the y intercept of f(x) , where $f(x)=\frac{x-4}{x^{2}+x-12}$ .

Possible Answers:

(4,0)

(0,12)

$\bigg(\frac{1}{3},0\bigg)$

$\bigg(0,\frac{1}{3}\bigg)$

(12,0)

Correct answer:

$\bigg(0,\frac{1}{3}\bigg)$

Explanation:

In order to determine the y-intercept of f(x) , set x=0

$f(0)=\frac{0-4}{0^{2}+0-12}$

$f(0)=\frac{-4}{-12}$

$f(0)=\frac{1}{3}$

Solving for y, when x is equal to zero provides you with the y coordinate for the intercept. Thus the y-intercept is $\bigg(0,\frac{1}{3}\bigg)$ .

Report an Error

Example Question #3 : Graphing Quadratic Functions

What is the -intercept of the function,

f(x)=(x-2)^2+4 ?

Possible Answers:

Correct answer:

Explanation:

To find the -intercept we need to find the cooresponding value when x = 0 .

Substituting x = 0 into our function we get the following:

f(0)=(0-2)^2+4

f(0)=4+4

f(0)=8

Therefore, our -intercept is .

Report an Error

Example Question #4 : Graphing Quadratic Functions

What is the value of the -intercept of f(x)=(2x+4)^2+5 ?

Possible Answers:

The graph does not have a -intercept

Correct answer:

Explanation:

To find the -intercept we need to find the cooresponding value when x=0 . Therefore, we substitute in x=0 and solve:

f(0)=(2(0)+4)^2+5

f(0)=(0+4)^2+5

f(0)=16+5

f(0)=21

Report an Error

View Pre-Calculus Tutors

Sheela
Certified Tutor

University of Pittsburgh, Master of Science, Information Science.

View Pre-Calculus Tutors

Lawrence
Certified Tutor

Imperial College England, Bachelor of Engineering, Mechanical Engineering. Columbia University in the City of New York, Docto...

View Pre-Calculus Tutors

Joseph
Certified Tutor

Ottawa University-Ottawa, Bachelor of Science, Mathematics.

All Precalculus Resources

12 Diagnostic Tests 380 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

SSAT Tutors in San Diego, Spanish Tutors in San Francisco-Bay Area, SAT Tutors in San Francisco-Bay Area, English Tutors in Boston, LSAT Tutors in Washington DC, French Tutors in Seattle, SSAT Tutors in Miami, ACT Tutors in Atlanta, Math Tutors in Boston, Spanish Tutors in Denver

Popular Courses & Classes

GMAT Courses & Classes in Houston, MCAT Courses & Classes in Dallas Fort Worth, Spanish Courses & Classes in Philadelphia, LSAT Courses & Classes in Miami, SAT Courses & Classes in Dallas Fort Worth, LSAT Courses & Classes in San Francisco-Bay Area, SAT Courses & Classes in Washington DC, Spanish Courses & Classes in Atlanta, Spanish Courses & Classes in Denver, ACT Courses & Classes in Atlanta

Popular Test Prep

SSAT Test Prep in Miami, SAT Test Prep in San Francisco-Bay Area, LSAT Test Prep in Miami, ACT Test Prep in Atlanta, SSAT Test Prep in Atlanta, GRE Test Prep in Washington DC, MCAT Test Prep in Phoenix, GMAT Test Prep in Boston, SAT Test Prep in Phoenix, GRE Test Prep in Dallas Fort Worth

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Precalculus : Pre-Calculus

Study concepts, example questions & explanations for Precalculus

All Precalculus Resources

Example Questions

Example Question #1 : Conic Sections

Example Question #2 : Conic Sections

Example Question #2 : Pre Calculus

Example Question #1 : Pre Calculus

Example Question #1 : Introductory Calculus

Example Question #1 : Derivatives

Example Question #11 : Pre Calculus

Example Question #12 : Pre Calculus

Example Question #3 : Graphing Quadratic Functions

Example Question #4 : Graphing Quadratic Functions

All Precalculus Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: