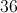Call Now to Set Up Tutoring:

(888) 888-0446

ISEE Lower Level Quantitative : Quadrilaterals

Study concepts, example questions & explanations for ISEE Lower Level Quantitative

Create An Account

All ISEE Lower Level Quantitative Resources

20 Diagnostic Tests 114 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #622 : Geometry

What is the width of the rectangle if the perimeter is 16in and the length is 4in ?

Possible Answers:

6in

5in

4in

7in

3in

Correct answer:

4in

Explanation:

The formula for perimeter of a rectangle is P=2(l + w)

To solve for the width we can plug our known values into the equation.

16=2(4+w)

16=8+2w

Subtract from both sides

8=2w

Divide by both sides

4=w

Report an Error

Example Question #582 : Geometry

What is the width of the rectangle if the perimeter is 10in and the length is 2in ?

Possible Answers:

4in

6in

5in

3in

7in

Correct answer:

3in

Explanation:

The formula for perimeter of a rectangle is P=2(l + w)

To solve for the width we can plug our known values into the equation.

10=2(2+w)

10=4+2w

Subtract from both sides

6=2w

Divide by both sides

3=w

Report an Error

Example Question #31 : Geometric Measurement: Recognizing Perimeter

What is the width of the rectangle if the perimeter is 32in and the length is 9in ?

Possible Answers:

7in

9in

11in

10in

8in

Correct answer:

7in

Explanation:

The formula for perimeter of a rectangle is P=2(l + w)

To solve for the width we can plug our known values into the equation.

32=2(9+w)

32=18+2w

Subtract from both sides

14=2w

Divide by both sides

7=w

Report an Error

Example Question #624 : Geometry

What is the width of the rectangle if the perimeter is 38in and the length is 10in ?

Possible Answers:

8in

9in

11in

10in

12in

Correct answer:

9in

Explanation:

The formula for perimeter of a rectangle is P=2(l + w)

To solve for the width we can plug our known values into the equation.

38=2(10+w)

38=20+2w

Subtract from both sides

18=2w

Divide by both sides

9=w

Report an Error

Example Question #32 : Geometric Measurement: Recognizing Perimeter

What is the width of the rectangle if the perimeter is 26in and the length is 8in ?

Possible Answers:

8in

6in

5in

9in

7in

Correct answer:

5in

Explanation:

The formula for perimeter of a rectangle is P=2(l + w)

To solve for the width we can plug our known values into the equation.

26=2(8+w)

26=16+2w

Subtract from both sides

10=2w

Divide by both sides

5=w

Report an Error

Example Question #1 : Squares

What is the area of a square if one side of the square is 6?

Possible Answers:

Correct answer:

Explanation:

If one side of a square is 6, then each of the four sides of the square are equal to 6. To find the area of a square, we multiply the length and the height together. The length is 6, and the height is 6, thus the equation we use is 6*6=36 .

Remember the formula for the area of a quadrilateral is length*width . For a square, one side is equal to both the length and the width.

Report an Error

Example Question #2 : Squares

One side of a square is centimeters long. What is the area of the square?

Possible Answers:

$16\: cm^{2}$

$8\: cm^{2}$

$64\: cm^{2}$

$32\: cm^{2}$

Correct answer:

$16\: cm^{2}$

Explanation:

The formula for finding the area of a square is $area = base\times height$ , or, because this is a square, $area = side^{2}$ .

area = centimeters $\times 4$ centimeters, or $16\: cm^{2}$

Report an Error

Example Question #3 : How To Find The Area Of A Square

A right triangle has a base of and a height of .

What is the area of the rectangle made by 2 of these triangles aligned along the hypotenuse?

Possible Answers:

Correct answer:

Explanation:

If one combines the 2 identical triangles, their base and height become the length and width of the rectangle.

Area of a rectangle is:

$length\times height$

In this case

$7\times 4=28$

Report an Error

Example Question #3 : Squares

A square has an area of $16\ in^{2}$ . What is the length of one side?

Possible Answers:

$\dpi{100} 8\ inches$

$\dpi{100} 4\ inches$

$\dpi{100} 16\ inches$

$\dpi{100} 2\ inches$

Correct answer:

$\dpi{100} 4\ inches$

Explanation:

You can find the area of a square by multiplying two sides together. All of the sides of a square are equal. In this case, $\dpi{100} 4\times 4=16$ , so the length of all of the sides of the square is 4 inches.

Report an Error

Example Question #5 : How To Find The Area Of A Square

Michaela drew th square below.

Screenshot_2015-03-25_at_3.13.25_pm

What is the area of the square?

Possible Answers:

144 square centimeters

48 square centimeters

100 square centimeters

12 square centimeters

112 square centimeters

Correct answer:

144 square centimeters

Explanation:

The area of a square can be found by multiplying the length of a side times itself. The side length of the above square is 12 cm. By finding 12 x 12, we find that the area of the square is 144 cm. squared.

Report an Error

View Tutors

Grace
Certified Tutor

St John's University-New York, Bachelor in Arts, Psychology. University of Bridgeport, Masters in Education, Education.

View Tutors

Gregory
Certified Tutor

Pennsylvania State University-Main Campus, Bachelor of Science, Chemical Engineering. Carnegie Mellon University, Doctor of P...

View Tutors

Brianna
Certified Tutor

Florida Atlantic University, Bachelor in Arts, Psychology. Florida Memorial University, Master of Arts Teaching, Reading Teac...

All ISEE Lower Level Quantitative Resources

20 Diagnostic Tests 114 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Biology Tutors in Miami, French Tutors in Atlanta, Physics Tutors in Miami, Calculus Tutors in San Diego, French Tutors in San Francisco-Bay Area, English Tutors in Dallas Fort Worth, Algebra Tutors in Denver, Spanish Tutors in San Francisco-Bay Area, Algebra Tutors in San Francisco-Bay Area, MCAT Tutors in Washington DC

Popular Courses & Classes

Spanish Courses & Classes in Houston, GMAT Courses & Classes in Boston, ACT Courses & Classes in Boston, SSAT Courses & Classes in San Francisco-Bay Area, GMAT Courses & Classes in Denver, MCAT Courses & Classes in San Diego, GMAT Courses & Classes in Seattle, LSAT Courses & Classes in Atlanta, LSAT Courses & Classes in Houston, GRE Courses & Classes in Boston

Popular Test Prep

ACT Test Prep in Boston, SSAT Test Prep in Washington DC, SSAT Test Prep in Chicago, SAT Test Prep in Houston, GMAT Test Prep in Atlanta, GRE Test Prep in Miami, ACT Test Prep in Washington DC, GMAT Test Prep in Boston, GRE Test Prep in Boston, GRE Test Prep in Phoenix

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

ISEE Lower Level Quantitative : Quadrilaterals

Study concepts, example questions & explanations for ISEE Lower Level Quantitative

All ISEE Lower Level Quantitative Resources

Example Questions

Example Question #622 : Geometry

Example Question #582 : Geometry

Example Question #31 : Geometric Measurement: Recognizing Perimeter

Example Question #624 : Geometry

Example Question #32 : Geometric Measurement: Recognizing Perimeter

Example Question #1 : Squares

Example Question #2 : Squares

Example Question #3 : How To Find The Area Of A Square

Example Question #3 : Squares

Example Question #5 : How To Find The Area Of A Square

All ISEE Lower Level Quantitative Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: