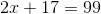Step 1: Find the Least Common Denominator of these fraction. We will list out multiples of each denominator until we find a common number for all three fractions...

The smallest common denominator is .

Step 2: Since the denominator is , we will convert all denominators to .

Step 3: Add up all the values of x...

Step 4: The result from step  is the numerator and  is the denominator. We will put these together.

Final Answer: 

A polynomial function with zeroes 3 and 7 has as its factors and . The function is given to be quadratic, so this function is

.

Apply the FOIL method to rewrite the polynomial:

Collect like terms:

,

the correct choice.

In order to solve for the variable, , we need to isolate it on the left side of the equation. We will do this by reversing the operations done to the variable by performing the opposite of each operation on both sides of the equation. 

Let's begin by rewriting the given equation.

Subtract  from both sides of the equation.

Simplify.

Multiply both sides of the equation by .

Solve.

can be rewritten as the compound inequality

.

The solution set will be the union of the two individual solution sets. Find the solution set of the first inequality as follows:

Isolate by first subtracting from both sides:

Divide both sides by , reversing the direction of the inequality symbol, since you are dividing by a negative number:

.

In interval notation, this is the set .

Find the solution set of the other inequality similarly:

In interval notation, this is the set .

The union of these sets is the solution set: .

Solve for in the equation

by isolating on the left side. Do this by reversing the operations in the reverse of the order of operations.

First, subtract 17 from both sides:

Now, divide both sides by 2:

One way to find 25% of this value is to multiply 41 by 25 and divide by 100:

,

the correct choice.

Given a quadratic function

the vertex will always be

.

Thus, since our function is 

, , and .

We plug these variables into the formula to get the vertex as

.

Hence, the vertex of

is

.

The discriminant of a quadratic polynomial

is given by

.

Thus, since our quadratic polynomial is 

,

, , and . 

Plugging these values into the discriminant equation, we find that the discriminant is

.

A polynomial equation of the form

has one and only one (real) solution if and only if its discriminant is equal to zero - that is, if its coefficients satisfy the equation

In each of the choices,  and , so it suffices to determine the value of  which satisfies this equation. Substituting, we get

Solve for  by first adding 400 to both sides:

Take the square root of both sides:

The choice that matches this value of  is the equation

To determine the nature of the solution set of a quadratic equation, it is necessary to first express it in standard form 

To accomplish this, first, multiply the binomials on the left using the FOIL technique:

Collect like terms:

Add 6 to both sides:

The key to determining the nature of the solution set is to examine the discriminant . Setting , the value of the discriminant is 

The discriminant is a positive number; furthermore, it is a perfect square, being equal to the square of 11. Therefore, the solution set comprises two rational solutions.

A polynomial equation of the standard form

has one and only one (real) solution if and only if its discriminant is equal to zero - that is, if its coefficients satisfy the equation

Each of the choices can be rewritten in standard form by subtracting the term on the right from both sides. One of the choices can be rewritten as follows:

By similar reasoning, the other four choices can be written:

In each of the five standard forms,  and , so it is necessary to determine the value of  that produces a zero discriminant. Substituting accordingly:

Add 900 to both sides and take the square root:

Of the five standard forms, 

fits this condition. This is the standard form of the equation 

,

the correct choice.