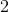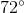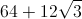In general, the formula for the area of a trapezoid is (1/2)(a + b)(h), where a and b are the lengths of the bases, and h is the length of the height. Thus, we can write the area for the trapezoid given in the problem as follows:

area of trapezoid = (1/2)(4 + s)(s)

Similarly, the area of a square with sides of length a is given by a². Thus, the area of the square given in the problem is s².

We now can set the area of the trapezoid equal to the area of the square and solve for s.

(1/2)(4 + s)(s) = s²

Multiply both sides by 2 to eliminate the 1/2.

(4 + s)(s) = 2s²

Distribute the s on the left.

4s + s² = 2s²

Subtract s² from both sides.

4s = s²

Because s must be a positive number, we can divide both sides by s.

4 = s

This means the value of s must be 4.

The answer is 4.

In order to find the area of an isoceles trapezoid, you must average the bases and multiply by the height.  

The average of the bases is straight forward:

In order to find the height, you must draw  an altitude. This creates a right triangle in which one of the legs is also the height of the trapezoid.  You may recognize the Pythagorean triple (6-8-10) and easily identify the height as 8. Otherwise, use .  

Multiply the average of the bases (12) by the  height (8) to get an area of 96.

The sum of the angles in any quadrilateral is 360°, and the properties of an isosceles trapezoid dictate that the sets of angles adjoined by parallel lines (in this case, the bottom set and top set of angles) are equal. Subtracting 2(72°) from 360° gives the sum of the two top angles, and dividing the resulting 216° by 2 yields the measurement of x, which is 108°.

The perimeter of the isoceles trapezoid is the sum of all the sides.  You can assume the left side is also 10 in. because it is an isoceles trapezoid. 

The formula for the perimeter of a trapezoid is:

Where  is the base and  is the edge

Plugging in our values, we get:

Use the formula for  triangles in order to find the lengths of all the sides and bases.

The formula is:

Where  is the length of the side opposite the .

Beginning with the  side, if we were to create a  triangle, the length of the base is , and the height is .

Creating another  triangle on the left, we find the height is , the length of the base is , and the side is .

The formula for the perimeter of a trapezoid is:

,

where  is the length of the base and  is the length of the edge.

Plugging in our values, we get:

The formula for the perimeter of a trapezoid is:

,

where  is the length of the base and  is the length of the edge.

Plugging in our values, we get:

The formula for the perimeter of a trapezoid is

.

Use the formula for a  triangle to find the length of the base and side:

Use the formula for a  triangle to find the length of the base and side:

Plugging in our values, we get:

The triangle on the left side of the figure has a and a  angle. Since all of the angles of a triangle must add up to , we can find the angle measure of the third angle:

Our third angle is and we have a triangle.

A triangle has sides that are in the corresponding ratio of . In this case, the side opposite our angle is , so

We also now know that

Now we know all of our missing side lengths.  The right and left side of the parallelogram will each be . The bottom and top will each be . Let's combine them to find the perimeter: