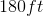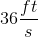Call Now to Set Up Tutoring:

(888) 888-0446

College Physics : College Physics

Study concepts, example questions & explanations for College Physics

Create An Account

All College Physics Resources

66 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Motion Diagrams

The bases on a baseball field are 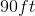90ft apart in a perfect square.

A player hits a home run and goes around all 4 bases. What is the total distance he travelled?

Possible Answers:

360ft

180ft

450ft

0ft

270ft

Correct answer:

360ft

Explanation:

There is 90 feet between each base and distance does not depend on direction. Distance is scaler therefore, the batter travels 360 feet.

Report an Error

Example Question #41 : College Physics

The bases on a baseball field are 90 feet apart.

A players hits a home run and gets around the bases in 20 seconds what is the players total velocity?

Possible Answers:

$20\frac{ft}{s}$

$0\frac{ft}{s}$

$18\frac{ft}{s}$

$36\frac{ft}{s}$

$15\frac{ft}{s}$

Correct answer:

$0\frac{ft}{s}$

Explanation:

$Velocity = \frac{displacement}{time}$

Displacement is a vector. Magnitude and direction matter.

$Displace = 0 \rightarrow Velocity= 0$

Report an Error

Example Question #2 : Motion Diagrams

The bases on a baseball field are 90ft apart.

A player hits a home run and runs around all four bases. What is his total displacement?

Possible Answers:

90ft

360ft

270ft

0ft

180ft

Correct answer:

0ft

Explanation:

Displacement is a vector. Therefore, magnitude and direction matters and because direction matters the total displacement is 0 feet.

Report an Error

Example Question #3 : Motion Diagrams

A 2kg ball is dropped and it hits the floor is 4 s . How high was the ball dropped from?

$g=10\frac{m}{s^2}$

Possible Answers:

120 m

100 m

80 ft

160 m

80 m

Correct answer:

80 m

Explanation:

We are dealing with motion in one plane and the initial velocity is zero. We must use the following kinematics equation to plug in known values and solve:

$d = \frac{1}{2}at^2$

$d = \frac{1}{2}(10\frac{m}{s^2})(4s^2)$

d = 80m

Report an Error

Example Question #42 : College Physics

There are 90 ft between the bases on a baseball field and they make a square.

Jim hits a double and reaches second base in 12 s . What is his velocity?

Possible Answers:

$30\frac{ft}{s}$

$90\frac{ft}{s}$

$15\frac{ft}{s}$

$21.2\frac{ft}{s}$

$3\frac{ft}{s}$

Correct answer:

$21.2\frac{ft}{s}$

Explanation:

$Speed=\frac{Distance}{Time}$

$Speed=\frac{180ft}{12s}=15\frac{ft}{s}$

Velocity is a vector so direction matters. Therefore this creates a right triangle with the two isosceles side having a velocity of $15\frac{ft}{s}$ . Therefore, the hypotenuse is $21.2\frac{ft}{s}$ when you perform Pythagorean theorem.

Report an Error

Example Question #1 : Forces

A juggler throws a ball straight up into the air, which exerts air resistance on the ball. Which of the following best describes the speed of the ball after it falls and just reaches the juggler's hand?

Possible Answers:

The speed of the ball is greater than when it was released.

The speed of the ball is zero.

None of the other answers.

The speed of the ball is exactly the same as when it was released.

The speed of the ball is less than when the juggler first threw it.

Correct answer:

The speed of the ball is less than when the juggler first threw it.

Explanation:

Air resistance is like a friction force that takes energy away from the ball. Because the ball has energy taken from it on the way up and the way down, there is net negative work done on the ball. The energy of the ball is not conserved and it slows down slightly compared to when it was released.

Report an Error

Example Question #1 : Newton's Laws And Fundamental Concepts

A 50 kg cinder block sitting on a frictionless surface is attached to a rope. If the rope is pulled parallel to the surface such that the block accelerates at a rate of $5\frac{m}{s^2}$ , what is the minimum amount of tension the rope must be able to support without breaking?

Possible Answers:

2450 N

10 N

490 N

250 N

740 N

Correct answer:

250 N

Explanation:

This is a simple case of F=ma . In this instance, is 50 kg and is $5\frac{m}{s^2}$ . Substituting, we find F=250 N .

Report an Error

Example Question #1 : Newton's Laws And Fundamental Concepts

A 50 kg cinder block sitting on the ground is attached to a rope. If the rope is pulled such that the block accelerates directly upwards at a rate of $5\frac{m}{s^2}$ , what is the minimum amount of tension the rope must be able to support without breaking?

Possible Answers:

741 N

241 N

50 N

250 N

10N

Correct answer:

741 N

Explanation:

In this case, the rope must be able to support both the force of gravity and the force required to accelerate the block. Both are given by F=ma :

$F_{gravity} = (50 kg)(9.81\frac{m}{s^2}) = 490.5 N$

$F_{accelerate} = (50 kg)(5\frac{m}{s^2}) = 250 N$

$F_{total} = 490.5 N + 250 N = 740.5 N$

Report an Error

Example Question #3 : Newton's Laws And Fundamental Concepts

A 5kg object experiences a force of 27N in its direction of motion for . What is the change in the velocity the object moved at during this time?

Possible Answers:

$51.6\frac{m}{s}$

$61.6\frac{m}{s}$

$41.6\frac{m}{s}$

$31.6\frac{m}{s}$

$21.6\frac{m}{s}$

Correct answer:

$21.6\frac{m}{s}$

Explanation:

Let's first write down the information we are given:

F=27N

m=5kg

t=4s

We know that F=m*a . This equation can simplified to $F=\frac{m*v}{t}$ since acceleration is equal to velocity over time so $a=\frac{v}{t}$ .

Let's manipulate the equation by solving for velocity so we can simply plug in the numbers we are given:

t*F=m*v

so: $v=t*\frac{F}{m}$

$4*\frac{27}{5}=21.6\frac{m}{s}$ which is our final answer.

Report an Error

Example Question #4 : Newton's Laws And Fundamental Concepts

A 15kg experiences a net force that increases its velocity by $17\frac{m}{s}$ from rest for . What is the magnitude of this force?

Possible Answers:

36N

30N

21.25N

42.5N

50N

Correct answer:

42.5N

Explanation:

Let's write down the information we are given:

m=15kg

$v=17\frac{m}{s}$

t=6s

We know that F=m*a . This equation can simplified to $F=m\frac{v}{t}$ since acceleration is equal to velocity over time so $a=\frac{v}{t}$ .

So we can just plug in the information we are given to solve for the Force:

$15*\frac{17}{6}=42.5N$ , which is our final answer.

Report an Error

View Tutors

Alejandro
Certified Tutor

Kennesaw State University, Bachelor of Science, Computer Software Engineering.

View Tutors

Joy
Certified Tutor

Boston University, Bachelor in Arts, Conservation Biology.

View Tutors

Chiquita
Certified Tutor

Troy University, Bachelor of Science, Education. University of Phoenix-Atlanta Campus, Master of Arts, Adult and Continuing E...

All College Physics Resources

66 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

English Tutors in San Diego, Math Tutors in Boston, Statistics Tutors in Denver, MCAT Tutors in Boston, French Tutors in Washington DC, Biology Tutors in Miami, French Tutors in San Diego, Spanish Tutors in Chicago, Math Tutors in Seattle, ACT Tutors in San Francisco-Bay Area

Popular Courses & Classes

MCAT Courses & Classes in Seattle, GRE Courses & Classes in Denver, LSAT Courses & Classes in Philadelphia, SSAT Courses & Classes in Denver, MCAT Courses & Classes in San Diego, LSAT Courses & Classes in Chicago, LSAT Courses & Classes in Boston, MCAT Courses & Classes in Chicago, SAT Courses & Classes in Atlanta, LSAT Courses & Classes in Los Angeles

Popular Test Prep

MCAT Test Prep in Boston, LSAT Test Prep in Los Angeles, MCAT Test Prep in Washington DC, SAT Test Prep in Phoenix, SAT Test Prep in Seattle, MCAT Test Prep in Chicago, GMAT Test Prep in Miami, SSAT Test Prep in Boston, GRE Test Prep in Los Angeles, ACT Test Prep in Washington DC

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

College Physics : College Physics

Study concepts, example questions & explanations for College Physics

All College Physics Resources

Example Questions

Example Question #1 : Motion Diagrams

Example Question #41 : College Physics

Example Question #2 : Motion Diagrams

Example Question #3 : Motion Diagrams

Example Question #42 : College Physics

Example Question #1 : Forces

Example Question #1 : Newton's Laws And Fundamental Concepts

Example Question #1 : Newton's Laws And Fundamental Concepts

Example Question #3 : Newton's Laws And Fundamental Concepts

Example Question #4 : Newton's Laws And Fundamental Concepts

All College Physics Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: