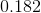Let's write down the information we are given:

We know that . This equation can simplified to    since acceleration is equal to velocity over time so .

So we can solve for  in this equation:

, which is our final answer.

Initially, when all the sand is in the upper chamber, the reading on the scale is constant and corresponds to the weight of the hourglass and the sand within. As some sand falls, there is no normal force on it so the scale can not register its weight. The fraction of sand that is falling is small, so there is only a small decrease in the apparent weight of the hourglass.

Let's first write down the information we are given:

We know that . This equation can simplified to  since acceleration is equal to velocity over time so .

Let's manipulate the equation by solving for time so we can simply plug in the numbers we are given:

so: 

= which is our final answer.

The gravitational force obeys an inverse-square law. Decreasing the distance by a factor of  will increase the force by a factor of , or .

 is the equation for Universal gravitation.

So the fact that we don't actually know any of the numbers doesn't matter. 

What matters is we have the equation 

The masses have a direct proportional relationship with the force. That means that if a mass doubles, the force doubles. 

We can also see that the radius (distance between the masses) has an inverse square relationship with Force. So if we double the radius, we divide the force by 4.

So based on the problem, we triple a mass and double the radius. So we triple the force then divide by 4.

In this question, we're told that an object is hanging by being supported by two ropes. We're asked to find the tension in each rope.

When answering this question, we have to keep in mind all of the forces acting on the object. Since the object isn't moving, there is no net force acting on it. Moreover, we know that its weight acts downward while the tension in the ropes act upwards.

For this question, we're told that an object hanging from a string is released from an initial height and allowed to swing. We're given the maximum velocity, the object's mass, and the length of the string. We're asked to find the maximum tension in the string.

The first step towards solving this problem is to realize that we're dealing with centripetal motion. When the object is released from an initial height, it begins to fall. But as it falls, the string exerts a tension on the mass and causes the mass to travel in a circular motion. Thus, the tension of the string is supplying the centripetal force.

Furthermore, it's important to remember the point at which the object will have maximum velocity. When the object was initially released from some initial height, all of the object's energy was in the form of gravitational potential energy. As it falls, that potential energy is converted into kinetic energy. Once the object reaches the very bottom of its path of motion, all of the potential energy it once had has been converted into kinetic energy at that instant. Since all of the energy is in the form of kinetic energy, and the object's mass remains constant, we know that this must be the point where the maximum velocity occurs.

Thus, we need to analyze the situation when the mass has swung to the very bottom of the path. At this point, the tension is providing an upward force. Moreover, the only downward force acting on the object is its weight due to gravity. The net force resulting from these two will result in the overall centripetal force on the object. We can put this into an equation as follows.

Since we're trying to solve for the tension in the string, we can isolate the  term in the above expression.

To solve this problem, we first need to take into account the forces acting in the vertical direction separately from the forces acting in the horizontal direction.

First, let's start with the vertical direction. Here, the only force force acting downward is the weight of the box. There are two forces acting upwards on the box; one is the normal force and the other is the vertical component of the tension in the rope that is pulling the box. Because the box is not moving in the vertical direction, there is no net force. Thus, the sum of these forces is equal to zero.

Next, let's take a look at the forces acting horizontally on the box. Acting to the right of the box is the horizontal component of the tension in the rope. Acting to the left is the frictional force. Because the box is moving to the right, it must have experienced a net force in this direction. Thus, the sum of these horizontally acting forces will equal a net force.

Now, we can take the expression we obtained for the normal force and substitute it into the expression we obtained for the horizontally acting forces.

Now that we've found an expression for the coefficient of kinetic friction, all we need to do is plug in the values given in the question stem to arrive at the answer.

All of these are identical to a normal force.