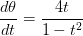Call Now to Set Up Tutoring:

(888) 888-0446

Calculus AB : Calculus AB

Study concepts, example questions & explanations for Calculus AB

Create An Account

All Calculus AB Resources

45 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #4 : Calculate Rates Of Change And Related Rates

The position of a car is given by the equation

$f(t) = sin(\pi t) + 3t^{2} - 10t + 7$ .

Find the car's acceleration when t = 4 .

Possible Answers:

$-\pi^{3}$

$\pi$ + 14

Correct answer:

Explanation:

To find the car's acceleration, take the SECOND derivative of f(t) .

$f'(t) = \pi cos(\pi t) + 6t - 10$ , and

$f''(t) = -\pi ^{2}sin(\pi t) + 6$ .

The car's position at t = 4 is then given by:

$f''(4) = -\pi ^{2}sin(\pi *4) + 6 = 6$

Report an Error

Example Question #5 : Calculate Rates Of Change And Related Rates

A point on a circle of radius 1 unit is orbiting counter-clockwise around the circle's center. It makes one full orbit every 8 seconds. How fast is the coordinate changing when the line segment from the origin to the point, (x,y) , forms an angle of $\frac{\pi}{4}$ radians above the positive x-axis?

Possible Answers:

$\frac{1}{2}$

$\frac{-\sqrt{2}\pi}{8}$

$-\frac{\sqrt{3}\pi}{8}$

$\frac{\sqrt{2}\pi}{8}$

$\frac{-\sqrt{2}}{2}$

Correct answer:

$\frac{-\sqrt{2}\pi}{8}$

Explanation:

This is a related rates problem.

Since the problem gives the time for one orbit, we can find the angular speed of the point. The angular speed is simply how many radians the particle travels in one second. We find this by dividing the number of radians in one revolution, $2\pi$ , by the time it takes to travel one revolution, 8 seconds.

$\frac{2\pi}{8} = \frac{\pi}{4}$

This gives us the change in the angle with respect to time, $\frac{\mathrm{d} \theta}{\mathrm{d} t} = \frac{\pi}{4}$ .

Now we need to relate the position to the angle, $\theta$ . Recall that

$\cos \theta = \frac{x}{r}$ , where r is the radius.

Since the radius is given as 1 unit, we can write this equation as

$x = \cos \theta$ .

Now we take the derivative of both sides with respect to time, using implicit differentiation. Remember that we use the chain rule for any variable that is not . This gives,

$\frac{\mathrm{d} x}{\mathrm{d} t} = -\sin\theta \frac{\mathrm{d} \theta}{\mathrm{d} t}$ .

Now we have a formula that relates the horizontal speed of the particle at an instant in time, $\frac{\mathrm{d} x}{\mathrm{d} t}$ , to the angle above the positive x-axis and angular speed at that same instant. We are told to find how fast the x coordinate is changing when the angle, $\theta$ is $\frac{\pi}{4}$ radians above the positive x-axis. So we will plug in $\frac{\pi}{4}$ for $\theta$ . However, we also need to know $\frac{\mathrm{d} \theta}{\mathrm{d} t}$ . Fortunately, we already found it. It is the angular speed, $\frac{\pi}{4}$ radians/second.

Plugging this information in, we get

$\frac{\mathrm{d} x}{\mathrm{d} t}= - \sin{(\frac{\pi}{4})} (\frac{\pi}{4})$

$\frac{\mathrm{d} x}{\mathrm{d} t} = -(\frac{\sqrt{2}}{2})(\frac{\pi}{4})$

$\frac{\mathrm{d} x}{\mathrm{d} t}= \frac{-\sqrt{2}\pi}{8}$

This is the answer. The negative makes sense because the point is traveling counter-clockwise. Hence, it is moving left when the angle is $\frac{\pi}{4}$ radians.

Report an Error

Example Question #1 : Calculate Rates Of Change And Related Rates

A man is standing on the top of a 10 ft long ladder that is leaning against the side of a building when the bottom of the ladder begins to slide out from under it. How fast is the man standing on the top of the ladder falling when the bottom of the ladder is 6 ft from the building and is sliding at 2ft/sec?

Possible Answers:

$3/4 \text{ feet/sec}$

$-1/4 \text{ feet/sec}$

$32 \text{ feet/sec}$

$-3/2 \text{ feet/sec}$

Correct answer:

$-3/2 \text{ feet/sec}$

Explanation:

This is a related rates problem. The ladder leaning against the side of a building forms a right triangle, with the 10ft ladder as its hypotenuse. The Pythagorean Theorem, a^2 + b^2 = c^2 relates all three sides of this triangle to each other. Let be the height from the top of the ladder to the ground. Let be the distance from the bottom of the ladder to the building. Since 10 is the hypotenuse, we have the following equation.

x^2 + y^2 = 10^2

Simplifying the right side gives us

x^2 + y^2 = 100

Since and are variables, we will wait to plug values into them until after we take the derivative.

The question asks how fast the man standing on the top of the ladder is falling whenthe ladder's base is 6ft from the building and is sliding away at 2 ft/sec. These two values, x=6 and $\frac{\mathrm{d} x}{\mathrm{d} t}=2$ , only happen at a single instant in time. So we will find the derivative of the equation at this point in time.

Using implicit differentiation to find the derivative with respect to time, we get

$2x \frac{\mathrm{d} x}{\mathrm{d} t} + 2y\frac{\mathrm{d} y}{\mathrm{d} t} = 0$

We only care about the instant that x=6 and $\frac{\mathrm{d} x}{\mathrm{d} t}=2$ . We need to find the rate that the top of the ladder, and thus the man, is falling. So we want to solve for $\frac{\mathrm{d} y}{\mathrm{d} t}$ . However, we will need to know what is at this instant in order to find an answer. Fortunately, the Pythagorean Theorem applies at all points in time, so we can use it for this particular instant to find .

6^2 + y^2 = 100

36 + y^2 = 100

y^2 = 64

$y = \pm 8$

Since we are dealing with physical distances, we will only use the positive 8.

Plugging all the information into our derivative equation gives us

$2x \frac{\mathrm{d} x}{\mathrm{d} t} + 2y\frac{\mathrm{d} y}{\mathrm{d} t} = 0$

$2(6)(2) + 2(8)\frac{\mathrm{d} y}{\mathrm{d} t}=0$

$24+ 16\frac{\mathrm{d} y}{\mathrm{d} t}=0$

$16\frac{\mathrm{d} y}{\mathrm{d} t}= -24$

$\frac{\mathrm{d} y}{\mathrm{d} t}= -3/2$

The negative makes sense because the man is falling down, so the height is getting smaller. Thus our answer is $-3/2 \text{ feet/sec}$

Report an Error

Example Question #6 : Calculate Rates Of Change And Related Rates

The velocity of a car is given by the equation:

$v(t) = 25e^{-t} + 15t$ , where is the time in hours.

If the car starts out at a distance of 3 miles from its home, how far will it be after 4 hours?

Possible Answers:

$98 + \frac{25}{e^{4}}$

$148 -\frac{25}{e^{4}}$

$148 + \frac{25}{e^{4}}$

$145 - \frac{25}{e^{4}}$

$95 + \frac{25}{e^{4}}$

Correct answer:

$148 -\frac{25}{e^{4}}$

Explanation:

To find the total distance traveled, the velocity function has to be integrated from t=0 to t=4 hours:

$distance = \int_{t=0}^{t=4} v(t) dt = \int_{t=0}^{t=4} (25e^{-t} + 15t) dt$

$distance = -25e^{-4} + \frac{15(4)^{2}}{2} - (-25e^{0} + \frac{15(0)^{2}}{2})$

$distance = \frac{-25}{e^{4}} + 120 - (-25 + 0) = \frac{-25}{e^{4}} + 145$

Finally, the question is asking how far the car will be from home. It was 3 miles from home when t=0 , so at t=4 , it will be:

$3 + \frac{-25}{e^{4}} + 145 = 148 -\frac{25}{e^{4}}$ miles from home.

Report an Error

Example Question #1 : Calculate Rates Of Change And Related Rates

A right triangle has sides of length and which are both increasing in length over time such that:

x(t)=2t

y(t)=4t^2

a) Find the rate at which the angle $\theta$ opposite y(t) is changing with respect to time.

Possible Answers:

$\frac{d\theta}{dt}=\frac{4t}{1-t^2}$

$\frac{d\theta}{dt}=\cos(t)-\frac{1}{1+t}$

$\frac{d\theta}{dt}=t+\frac{4}{1+2t^2}$

$\frac{d\theta}{dt}=\sin(t)+\frac{1}{1+t}$

$\frac{d\theta}{dt}=\frac{2}{1+4t^2}$

Correct answer:

$\frac{d\theta}{dt}=\frac{2}{1+4t^2}$

Explanation:

A right triangle has sides of lenght and which are both increasing in length over time such that:

x(t)=2t

y(t)=4t^2

Find the rate at which the angle $\theta$ opposite y(t) is changing with respect to time.

Screen shot 2020 09 04 at 11.29.59 am

a) First, we need to write an expression for the angle $\theta$ as a function of . Because the angle is opposite the side we know that the tangent is simply y/x . Take the inverse of the tangent:

$\theta (t)\, \, =\, \, \tan^{-1}\left(\frac{y(t)}{x(t)} \right )\: \: =\: \: \tan^{-1}\left(\frac{4t^2}{2t}\right)\, \, =\, \, \tan^{-1}\left(2t \right )$

$\theta(t)=\tan^{-1}(2t)$

Now we need to differentiate with respect to .

Recall the general derivative for the inverse tangent function is:

$\frac{d}{dx}\tan^{-1}(x)=\frac{1}{1+x^2}$

Applying this to our function for $\theta(t)$ , and remembering to use the chain rule, we obtain:

$\frac{d\theta}{dt}=\frac{d}{dt}\tan^{-1}(2t)=\frac{2}{1+(2t)^2}=\frac{2}{1+4t^2}$

$\frac{d\theta}{dt}=\frac{2}{1+4t^2}$

Report an Error

Example Question #2 : Calculate Rates Of Change And Related Rates

Soap is sometimes used to determine the location of leaks in industrial pipes. A perfectly spherical soap bubble is growing at a rate of 5 mm^3/sec . What is the rate of change of the surface area of the bubble when the radius of the bubble is 10 mm ?

Possible Answers:

$\frac{1}{80\pi} mm^2/sec$

1 mm^2/sec

80 mm^2/sec

$80\pi mm^2/sec$

Correct answer:

1 mm^2/sec

Explanation:

To determine the rate of change of the surface area of the spherical bubble, we must relate it to something we do know the rate of change of - the volume.

The volume of a sphere is given by the following:

$V=\frac{4\pi}{3}r^3$

The rate of change of the volume is given by the derivative with respect to time:

$\frac{\mathrm{d} V}{\mathrm{d} t}=4\pi r^2 \frac{\mathrm{d} r}{\mathrm{d} t}$

The derivative was found using the following rules:

$\frac{\mathrm{d} }{\mathrm{d} x} f(g(x))=f'(g(x)) \cdot g'(x)$ , $\frac{\mathrm{d} }{\mathrm{d} x}x^n=nx^{n-1}$

We must now solve for the rate of change of the radius at the specified radius, so that we can later solve for the rate of change of surface area:

$5=4\pi (100) \frac{\mathrm{d} r}{\mathrm{d} t}$

$\frac{\mathrm{d} r}{\mathrm{d} t}=\frac{1}{80\pi}$

Next, we must find the surface area and rate of change of the surface area of the sphere the same way as above:

$A=4\pi r^2$

$\frac{\mathrm{d} A}{\mathrm{d} t}=8 \pi r \frac{\mathrm{d} r}{\mathrm{d} t}$

Plugging in the known rate of change of the surface area at the specified radius, and this radius into the rate of surface area change function, we get

$\frac{\mathrm{d} A}{\mathrm{d} t}=8\pi (10)\cdot \frac{1}{80\pi}= 1mm^2/sec$

Report an Error

Example Question #1 : Calculate Rates Of Change And Related Rates

A pizzeria chef is flattening a circular piece of dough. The surface area of the dough (we are only considering the top of the dough) is increasing at a rate of 0.5 inches/sec. How quickly is the diameter of the pizza changing when the radius of the pizza measures 4 inches?

Possible Answers:

inches/sec

$\frac{1}{16 \pi}$ inches/sec

inches/sec

$\frac{1}{8 \pi}$ inches/sec

Correct answer:

$\frac{1}{8 \pi}$ inches/sec

Explanation:

To find the rate of change of the diameter, we must relate the diameter to something we do know the rate of change of: the surface area.

The surface area of the top side of the pizza dough is given by

$A=\pi r ^2$

The rate of change, then, is found by taking the derivative of the function with respect to time:

$\frac{\mathrm{d} A}{\mathrm{d} t}=2\pi r \frac{\mathrm{d} r}{\mathrm{d} t}$

Solving for the rate of change of the radius at the given radius, we get

$\frac{1}{2}=2\pi (4)\frac{\mathrm{d} r}{\mathrm{d} t}$

$\frac{\mathrm{d} r}{\mathrm{d} t}=\frac{1}{16\pi}$ inches/sec

Now, we relate the diameter to the radius of the pizza dough:

2r=d

Taking the derivative of both sides with respect to time, we get

$2 \frac{\mathrm{d} r}{\mathrm{d} t}=\frac{\mathrm{d} d}{\mathrm{d} t}$

Plugging in the known rate of change of the radius at the given radius, we get

$\frac{\mathrm{d} d}{\mathrm{d} t}=\frac{1}{8\pi}$ inches/sec

We could have found this directly by writing our surface area formula in terms of diameter, however the process we used is more applicable to problems in which the related rate of change is of something not as easy to manipulate.

Report an Error

Example Question #2 : Calculate Rates Of Change And Related Rates

A spherical balloon is increasing in volume at a constant rate of 0.1 cm^3/min . At a radius of 3 cm, what is the rate of change of the circumference of the balloon?

Possible Answers:

$\frac{1}{90\pi} cm/min$

$\frac{1}{180} cm/min$

$\frac{1}{90} cm/min$

$\frac{1}{360} cm/min$

$\frac{1}{360\pi} cm/min$

Correct answer:

$\frac{1}{180} cm/min$

Explanation:

To determine the rate of change of the circumference at a given radius, we must relate the circumference rate of change to the rate of change we know - that of the volume.

Starting with the equation for the volume of the spherical balloon,

$V=\frac{4}{3}\pi r^3$

we take the derivative of the function with respect to time, giving us the rate of change of the volume:

$\frac{\mathrm{d} V}{\mathrm{d} t}=4\pi r^2 \frac{\mathrm{d} r}{\mathrm{d} t}$

The derivative was found using the following rules:

$\frac{\mathrm{d} }{\mathrm{d} x} x^n=nx^{n-1}$ , $\frac{\mathrm{d} }{\mathrm{d} x} f(g(x))=f'(g(x))\cdot g'(x)$

The chain rule was used when taking the derivative of the radius with respect to time, because we know that it is a function of time.

Solving for $\frac{\mathrm{d} r}{\mathrm{d} t}$ using our known $\frac{\mathrm{d} V}{\mathrm{d} t}$ at the given radius, we get

$\frac{\mathrm{d} r}{\mathrm{d} t}=\frac{1}{360\pi} cm/min$

Now, we use this rate of change and apply it to the rate of change of the circumference, which we get by taking the derivative of the circumference with respect to time:

$c=2\pi r$

$\frac{\mathrm{d} c}{\mathrm{d} t}=2\pi \frac{\mathrm{d} r}{\mathrm{d} t}$

Solving for the rate of change of the circumference by plugging in the known rate of change of the radius, we get

$\frac{\mathrm{d} c}{\mathrm{d} t}=2\pi (\frac{1}{360 \pi})=\frac{1}{180} cm/min$

Report an Error

Example Question #3 : Calculate Rates Of Change And Related Rates

Determine the rate of change of the angle opposite the base of a right triangle -whose length is increasing at a rate of 1 inch per minute, and whose height is a constant 2 inches - when the area of the triangle is 2 square inches.

Possible Answers:

$\frac{1}{4}$ radians per minute

$\frac{1}{\sqrt{2}}$ radians per minute

$\frac{1}{4\sqrt{2}}$ radians per minute

$\frac{1}{2\sqrt{2}}$ radians per minute

Correct answer:

$\frac{1}{\sqrt{2}}$ radians per minute

Explanation:

To determine the rate of the change of the angle opposite to the base of the given right triangle, we must relate it to the rate of change of the base of the triangle when the triangle is a certain area.

First, we must determine the length of the base of the right triangle at the given area:

$A=\frac{bh}{2}$

4=b(2)

b=2

Now, we must find something that relates the angle opposite of the base to the length of the base and height - the tangent of the angle:

$\tan \theta=\frac{b}{h}$

To find the rate of change of the angle, we take the derivative of both sides with respect to time, keeping in mind that the base of the triangle is dependent on time, while the height is constant:

$\sec^2(\theta)\frac{\mathrm{d} \theta}{\mathrm{d} t}=\frac{2}{2}\frac{\mathrm{d} b}{\mathrm{d} t}$

We know the rate of change of the base, and we can find the angle from the sides of the triangle:

$\tan(\theta)=1$

$\theta=\frac{\pi}{4}$

Plugging this and the other known information in and solving for the rate of change of the angle adjacent to the base, we get

$\sec^2(\frac{\pi}{4})\frac{\mathrm{d} \theta}{\mathrm{d} t}=1$

$\frac{\mathrm{d} \theta}{\mathrm{d} t}=\frac{1}{\sqrt{2}}$ radians per minute

Report an Error

Example Question #4 : Calculate Rates Of Change And Related Rates

The position of a car is given by the equation

$f(t) = sin(\pi t) + 3t^{2} - 10t + 7$ .

Find the car's acceleration when t = 4 .

Possible Answers:

$-\pi^{3}$

$\pi$ + 14

Correct answer:

Explanation:

To find the car's acceleration, take the SECOND derivative of f(t) .

$f'(t) = \pi cos(\pi t) + 6t - 10$ , and

$f''(t) = -\pi ^{2}sin(\pi t) + 6$ .

The car's position at t = 4 is then given by:

$f''(4) = -\pi ^{2}sin(\pi *4) + 6 = 6$

Report an Error

View Tutors

Lisa
Certified Tutor

Ashford University, Masters in Education, Curriculum and Instruction. University of Arizona, Master of Arts, Psychology.

View Tutors

Larry
Certified Tutor

Southeastern Oklahoma State University, Bachelor of Science, Microbiology.

View Tutors

Robert
Certified Tutor

University of Illinois at Chicago, Bachelor of Fine Arts, Graphic Design. DeVry Universitys Keller Graduate School of Managem...

All Calculus AB Resources

45 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

SSAT Tutors in San Diego, GMAT Tutors in Phoenix, SAT Tutors in Boston, GMAT Tutors in Denver, Calculus Tutors in Dallas Fort Worth, Computer Science Tutors in Washington DC, MCAT Tutors in Boston, ACT Tutors in Seattle, Spanish Tutors in Chicago, ISEE Tutors in Boston

Popular Courses & Classes

MCAT Courses & Classes in San Francisco-Bay Area, MCAT Courses & Classes in San Diego, LSAT Courses & Classes in Miami, LSAT Courses & Classes in Denver, GRE Courses & Classes in Washington DC, MCAT Courses & Classes in New York City, ISEE Courses & Classes in New York City, SAT Courses & Classes in Philadelphia, GMAT Courses & Classes in Chicago, ACT Courses & Classes in Dallas Fort Worth

Popular Test Prep

LSAT Test Prep in Seattle, SAT Test Prep in Philadelphia, ISEE Test Prep in Denver, GMAT Test Prep in Seattle, MCAT Test Prep in Dallas Fort Worth, SSAT Test Prep in San Diego, ACT Test Prep in Seattle, LSAT Test Prep in Boston, GMAT Test Prep in San Francisco-Bay Area, SAT Test Prep in New York City

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Calculus AB : Calculus AB

Study concepts, example questions & explanations for Calculus AB

All Calculus AB Resources

Example Questions

Example Question #4 : Calculate Rates Of Change And Related Rates

Example Question #5 : Calculate Rates Of Change And Related Rates

Example Question #1 : Calculate Rates Of Change And Related Rates

Example Question #6 : Calculate Rates Of Change And Related Rates

Example Question #1 : Calculate Rates Of Change And Related Rates

Example Question #2 : Calculate Rates Of Change And Related Rates

Example Question #1 : Calculate Rates Of Change And Related Rates

Example Question #2 : Calculate Rates Of Change And Related Rates

Example Question #3 : Calculate Rates Of Change And Related Rates

Example Question #4 : Calculate Rates Of Change And Related Rates

All Calculus AB Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: