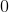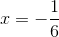All Calculus 1 Resources
Example Questions
Example Question #5 : Area
Find the area bounded by the curve 
The curve is in quadrant one over the interval ![[0,2]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/358442/gif.latex)
The definite integral below is solved by taking the antiderivative of each term of the given polynomial function, evaluating this antiderivative at the bounds of integration, and subtracting the values.
For this particular integral use the rule, 
Example Question #6 : Area
Find the area under the curve between the following bounderies of the following function.


We can find the area under the curve by taking the anti-derivative of the function and using the two boundaries as x values. The anti-derivative of 


Example Question #7 : Area
Evalute the following Definite Integral:
For this integral one may be tempted to directly integrate; however, this is no rule of integrals which allows us to do so.
We must apply a complex method of integration, here u-subtitution works best.
We notice that Cos(x) is the derivative of Sin(x) so it may be best to let 
Now that we have found a proper u and du, we may directly substiute into our original integral
However, our limits are in terms of x so we must substitute our u=Sin(x) back in before evaluating
Example Question #1 : Points
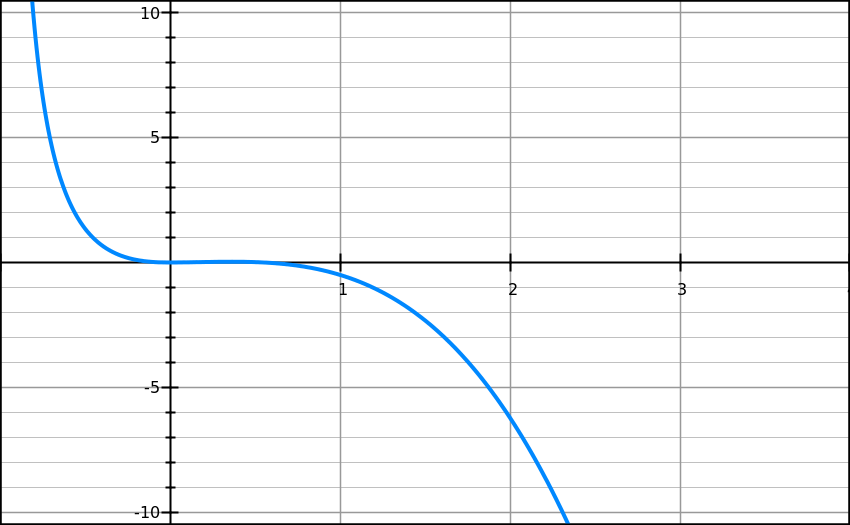
Suppose a point on the curve given above has the property that 
Based solely on the graph above, which of the following is most likely the 
If
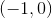

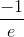

Example Question #1 : Describing Points
What is the critical point for 
To find the critical point, you must find the derivative first. To do that, multiply the exponent by the coefficient in front of the 




Example Question #1 : Points Of Inflection
Find the inflection point(s) of 
Inflection points can only occur when the second derivative is zero or undefined. Here we have

Therefore possible inflection points occur at 


Hence, both are inflection points
Example Question #1 : Points
Below is the graph of 


Not enough information
Possible inflection points occur when 



Example Question #3 : How To Graph Functions Of Points Of Inflection
Find the point(s) of inflection for the function 

There are no points of inflection.

A point of inflection is found where the graph (or image) of a function changes concavity. To find this algebraically, we want to find where the second derivative of the function changes sign, from negative to positive, or vice-versa. So, we find the second derivative of the given function 
The first derivative using the power rule



We then find where this second derivative equals 


We then look to see if the second derivative changes signs at this point. Both graphically and algebraically, we can see that the function 

Example Question #1 : How To Graph Functions Of Points Of Inflection
Find all the points of inflection of

There are no inflection points.
In order to find the points of inflection, we need to find 

Now we set 

To verify this is a true inflection point we need to plug in a value that is less than it and a value that is greater than it into the second derivative. If there is a sign change around the point than it is a true inflection point.
Let
Now let
Since the sign changes from a positive to a negative around the point 
Example Question #4 : How To Graph Functions Of Points Of Inflection
Find all the points of inflection of
There are no points of inflection.
In order to find the points of inflection, we need to find 

Now to find the points of inflection, we need to set 

Now we can use the quadratic equation.
Recall that the quadratic equation is

where a,b,c refer to the coefficients of the equation 
In this case, a=12, b=0, c=-4.
Thus the possible points of infection are

Now to check if or which are inflection points we need to plug in a value higher and lower than each point. If there is a sign change then the point is an inflection point.
To check 

Therefore 
Now lets check 

Therefore 
Certified Tutor
Certified Tutor
All Calculus 1 Resources