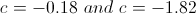To find the inflection points of a function we need to take the second derivative and find which values make it zero.

To find the first and second derivative we will need to apply the power rule, .

Given, 

and applying the power rule we get,

.

Points of inflection happen when the second derivative changes signs.  The quadratic above changes signs at and . 

This can be determined by factoring,

,

or by the quadratic formula,

.

The point of inflection can be found by setting the second derivative equal to 0.

The power rule is given by:

Use the power rule twice to find the second derivative.

Set the second derivative equal to  and solve for .

Find the point of inflection by plugging  back into the original equation.

Therefore, the point of inflection is 

To find where the points of inflection occur, we must find the points at which the second derivative changes sign. 

First, we must find the second derivative:

The derivatives were found using the following rule:

Next, we must find the x values at which the second derivative is equal to zero:

Now, we make the intervals on which we will examine the sign of the second derivative, using the above x value:

Note that at the bounds of the intervals the second derivative is neither positive nor negative.

On the first interval, the second derivative is negative, while on the second, the second derivative is positive. (We check the sign of the second derivative by plugging in any value on the interval into the second derivative function.) A sign change occured at , so this is our answer. 

The point of inflection of an object can be found by setting the second derivative of the function equal to zero and solving. 

First, we need to simplify the expression.  Sine the numerator has more terms than the denominator, we can simply divide out both terms.  

.

Now, we must compute the second derivative of this function, and set it equal to zero. 

.

.

.

To find the points of inflection, we must find the points at which the second derivative of the function changes sign.

First, we must find the second derivative:

The derivatives were found using the following rule:

Next, we must find the x value at which the second derivative is equal to zero:

Using this value, we make our intervals on which we examine the sign of the second derivative:

Note that at the endpoints of the intervals, the second derivative is neither positive nor negative.

Now, simply plug in any value on the interval into the second derivative function and check the sign. On the first interval, the second derivative is negative, while on the second interval, it is positive. Thus, a point of inflection exists at . To get the y-coordinate, simply evaluate the function at this x value:

The point of inflection is .

To determine the points of inflection, we must determine the x values at which the second derivative changes in sign.

So, we must first find the second derivative:

The derivatives were found using the rule

Next, we must find the values at which the second derivative is equal to zero:

Now, using this zero, we can make the intervals on which we check the sign of the second derivative:

Note that at the endpoints, the second derivative is neither positive nor negative.

Plug in any value on each interval into the second derivative function and check the sign. On the first interval, the second derivative is negative, while on the second, it is positive. Because a sign change occured at , there exists the point of inflection.

To determine the points of inflection of the function, we must determine where the second derivative of the function changes sign.

First, we find the second derivative:

The derivatives were found using the following rule:

Now, we must find where the second derivative equals zero:

Using this value, we can now create the intervals on which we check the sign of the second derivative:

Note that at the endpoints of the intervals, the second derivative is neither positive nor negative.

To check the second derivative's sign on each interval, simply plug in any point on the interval into the second derivative function. On the first interval, the second derivative is negative, while on the second interval, it is positive. Because the sign of the second derivative changes at , there exists the point of inflection.

Infelction points are the points of a graph where the concavity of the graph changes.  The inflection points of a graph are found by taking the double derivative of the graph equation, setting it equal to zero, then solving for .  

To take the derivative of this equation, we must use the power rule,

 .  

We also must remember that the derivative of an constant is 0.  

After taking the first derivative of the graph equation using the power rule, the equation becomes

.  

In this problem the double derivative of the graph equation comes out to , factoring this equation out it becomes .  

Solving for when the equation is set equal to zero, the inflection points are located at .

To find the critical points, set  and solve for .

Differentiate:

Set equal to zero:

Solve for  using the quadratic formula: