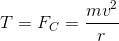All AP Physics C: Mechanics Resources
Example Questions
Example Question #46 : Ap Physics C

A ball of mass 


None of these
This is a centripetal force problem. In this case the tension on the rope is the centripetal force that keeps the ball moving on a circle.
If we want for the rope not to break, then the tension should never exceed 
Now we just solve for velocity:
Example Question #1 : Understanding Linear Rotational Equivalents
What is the rotational equivalent of mass?
Moment of inertia
Angular momentum
Radius
Torque
Moment of inertia
The correct answer is moment of inertia. For linear equations, mass is what resists force and causes lower linear accelerations. Similarly, in rotational equations, moment of inertia resists torque and causes lower angular accelerations.
Example Question #1 : Understanding Linear Rotational Equivalents
In rotational kinematics equations, what quantity is analogous to force in linear kinematics equations?
Angular acceleration
Moment of inertia
Torque
Impulse
Torque
Just as force causes linear acceleration, torque causes angular acceleration. This can be seen most in the linear-rotational comparison of Newton's second law:
Example Question #3 : Understanding Linear Rotational Equivalents
A boot is put in a 

Linear (tangential) velocity, 
Here, 

Solve.
Example Question #1 : Rotational Motion And Torque

A particle is moving at constant speed in a straight line past a fixed point in space, c. How does the angular momentum of the particle about the fixed point in space change as the particle moves from point a to point b?
The particle does not have angular momentum since it is not rotating
It cannot be determined without knowing the mass of the particle
The angular momentum increases
The angular momentum decreases
The angular momentum does not change
The angular momentum does not change
The angular momentum of a particle about a fixed axis is 







Example Question #2 : Circular And Rotational Motion
Doing which of the following would allow you to find the center of mass of an object?
Recording its shape
Sliding it along a flat surface
Spinning it
Hanging it from a fixed point
Spinning it
Center of mass can be found by spinning an object. It will naturally spin around its center of mass, due to the concept of even distribution of mass in relation to the center of mass. Shape and mass are important factors in this property, but the most improtant factor is the mass distribution.
Example Question #1 : Rotational Motion And Torque
If the fulcrum of a balanced scale is shifted to the left, what type of adjustment must be made to rebalance the scale?
Apply more mass to the right end
Apply the same amount of mass to both ends
Apply more mass to the new position of the fulcrum
Apply more mass to the left end
If the scale was initially balanced, moving the fulcrum will not change this
Apply more mass to the left end
Changing the position of the fulcrum by moving it to the left means the center of mass will be to the right of the new position. Therefore, the scale will tip right. Adding more mass to the left end will rebalance the scale. None of the other options make sense. Adding more mass to the new fulcrum position will not change the balance of the scale because that mass is a negligible distance from the new fulcrum position and does nothing to change the masses on either side.
Example Question #54 : Ap Physics C
If two masses, 


This question asks us to find the center of mass for this system. We know that the center of mass resides a distance 

In this case:
Plug in known values and solve.
Example Question #55 : Ap Physics C
Three 





How far from the origin is the center of mass of the system?
To find the center of mass, we have to take the weighted average of the x coordinates and the y coordinates.



First, we take the weighted measurement of the x-axis:
We can see that the result of the x-axis contribution is equal to 
Now, let's look at the y-axis contribution:
This equals to
Now that we have the x and y components, we take the root of squares to get the final answer:
This will give us
Example Question #1 : Circular And Rotational Motion
An object starts from rest and accelerates to an angular velocity of 

Since it is experiencing a constant torque and constant angular acceleration, the angular displacement can be calculated using:
The angular acceleration is easily calculated using the angular velocity and the time:
Using this value, we can find the angular displacement:
Convert the angular displacement to revolutions by diving by 
All AP Physics C: Mechanics Resources