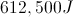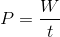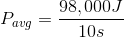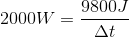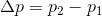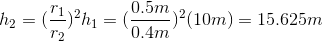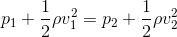All AP Physics B Resources
Example Questions
Example Question #21 : Ap Physics 1
A 


First, we calculate the initial kinetic energy of the car:
Then, calculate the final kinetic energy after the car has reached a velocity of 
To find the change in kinetic energy, we subtract the initial energy from the final energy.
This answer in positive because the car gains energy when it increases its velocity.
Example Question #1 : Understanding Kinetic Energy
A 

The first thing we need to find is the final velocity of the car. We know it starts from rest and has an acceleration of 
The formula for kinetic energy is:
We can use the calculated final velocity and the mass of the car to determine its final kinetic energy.
Example Question #61 : Newtonian Mechanics
A uniform thin hoop of mass 


Note that the moment of inertia of a uniform thin hoop of mass 


The total kinetic energy for an object rolling without slipping is the sum of its translational and rotational kinetic energies.
The translational kinetic energy treats the object as if it were a mass sliding along at a constant velocity. We can calculate the translational kinetic energy using the equation:
Use this equation with the given mass and velocity to solve for translational kinetic energy.
The rotational kinetic energy is given by the formula:
In this equation, 

The relationship between angular and linear velocity in the case of a hoop rolling without slipping is:
Solve for the moment of inertia and the angular velocity of the hoop.
Use these values in the formula for rotational kinetic energy.
The total kinetic energy is the sum of the translational and rotational kinetic energies.
Example Question #61 : Ap Physics B
A crane lifts a 


To find average power, we find the total work done by the crane in lifting the steel beam, and then divide it by the time taken to complete the task.
In this case, the total work done is equal to the change in potential energy of the steel beam. for this, we use the potential energy equation:
We then divide the work by 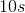
Example Question #4 : Power
A crane is being used to lift a 


First we find the total work required to lift the crate:
We are given the mass, change in height, and the acceleration of gravity. Using these values, we can solve for the total work done.
Next, we can use the equation for power to find the time to complete this task if the engine is working at its maximum power.
Example Question #1 : Understanding Conservation Of Momentum
A baseball pitcher throws a baseball at 


In order to calculate the change in momentum, we must find the initial and final momentum of the baseball, and then find the difference.
Use the given velocities and mass to calculate the initial and final values.
The initial momentum is positive because the problem states that the ball was originally thrown in the positive direction. The final momentum is negative due to the change in direction.
Now we find the change in momentum:
Example Question #51 : Mcat Physical Sciences
Ball A, traveling 

A perfectly inelastic collision is when the two bodies stick together at the end. At the beginning the two balls are traveling separately with individual momentum values. Using the momentum equation 
Note: ball B's velocity is negative because they are traveling in opposite directions.
The negative sign indicates the direction in which the two balls are traveling. Since the sign is negative and we indicated that traveling to the left is negative, the two balls must be traveling 2m/s to the left after the perfectly inelastic collision.
Example Question #1 : Momentum
Two astronauts, Ann and Bob, conduct a collision experiment in a weightless, frictionless environment. Initially Ann moves to the right with a momentum of 
to the left. What is Bob's final momentum?

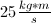




Apply conservation of momentum before and after the collision.

Taking left to be the negative direction, and noting that Bob's initial momentum is 0 since he is at rest, we can use the provided information to see that 
Solving for 

Example Question #2 : Momentum
A 










This is a completely inelastic collision, and, as with all collisions, it conserves linear momentum. To get magnitude and direction in two dimensions, it is typically best to look at the horizontal and vertical directions individually.
Horizontal direction: The 

The 

So the total x-momentum after the collision is the sum of these two initial x-momenta, since total momentum is conserved in the collision.
This immediately shows that the combined mass after the collision is moving in the positive x-direction.
We now need to compute the final velocity in the x-direction. In this case:


Vertical direction: The same procedure can be applied to the y-direction. In this case, the process is simpler, because only the 

Since the final momentum is now known to be positive in both the horizontal and vertical directions, the angle with respect to the x-axis must be positive. Now, the final y-direction velocity can be calculated.
Final velocity: With the final velocity components known, the final velocity can be calculated from trigonometry.
Magnitude:
Direction:
Example Question #1 : Fluid Flow
An incompressible fluid flows without viscosity at 

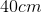



The mass density of the fluid is 
If the fluid is incompressible and flows without viscosity in a confined space, then an equal volume must move past any point in that space in the same time interval, even if the geometry of the confines changes. An equal volume must pass Points 


Since the diameter of the section on the right is smaller, the fluid must move faster in that region for an equal volume to pass by point 
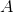
In this problem, the flow speed in the larger section of pipe is 

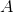


Hence, the flow speed in the smaller section of pipe is 
Now use Bernoulli’s principle, which expresses conservation of energy in fluid flow as:
Pressure + kinetic energy of flow + fluid potential energy = constant
Fluid potential energy depends on height (like gravitational potential energy), and so does not change from one part of the pipe to the other. So, for this case, Bernoulli's principle becomes:





All AP Physics B Resources