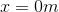The relationship between the number of turns for the primary coil and secondary coil in a transformer ( and  respectively) to the relative potentials is

Solving for ,

Magnetism is caused by electrons in a material aligning and causing an aggregate magnetic field that can exert influence over other things. The magnetic strength is limited by random fluctuations in the electrons, making them no longer aligned. If the magnet were to be cooled, the electrons would have less kinetic energy, and would be less likely to have random fluctuations. This would make the strength increase.

All of the other things would make the strength of a magnet decrease. Striking it would impart kinetic energy, and would momentarily vibrate the electrons. Likewise, heating it would make the electrons vibrate more.

In this question, we're told that the velocity filter is able to detect the speed of a particle when the magnetic force and electric force are equal to each other. In order to determine when the particle's velocity will be the greatest, we'll need to keep in mind the equations for both the electric force and magnetic force:

Next, we'll need to set them equal to each other:

Then, we can isolate the velocity term:

Based on this equation, we see that if we want to increase the particle's velocity, we'll need to increase the electric field. Increasing the particle's charge will have no effect on the particle's velocity, and increasing the magnetic field will actually decrease the particle's velocity.

The magnetic field around permanent magnets is caused by the alignment of the material's electrons, which no longer average out to a net field of zero and instead combine to form a greater field. If the electrons come out of alignment, then the field wanes or stops altogether. Additionally, the random vibrations of the electrons inhibits the effectiveness of the field. Therefore, if you were to apply energy to a magnet, it would be less effective because the electrons would have greater random motion. Heating the magnet applies thermal energy to it, and dropping it applies kinetic energy to it, which means neither of them would be the right answer. When you cool a magnet, you are removing some of the energy it has, making the electrons have less random motion.

A diamagnet is a material that, when it is exposed to a magnetic field, produces a magnetic field in the opposite direction as the external field, which leaves an overall lesser field.

The equation for an electric field from a point charge is

To find the point where the electric field is 0, we set the equations for both charges equal to each other, because that's where they'll cancel each other out. Let  be the point's location. The radius for the first charge would be , and the radius for the second would be .

Therefore, the only point where the electric field is zero is at , or 1.34m.

To find where the electric field is 0, we take the electric field for each point charge and set them equal to each other, because that's when they'll cancel each other out.

The 's can cancel out. 

Therefore, the electric field is 0 at .

The equation for force experienced by two point charges is

We're trying to find , so we rearrange the equation to solve for it.

Now, we can plug in our numbers.

Therefore, the strength of the second charge is .

The force between two point charges is shown in the formula below:

, where  and  are the magnitudes of the point charges,  is the distance between them, and  is a constant in this case equal to 

Plugging in the numbers into this equation gives us

We are given a situation in which we have a frame containing an electric field lying flat on its side. In this frame, a positively charged particle is traveling through an electric field that is oriented such that the positively charged terminal is on the opposite side of where the particle starts from. We are being asked to find the horizontal distance that this particle will travel while in the electric field. Since this frame is lying on its side, the orientation of the electric field is perpendicular to gravity. Therefore, the only force we need concern ourselves with in this situation is the electric force - we can neglect gravity. However, it's useful if we consider the positive y-direction as going towards the positive terminal, and the negative y-direction as going towards the negative terminal. It's also important to realize that any acceleration that is occurring only happens in the y-direction. That is to say, there is no acceleration in the x-direction. We'll start by using the following equation:

We'll need to find the x-component of velocity.

Our next challenge is to find an expression for the time variable. To do this, we'll need to consider the motion of the particle in the y-direction. Also, since the acceleration in the y-direction is constant (due to a constant electric field), we can utilize the kinematic equations.

And since the displacement in the y-direction won't change, we can set it equal to zero.

Just as we did for the x-direction, we'll need to consider the y-component velocity.

We also need to find an alternative expression for the acceleration term. We can do this by noting that the electric force is providing the acceleration.

Also, it's important to remember our sign conventions. Since the electric field is pointing from the positive terminal (positive y-direction) to the negative terminal (which we defined as the negative y-direction) the electric field is negative.

Now, plug this expression into the above kinematic equation.

Rearrange and solve for time.

Now that we've found an expression for time, we can at last plug this value into our expression for horizontal distance.

And lastly, use the trigonometric identity:

We are given a situation in which we have a frame containing an electric field lying flat on its side. In this frame, a positively charged particle is traveling through an electric field that is oriented such that the positively charged terminal is on the opposite side of where the particle starts from. We are being asked to find an expression for the amount of time that the particle remains in this field. Since this frame is lying on its side, the orientation of the electric field is perpendicular to gravity. Therefore, the only force we need concern ourselves with in this situation is the electric force - we can neglect gravity. However, it's useful if we consider the positive y-direction as going towards the positive terminal, and the negative y-direction as going towards the negative terminal. To begin with, we'll need an expression for the y-component of the particle's velocity.

Next, we'll need to make use of one of the kinematic equations (we can do this because acceleration is constant).

Since the particle will not experience a change in its y-position, we can set the displacement in the y-direction equal to zero.

At this point, we need to find an expression for the acceleration term in the above equation. The only force on the particle during its journey is the electric force.

It's also important for us to remember sign conventions, as was mentioned above. Since the electric field is pointing towards the negative terminal (negative y-direction) is will be assigned a negative value.

Now, plug this expression for acceleration into the previous expression we derived from the kinematic equation, we find:

Cancel negatives and expand the expression for the y-component of velocity, so we are left with:

Rearrange to solve for time.