All AP Physics 1 Resources
Example Questions
Example Question #1 : Tension
Consider the following system:
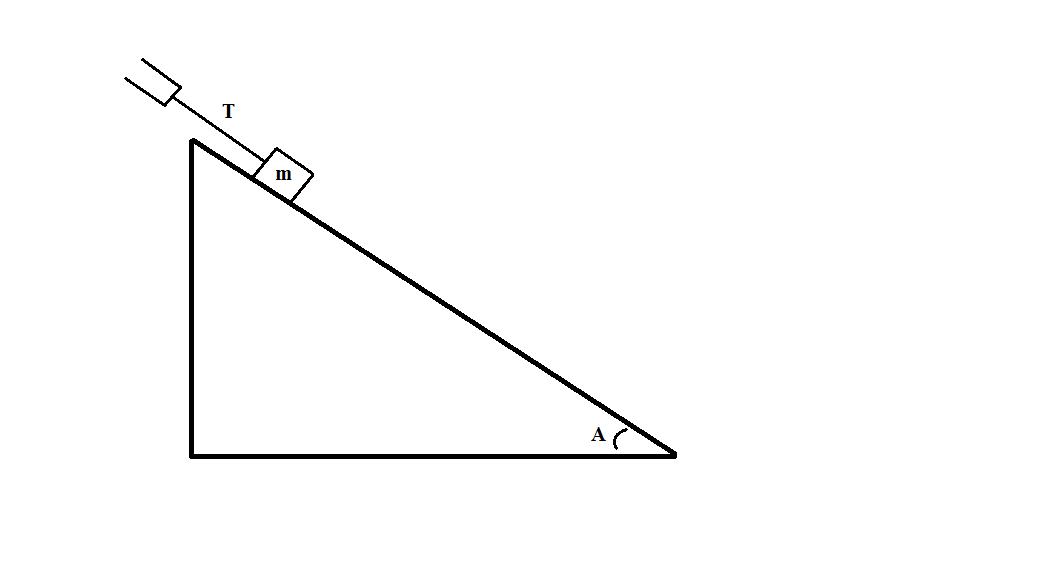
If the mass is accelerating at a rate of 




There are three relevant forces acting on the block in this situtation: friction, gravity, and tension. We can use Newton's second law to express the system:
Substituting expressions in for the forces, we get:
Canceling out mass and rearranging to solve for tension, we get:
We have values for each variable, allowing us to solve:
Example Question #1 : Tension

A 12kg block is sliding down a 

In order to find the mass of block 2, we're going to need to calculate a few other things, such as the tension in the rope.
To begin with, we'll need to identify the various forces on our free-body diagram. To do this, we will begin with block 1 and use a rotated coordinate system to simplify things. In such a system, the x-axis will run parallel to the surface of the ramp, while the y-axis will be perpendicular to the ramp's surface, as shown below:


Now we can identify the forces acting on block 1. Along the rotated y-axis, the force of gravity acting on the block is equal to 

Now, considering the forces acting along the rotated x-axis, we have a force pointing downwards equal to 


The formula for calculating the force due to kinetic friction is:
Since we have already determined what the normal force is, we can substitute that expression into the above equation to obtain:
Now, we can write an expression for the net force acting upon block 1 in the x direction:
Rearrange the above expression to solve for tension.
So far, we have only been looking at block 1. Now let's turn our attention to block 2 and see what forces are acting on it. In the downward direction we have the weight of the block due to gravity, which is equal to 

Since we calculated the expression for tension from the information regarding block 1, we can plug that expression into the above equation in order to obtain:
Now rearrange to solve for the mass of block 2.
Then plugging in values, we can finally calculate block 2's mass:
Example Question #191 : Forces
What is the tension force on a wire holding a 10kg ball 20ft above the ground, if the ball is not moving at that height?
Since the gravitational force must be cancelled by the tension force, as the ball is experiencing no acceleration, and no other forces are being applied to it:
Example Question #1 : Tension
A block weighing 

The block has three forces on it: the force of tension, the force of gravity, and the force from Bruce. The force of gravity is:
The force from Bruce plus the force of tension has to equal gravity (since Bruce's force and tension are up while gravity is down) so the block is in equilibrium.
Example Question #10 : Tension
A helicopter is lifting a box of mass 

Plug in values:
Solve for
Example Question #761 : Newtonian Mechanics
Earth mass:
Earth's distance from the Sun:
Velocity of the Earth:
Imagine that, instead of gravity, the earth was attached to the sun with a giant, unbreakable rope. Determine what the tension would be in the rope.
The velocity of the earth is
Convert to 
Plug in values:
Example Question #761 : Newtonian Mechanics
An elevator accelerates upward for a short period of time at a rate of 

During the period when the elevator is accelerating, what is the magnitude of the tension in the rope?
None of the other answers

Here is a (simplistic) diagram of the elevator. There are three forces acting on this object. There is it's own weight due to gravity 




Since we want the magnitude of the tension, and not the value of the vector, we omit the minus sign. Therefore the tension in the rope is 
Example Question #201 : Specific Forces
Suppose that a 

For this question, we're presented with a scenario in which an object of a given mass is hanging to a rope. That rope is being pulled on, causing the object to accelerate upwards at a certain rate. We're then asked to calculate the tension that results in the rope.
In order to answer this question, it's best if we approach this by examining a force diagram. Since there are no pertinent forces occurring in the x direction, the only concern we have is in the y direction.
One of the forces acting on the object is the downward force of gravity. Another force is the upward tension caused by the rope. Hence, we know what force components contribute to the net force. Furthermore, we're told that as it is pulled up, the object accelerates at a rate of 
Next, we can rearrange the above terms in order to isolate the term for tension.
Finally, if we plug in the values that we know, we can calculate our answer.
Example Question #201 : Specific Forces
Two objects of equivalent mass are attached with a very strong rope that goes through a pulley. The masses are left to hang. What will happen?
Both masses will fall.
One mass will fall and the other will rise.
None of these
The masses will remain motionless.
Both masses will rise.
The masses will remain motionless.
The masses will each provide an equal force to each other through the rope. Thus, there will be no net force and no net acceleration.
Example Question #202 : Specific Forces
Four ropes are used to lift a 

Using superposition of forces and definition of force:
All four ropes will be providing the same force.
Combining equations
Solving for 
Plugging in values, remembering that gravity is pointing down and thus will be a negative number.
All AP Physics 1 Resources
























![m_{2}a=[m_{1}gsin(\theta )-\mu _{k}m_{1}gcos(\theta )-m_{1}a]-m_{2}g](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/454498/gif.latex)

![m_{2}=\frac{[m_{1}gsin(\theta )-\mu _{k}m_{1}gcos(\theta )-m_{1}a]}{(a+g)}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/454500/gif.latex)
![m_{2}=\frac{[(12kg*9.8\frac{m}{s^{2}}* sin(30^{o}))-(0.18* 12kg* 9.8\frac{m}{s^{2}}* cos(30^{o}))-(12kg* 1.5\: \frac{m}{s^{2}})]}{(1.5 \frac{m}{s^{2}}+9.8 \frac{m}{s^{2}})}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/454501/gif.latex)





































































