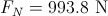AP Physics 1 : AP Physics 1
Study concepts, example questions & explanations for AP Physics 1
All AP Physics 1 Resources
Example Questions
Example Question #11 : Ap Physics 1
A yo-yo trick called Around the World involves swinging the yo-yo around in a vertical circle at the end of its string. A man performs this trick with a 
Centripetal force can be found using the equation:
In this situation, the radius is the length of the string. We are given the linear velocity and mass, as well as the radius, allowing us to solve for the centripetal force.
Example Question #1 : Using Torque Equations
A 
This problem requires us to add torques about the pivot point. In order for the seesaw to be balanced, the torque must be equal on each side of the pivot point.
Use the equation for torque in this equation.
The force of each object will be equal to the force of gravity.
Gravity can be canceled from each side of the equation. for simplicity.
Now we can use the mass of the rock and the mass of the child. The total length of the seesaw is two meters, and the child sits at one end. The child's distance from the center of the seesaw will be one meter.
Solve for the distance between the rock and the center of the seesaw.
Example Question #41 : Translational Motion
A 2kg box is at the top of a frictionless ramp at an angle of 60o. The top of the ramp is 30m above the ground. The box is sitting still while at the top of the ramp, and is then released.
If the angle of the ramp is decreased, which of the following statements is false?
The net force on the box will decrease
The normal force will decrease
The time to reach the ground will increase
The force due to gravity will decrease
The normal force will decrease
When given a question about the angle of a ramp, compare it to the extreme angles: 0o and 90o.
1. When the ramp has an angle of 0o, the net force 0. The force due to gravity must equal the normal force; thus the normal force is at a maximum value.
2. When the angle of the ramp is 90o, the full force of gravity is experienced by the box, and there is no normal force. The net force is equal to the force of gravity. Remember that 
When we decrease the angle of the ramp, we get closer to scenario 1. As a result, we can conclude that the normal force on the box increases, rather than decreases.
Example Question #1 : Understanding Normal Force
A woman is standing on a scale in an elevator as it accelerates upward. The elevator then stops accelerating and continues upward at a constant speed. Which of the following statements is true?
According to the scale, the woman weighed more when the elevator was moving at a constant speed
According to the scale, the woman weighed more when the elevator was accelerating
According to the scale, the woman weighed less when the elevator was accelerating
According to the scale, the woman's weight did not change throughout the motion
We must know the woman's mass to draw any conclusions
According to the scale, the woman weighed more when the elevator was accelerating
The normal force of the woman is measured by the scale. While accelerating upward, the scale should read a larger weight than when it is at rest. When the elevator is moving upward at a constant speed, the scale should read the same as when it is at rest. This is because the normal force is generated to counter the downward forces pushing against the floor. When the elevator is accelerating, there is a net upward force from the acceleration as well as the normal force to counter gravity. The normal force generates an upward acceleration. When moving at a constant speed, there is no upward acceleration and the normal force acts only to counter gravity. The normal force, and scale reading, will thus be greater during the period of acceleration.
Example Question #11 : Ap Physics 1
A 
To find the normal force on the incline, we use the relationship:
This provides the magnitude of the force of gravity in the direction perpendicular to the incline. Normal force will always act in the direction perpendicular to the surface, and in this case will be equal and opposite to the force of gravity. We then plug in the mass and gravitational acceleration to find the normal force on this block:
Example Question #1 : Understanding Normal Force
Five substitute players on a basketball team are sitting on the bench during a game. The bench weighs 
The normal force is generated as a result of a force against a solid surface. As per Newton's third law, the surface will exert an equal and opposite force on the object in contact. If an object is resting on a flat surface, then the normal force will be working to counter the weight of the object due to gravity.
The normal force acting on the bench with five players is equal and opposite to the total weight of the bench and players. Keep in mind that weight acts in the downward direction.
When the two players stand up, the new normal force is reduced.
The difference in the normal force is:
We could also have found this change by adding the weights of the two players who stood.
Example Question #1 : Understanding Friction
A 
We know that normal force on a flat surface is equal and opposite the force of gravity.
Then, find the friction force between the crate and the floor using the equation:
This force is the minimum force required to start moving the crate.
Example Question #11 : Forces
A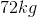 
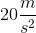
This question essentially asks how much the astronaut will weigh during acceleration. The two forces acting on the astronaut are the downward gravitational force and the upward normal force. The net force acting on this astronaut is 
Notice that the net force is set to equal to 
Isolate the normal force.
Use the given values to solve.
Example Question #13 : Ap Physics 1
A potted plant is hanging from a rope attached to a hook. The plant exerts 25N of force. Assume the rope is weightless.
If the packaging says the rope is able to withstand 800N of force, what is the maximum amount of mass that can be hanging from the rope?
If the rope can only withstand 800N of force, we can solve for the mass of the object which would result in this maximum force when hanging from the rope.
Example Question #1 : Understanding Newton's Laws
A skydiver with a mass of 100kg jumps out of an airplane and reaches a terminal velocity of 
Which of the following factors will not decrease the total force on the man as he jumps from the plane?
The man jumps as the plane is descending sharply
The man immediately opens his parachute
Increasing the man's mass
The man orients his stomach toward the ground, rather than his feet
Increasing the man's mass
The equation for force is 
All other options will result in a decreased acceleration when falling. Falling with his stomach downward increases the surface area of the diver, which increases his air resistance. A parachute will also increase the air resistance and surface area. If the plane is descending when he jumps, his time to reach terminal velocity will be reduced, as he will already have an initial velocity in the downward direction.
Certified Tutor
All AP Physics 1 Resources
