All AP Physics 1 Resources
Example Questions
Example Question #185 : Specific Forces
Two springs with unknown constants are hanging independently from a ceiling. However, we do know that one spring has a constant three times that of the other. A block of mass 

Much like resistors in a circuit, spring constants can be added together to get one equivalent constant. Since these springs are added in parallel, we can simply add their constants together:
Furthermore, we know that:
Substituting this in, we get:
Now we can use Hooke's Law to determine what this equivalent resistance is:
Rearranging for the equivalent constant:
Example Question #41 : Spring Force
Two springs are attached independently from a ceiling with the constant of one being 


Much like resistors in a circuit, spring constants can be combined to obtain a single equivalent constant. When springs are in parallel, we simply add their constants, and when they are added linearly, we add their inverses. Just like a circuit, let's begin with the two springs in parallel:
Now we can combine this equivalent constant with the third spring:
Example Question #187 : Specific Forces
Two springs with unknown constants are hung in parallel from a ceiling. However, we do know that one spring has a constant 



Since the springs are held in constant, we can determine a total equivalent constant by simply adding the two individual constants together:
From the problem statement, we know:
Substituting this in, we get:
The problem statement tells us that a mass is attached and the springs stretch an unknown distance. At this point, the system has reached a new equilibrium. The problem statement tells us that another mass is attached and the springs stretch more. Since spring forces are linear, the new mass and distance stretched is the only information we need to solve the problem. Using Hooke's Law:
Rearranging for the spring constant:
Plugging in our values:
Example Question #751 : Newtonian Mechanics
Two springs with unknown constants are hung from a ceiling. However, we do know that the constant of one is four times that of the other. A block of mass 


Since the springs are added in parallel, we can simply add their constants together to get an equivalent constant.
From the statement, we know:
Substituting this in, we get:
Now we can use Hooke's Law along with the information of how far the block causes the springs to stretch:
Rearranging for the equivalent constant:
Plugging in our values:
Now we can use the expression for the potential energy stored in a spring:
The problem statement is asking for the total potential energy stored, so we need to use the total distance stretched (which includes both from the mass and manually). Plugging in our values, we get:
Example Question #791 : Ap Physics 1
A force of 

The force applied to a spring can be represented by
where F is force, k is spring constant, and x is the distance compressed.
Solving this equation for k
and plugging in our values
Example Question #1 : Tension
A 10kg block is suspended by two ropes. Each rope makes an angle of 45 degrees to the horizontal.
What is the magnitude of the tension force in each rope?
Luckily enough, the angles of the two ropes are the same. Therefore, the tension in each will be the same. This immediately eliminates two of the five answers. Now we just need to calculate what that force is.
We know that together, the vertical components of the tension must equal the weight of the block. Therefore we can write:
Since we know that the two tension forces are equal, we can rewrite:
Rearranging for T, we get:
Example Question #1 : Tension
Consider the following system:
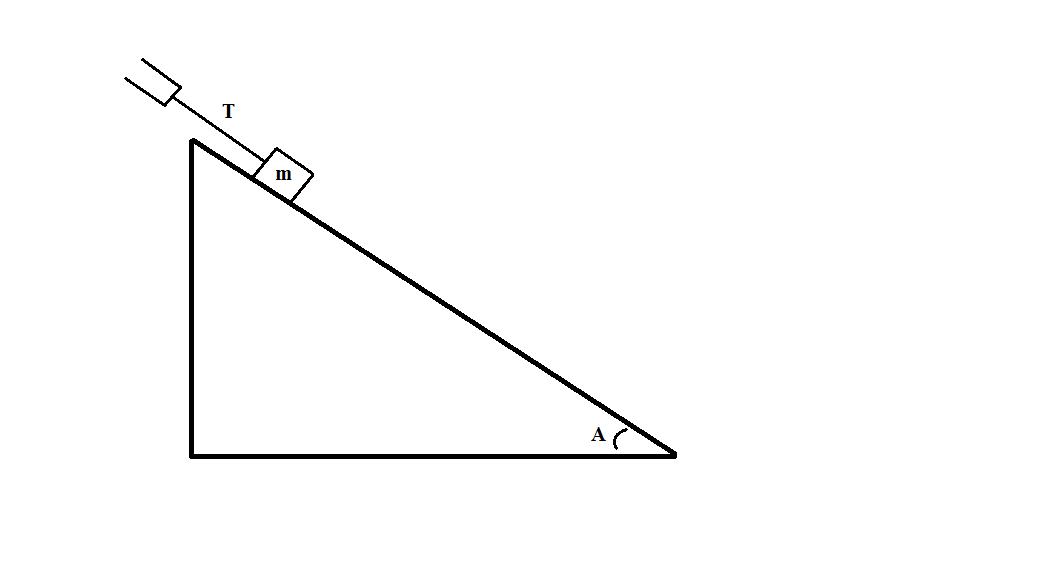
If the mass is 


Since there is no friction between the mass and slope, there are only two relevant forces acting on the mass: gravity and tension. Furthermore, since the block is not in motion, we know that these forces are equal to each other. Therefore:
Substituting in an expression for the force of gravity, we get:
We know all of these values, allowing us to solve for the tension:
Example Question #792 : Ap Physics 1
Consider the following system:

If the force of tension is 



There are three relevant forces acting on the block in this scenario: friction, tension, and gravity. We are given two of these values, so we simply need to develop an expression for the force of gravity in the direction of the slope. Since the block is motionless, we can write:
Substituting in an expression for the force of gravity, we get:
Rearrange to solve for the angle:
We know all of these values, allowing us to solve:
Example Question #1 : Tension
Consider the following system:

If the coefficient of static friction is 


There are three relevant forces acting on the block in this scenario: tension, friction, and gravity. We are given tension, so we will need to develop expressions for friction and gravity. Since the block is motionless, we can say:
Plugging in expressions for the force of gravity and friction, we get:
Rearranging for the mass, we get:
We know all of these values, allowing us to solve:
Example Question #793 : Ap Physics 1
Consider the following system:

If the block has a mass of 


Since there is no tension, there are only two relevant forces acting on the block: friction and gravity. Since the block is motionless, we can also write:
Substitute the expressions for these two forces:
Canceling out mass and gravitational acceleration, and rearranging for the coefficient of static friction, we get:
All AP Physics 1 Resources
































































































































