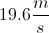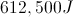The man has reached a terminal velocity, which means that he has an acceleration of   Because , the total force on the skydiver is 0N. At this point, the force due to gravity in the downward direction is equal to the force of air resistance in the upward direction.

According to Newton's third law, the force on the crate must have the same magnitude as the force on the man. The forces must also be in opposite directions. We can conclude that the net force on the man is zero, since he is moving with the crate at a constant velocity.

We could approach this problem by using one of the kinematics equations but we should use conservation of energy to solve. Conservation of energy states that the initial total energy of a system is equal to its final total energy. In our case, the system is a rock with an initial speed of . Choosing the instant at which the rock was thrown at zero height, the rock has only has kinetic energy initially.

At maximum height, the velocity is zero (no kinetic energy) and the ball will have only potential energy.

By conservation of energy, we know that the initial and final energies are equal.

Using this, we can solve for the height.

This question can be solved using either conservation of energy or linear motion equations.

The ball reaches maximum height when all of the initial kinetic energy is converted to potential energy. From this, we use the relationship:

Mass cancels out from the equation, and we can isolate the velocity variable.

We are given the final height and the acceleration from gravity, allowing us to find the initial velocity.

We can use conservation of energy to solve this problem. The rock initially has a potential energy, but no kinetic energy. When the rock reaches the ground, it has no potential energy and all kinetic energy. By conservation of energy:

Substitute the equations for potential and kinetic energy.

We can cancel the mass from each term, and isolate the final velocity variable.

Finally, use the given value for the height and the acceleration of gravity to solve for the final velocity.

The bowl described is, fundamentally, a perpetual motion device. When a ball is rolled down the side of a bowl it will gather kinetic energy. As it rolls up the opposite side, this kinetic energy is converted to potential energy until the ball reaches a maximum height. At this point, potential is converted back to kinetic energy. This process would repeat infinitely as long as no energy is lost to the outside environment.

In reality, this machine cannot exist. While a frictionless surface would prevent loss of energy as heat and the vacuum would prevent air resistance, these conditions cannot be met in the real world.

In the given scenario, however, total energy never decreases. The maximum potential energy will always be the same, meaning that the ball will always reach the same maximum height, and the maximum kinetic energy will always be the same, meaning that the ball will always reach the same maximum velocity. Since no energy is lost, both kinetic and potential energy will constantly change in relation to one another, but total mechanical energy will remain forever constant.

This question can be solved by using conservation of energy. Initially, the ball has a displacement of zero, but a non-zero initial velocity. We can say that the initial energy is equal to the total kinetic energy.

At the peak height of the trajectory, the velocity will be zero and the ball will have a maximum displacement. We can say that the energy at this point is equal to the total potential energy.

Finally, when the ball lands, its displacement will again be zero and it will have accelerated (due to gravity) to regain the same magnitude of velocity as the initial throw.

Due to conservation of energy, these terms are all equal. As potential energy increases, kinetic energy decreases, and vice versa. To solve this question, we need to know when the potential energy is equal to half of the total energy.

At exactly half the height, the potential energy will comprise exactly half the total energy. By necessity, the other half will be kinetic energy and the two values will be equal.

The time to travel half the height will be equal to the full flight time divided by four, since acceleration remains constant. The ball will be at this height twice during its trajectory: when it is traveling upward and when it is traveling down. The second time point will be equal to three times this interval.

At these two times, the kinetic energy and potential energy will have equal values.

We can use work and energy to solve this problem. First, solve for the initial kinetic energy of the shopping cart, using its initial velocity:

The final kinetic energy will be zero, since the cart will come to a complete stop. Work is equal to the change in mechanical energy (in this case kinetic energy).

Work can also be written as the product of a force acting over a distance. In this case, the constant force is friction.

Use the calculated value of work and the force of friction to solve for the distance. Remember that force of friction will be negative since it acts in the opposite direction of the motion.

We know that  and . First, calculate the translational velocity by using the Pythagorean Theorem.

We can use this to find the total kinetic energy.

First, we calculate the initial kinetic energy of the car:

Then, calculate the final kinetic energy after the car has reached a velocity of :

To find the change in kinetic energy, we subtract the initial energy from the final energy.

This answer in positive because the car gains energy when it increases its velocity.