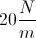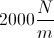All AP Physics 1 Resources
Example Questions
Example Question #1 : Ap Physics 1
A ball is thrown at a velocity of 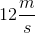


There is not enough information to solve this problem
The velocity of 

Use the total velocity, the x-component, and the y-component to form a right triangle below.

Treating 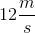
Plugging in the given values, we can solve for the x and y velocity components.
Example Question #1 : Newtonian Mechanics
If a 15kg object is subjected to a force of 175N to the east and another force of 1020N to the west, what is the object’s acceleration?
56m/s2
113m/s2
1.4m/s2
5.6m/s2
56m/s2
First, find the net force by subtracting the opposing forces.
F = 1020N – 175N = 845 N to the west
Next, find acceleration using Newton's second law, 
Example Question #1 : Ap Physics 1
Three blocks (in left-to-right order: block A, block B, block C) are pushed along a frictionless horizontal surface by a 50N force to the right, which acts on block A.
If the masses of the blocks are given by 


The three blocks must remain in contact as they move, so they will each have the same velocity and acceleration regardless of their different masses. So, 
Example Question #2 : Ap Physics 1
A 



Know what forces are involved by drawing a force diagram.

The gravitational force is broken into the x and y components. The net force on the block in the y-direction is the normal force minus the y component of the gravitational acceleration (
Notice that the net y-force is equal to zero to show that the block is not moving anywhere in the y-direction. Now, we can isolate the normal force.
The net force in the x-direction is 

We can use the friction equations to substitute for the x-direction forces.
We can isolate the acceleration and solve using the provided values.
Example Question #3 : Ap Physics 1
A car of mass 



The first step will be to find the final velocity of the car. We know the acceleration and time, so we can find the final velocity using kinematics. The initial velocity is zero, since the car starts at rest.
Use this velocity and the mass of the car to solve for the final kinetic energy.
Example Question #1 : Ap Physics 1
A man throws a 


We first need to find the net force acting on the ball during flight. We can then use the net force and Newton's second law to find the total acceleration on the ball.
Use this net force to find the acceleration.
From here, there are two ways to solve. One way uses kinematic equations, and the other uses energy. We will solve using energy.
Total energy must be conserved during the throw, so the initial kinetic energy must equal the final potential energy (since velocity is zero at the maximum height).
Use the given initial velocity to find the final height.
Example Question #1 : Newton's Second Law

What is the acceleration of the system shown above? (Assume the table is frictionless and the mass of the rope connecting blocks is negligible).
The force that translates to the entire system is that of gravity acting on the mass hanging over the ledge.
140N is the total force acting on the system, which has a mass equal to both blocks combined (65 kg + 14 kg = 79 kg). We can find the acceleration using Newton's second law.
Example Question #1 : Ap Physics 1
A spring is compressed 

We use Hooke's law equation to relate the force, displacement, and spring constant:
We are given the force and the displacement, allowing us to solve for the spring constant.
Note that the displacement is negative, since the spring is compressed. For springs, compressions represents a negative displacement, while stretching represents a positive displacement.
Example Question #2 : Ap Physics 1
A mass of weight 

First, we need to solve for the spring constant by using the force on the spring. We can use Hooke's Law:
The magnitude of the force on the spring will be equal to the force of gravity on the mass, which is given to be 
Next, use the spring energy equation with the displacement and spring constant to solve for the energy stored in the spring.
Example Question #1 : Ap Physics 1
A ball of mass 


We use the equation for centripetal force to find the radius:
Since the string ties the ball to the axis, the force of tension will be equal to the centripetal force.
Use the given mass and velocity to solve for the radius.
All AP Physics 1 Resources