All AP Calculus AB Resources
Example Questions
Example Question #1 : Continunity As A Property Of Functions










Example Question #2 : Geometric Understanding Of Graphs Of Continuous Functions

Consider the graph of above. What can we say about
when

Two or more of these are correct.





None of these are correct.
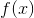

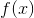

Note that 


The other two are incorrect because sharp turns only apply when we want to take the derivative of something. The derivative of a function at a sharp turn is undefined, meaning the graph of the derivative will be discontinuous at the sharp turn. (To see why, ask yourself if the slope at 
In this case, to get from 

Example Question #1 : Asymptotic And Unbounded Behavior
Where are the vertical asymptotes on the graph of the function
To solve this, find where the function cannot exist. Here, the function cannot exist if the denominator is zero. This happens at x=2 and x=-2. Graph the function on a graphing calculator or by hand to see that the function never crosses these vertical lines. It only gets infinitely close. This is characteristic of vertical asymptotes.
Example Question #1 : Asymptotic Behavior In Terms Of Limits Involving Infinity
Find all vertical asymptotes and horizontal asymptotes of the function,
The horizontal asymptote is,
The vertical asymptotes are,
Horizontal Aysmptotes
Vertical Asymptotes
Horizontal Aysmptotes
Vertical Asymptotes
The horizontal asymptote is,
The vertical asymptotes are,
Horizontal Aysmptotes
None
Vertical Asymptotes
The horizontal asymptote is,
The vertical asymptotes are,
1) To find the horizontal asymptotes, find the limit of the function as 
Therefore, the function 
________________________________________________________________
2) Vertical asympototes will occur at points where the function blows up, 
Factor the denominator and set to zero,
So the graph of 




Example Question #3 : Asymptotic And Unbounded Behavior
Undefined.
For this infinity limit, we need to consider the leading terms of both the numerator and the denominator. In our problem, the leading term of the numerator is larger than the leading term of the denominator. Therefore, it will be growing at a faster rate.
Now, simply input the limit value, and interpret the results.
Example Question #1 : Asymptotic Behavior In Terms Of Limits Involving Infinity
Undefined.
For infinity limits, we need only consider the leading term in both the numerator and the denominator. Here, we have the case that the exponents are equal in the leading terms. Therefore, the limit at infinity is simply the ratio of the coefficients of the leading terms.
Example Question #5 : Asymptotic And Unbounded Behavior
Undefined.
Infinity limits can be found by only considering the leading term in both the numerator and the denominator. In this problem, the numerator has a higher exponent than the denominator. Therefore, it will keep increasing and increasing at a much faster rate. These limits always tend to infinity.
Example Question #6 : Asymptotic And Unbounded Behavior
Undefined.
For infinity limits, we only consider the leading term in both the numerator and the denominator. Then, we need to consider the exponents of the leading terms. Here, the denominator has a higher degree than the numerator. Therefore, we have a bottom heavy fraction. Even though we are evaluating the limit at negative infinity, this will still tend to zero because the denominator is growing at a faster rate. You can convince yourself of this by plugging in larger and larger negative values. You will just get a longer and smaller decimal.
Example Question #7 : Asymptotic And Unbounded Behavior

Example Question #1 : Functions, Graphs, And Limits

Certified Tutor
All AP Calculus AB Resources









































































