We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

AP Calculus AB : Functions, Graphs, and Limits

Study concepts, example questions & explanations for AP Calculus AB

Create An Account

All AP Calculus AB Resources

3 Diagnostic Tests 164 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

← Previous 1 2 3 4 5 6 7 8 9 … 20 21 Next →

Example Question #41 : Functions, Graphs, And Limits

Evaluate the following definite integral.

$\int_{1}^{3}6xdx$

Possible Answers:

3x^2+C

6x^2

Correct answer:

Explanation:

Unlike an indefinite integral, the definite integral must be evaluated at its limits, in this case, from 1 to 3. First, we use our inverse power rule to find the antiderivative. So, we have that 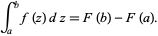$\int x^ndx=\frac{x^{n+1}}{n+1}$ . Once you find the antiderivative, we must remember that int_a^bf(z)dz=F(b)-F(a). where is the indefinite integral. So, we plug in our limits and subtract the two. So, we have (3x^2)|_1^3=3(3^2)-3(1^2)=24 .

Report an Error

Example Question #42 : Functions, Graphs, And Limits

Evaluate the following definite integral.

$\int_{1}^{4}\frac{1}{x}dx$

Possible Answers:

$ln\ 4$

$ln\ 1$

2x^2

$ln\ 3$

Correct answer:

$ln\ 4$

Explanation:

Unlike an indefinite integral, the definite integral must be evaluated at its limits, in this case, from 1 to 4. First, we use our inverse power rule to find the antiderivative. So since 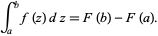is to the power of , we have that $\int x^{-1}=ln|x|$ . Once you find the antiderivative, we must remember that int_a^bf(z)dz=F(b)-F(a). where is the indefinite integral. So, we plug in our limits and subtract the two. So, we have ln(x)|_1^4=ln(4)-ln(1)=ln(4) because we know that $ln\ 1=0$ .

Report an Error

Example Question #43 : Functions, Graphs, And Limits

Evaluate the following indefinite integral.

$\int 4xdx$

Possible Answers:

x^2+C

8x^2+C

2x^2+C

(1/2)x^2

Correct answer:

2x^2+C

Explanation:

First, we know that we can pull the constant "4" out of the integral, and we then evaluate the integral according to this equation:

$\int x^n dx=\frac{x^{n+1}}{n+1}+C, n\ne1$ . From this, we acquire the answer above. As a note, we cannot forget the constant of integration which would be lost during the differentiation.

Report an Error

Example Question #44 : Functions, Graphs, And Limits

Evaluate the following indefinite integral.

$\int (6x+3x^2+4)dx$

Possible Answers:

x^2+x^3+4x+C

$\frac{1}{3}x^2+x^3+\frac{1}{4}x+C$

3x^2+x^3+4x

6+3x+C

3x^2+x^3+4x+C

Correct answer:

3x^2+x^3+4x+C

Explanation:

First, we know that the integral of a sum is the same as the sum of the integrals, so if needed, we can split the three integrals up and evaluate them seperately. We then evaluate each integral according to this equation:

$\int x^n dx=\frac{x^{n+1}}{n+1}+C, n\ne1$ . From this, we acquire the answer above. As a note, we cannot forget the constant of integration which would be lost during the differentiation.

Report an Error

Example Question #45 : Functions, Graphs, And Limits

Evalulate the following indefinite integral.

$\int \frac{1}{x}dx$

Possible Answers:

$1/2 x^{-2}+C$

-1/x^2+C

$1/2 x^{-2}$

e^x

ln(x)+C

Correct answer:

ln(x)+C

Explanation:

Normally, we would evalute the indefinite integral according to the following equation:

$\int x^n dx=\frac{x^{n+1}}{n+1}+C, n\ne1$ . However, in this case, n=-1 . Now we use our other rule that states the integral of $\frac{1}{x}$ is equal to $\ln (x)$ plus a constant. From this, we acquire the answer above. As a note, we cannot forget the constant of integration which would be lost during the differentiation.

Report an Error

Example Question #46 : Functions, Graphs, And Limits

Evaluate the following indefinite integral.

$\int\frac{1}{x^2}dx$

Possible Answers:

$-x^{-1}+C$

3x^3+C

(1/2)ln(x)+C

$\frac{-1}{3x^3}+C$

2ln(x)+C

Correct answer:

$-x^{-1}+C$

Explanation:

We evaluate the integral according to this equation:

$\int x^n dx=\frac{x^{n+1}}{n+1}+C, n\ne1$ . From this, we acquire the answer above. Keep in mind that $\frac{1}{x^{2}}$ is the same as $x^{-2}$ . As a note, we cannot forget the constant of integration which would be lost during the differentiation.

Report an Error

Example Question #47 : Functions, Graphs, And Limits

Evaluate the following indefinite integral.

$\int(3x^7+4x^4)dx$

Possible Answers:

$\frac{1}{8}x^8+\frac{1}{5}x^5+C$

$\frac{3}{8}x^8+\frac{4}{5}x^5+C$

$\frac{8}{3}x^8+\frac{5}{4}x^5+C$

8x^8+5x^5+C

3x^6+4x^3+C

Correct answer:

$\frac{3}{8}x^8+\frac{4}{5}x^5+C$

Explanation:

First, we remember that the integral of a sum is the same as the sum of the integrals, so we can split the sum into seperate integrals and solve them individually. We then evaluate each integral according to this equation:

$\int x^n dx=\frac{x^{n+1}}{n+1}+C, n\ne1$ . From this, we acquire the answer above. As a note, we cannot forget the constant of integration which would be lost during the differentiation.

Report an Error

Example Question #11 : Comparing Relative Magnitudes Of Functions And Their Rates Of Change

Evaluate the following indefinite integral.

$\int\frac{1}{2}x^2dx$

Possible Answers:

$\frac{1}{6}x^3+C$

$\frac{1}{2}x^3+C$

3x^3+C

6x^3+C

$\frac{1}{2}x^3+C$

Correct answer:

$\frac{1}{6}x^3+C$

Explanation:

First, we know that we can pull the constant $\frac{1}{2}$ out of the integral, and we then evaluate the integral according to this equation:

$\int x^n dx=\frac{x^{n+1}}{n+1}+C, n\ne1$ . From this, we acquire the answer above. As a note, we cannot forget the constant of integration which would be lost during the differentiation.

Report an Error

Example Question #21 : Comparing Relative Magnitudes Of Functions And Their Rates Of Change

Evaluate the following indefinite integral.

$\int \frac{3}{5}x^3dx$

Possible Answers:

$\frac{3}{20}x^4+C$

$\frac{9}{5}x^4+C$

$\frac{20}{3}x^4+C$

$\frac{1}{20}x^4+C$

$\frac{3}{15}x^4+C$

Correct answer:

$\frac{3}{20}x^4+C$

Explanation:

First, we know that we can pull the constant $\frac{3}{5}$ out of the integral, and we then evaluate the integral according to this equation:

$\int x^n dx=\frac{x^{n+1}}{n+1}+C, n\ne1$ . From this, we acquire the answer above. As a note, we cannot forget the constant of integration which would be lost during the differentiation.

Report an Error

Example Question #22 : Comparing Relative Magnitudes Of Functions And Their Rates Of Change

Evaluate the following indefinite integral.

$\int\sqrt{x}dx$

Possible Answers:

$3x^{-1/2}+C$

$\frac{2}{3}x^{\frac{3}{2}}+C$

ln(x)+C

e^x+C

$\frac{1}{3}x^{\frac{1}{2}}+C$

Correct answer:

$\frac{2}{3}x^{\frac{3}{2}}+C$

Explanation:

We evaluate the integral according to this equation:

$\int x^n dx=\frac{x^{n+1}}{n+1}+C, n\ne1$ . Keep in mind that $\sqrt{x}$ is the same as $x^{1/2}$ . From this, we acquire the answer above. As a note, we cannot forget the constant of integration which would be lost during the differentiation.

Report an Error

← Previous 1 2 3 4 5 6 7 8 9 … 20 21 Next →

View AP Calculus AB Tutors

Damion
Certified Tutor

Saint Edward's University, Bachelors, Accounting. The University of Texas at Austin, Master of Science, Accounting.

View AP Calculus AB Tutors

Christine
Certified Tutor

Johns Hopkins University, Bachelor of Science, Chemical Engineering.

View AP Calculus AB Tutors

Gregory
Certified Tutor

Pennsylvania State University-Main Campus, Bachelor of Science, Chemical Engineering. Carnegie Mellon University, Doctor of P...

All AP Calculus AB Resources

3 Diagnostic Tests 164 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

ISEE Tutors in New York City, GMAT Tutors in San Francisco-Bay Area, Computer Science Tutors in Seattle, Algebra Tutors in Denver, SSAT Tutors in Seattle, Math Tutors in Phoenix, SAT Tutors in Los Angeles, Statistics Tutors in Dallas Fort Worth, Computer Science Tutors in San Francisco-Bay Area, MCAT Tutors in San Diego

Popular Courses & Classes

MCAT Courses & Classes in Seattle, SAT Courses & Classes in San Diego, Spanish Courses & Classes in San Diego, LSAT Courses & Classes in Miami, Spanish Courses & Classes in Denver, GRE Courses & Classes in Los Angeles, ACT Courses & Classes in Miami, LSAT Courses & Classes in San Francisco-Bay Area, SAT Courses & Classes in Miami, GMAT Courses & Classes in New York City

Popular Test Prep

ISEE Test Prep in Houston, GMAT Test Prep in San Diego, LSAT Test Prep in Houston, LSAT Test Prep in San Diego, GRE Test Prep in Chicago, ISEE Test Prep in Boston, ACT Test Prep in Miami, LSAT Test Prep in Philadelphia, MCAT Test Prep in Dallas Fort Worth, ISEE Test Prep in Washington DC

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

AP Calculus AB : Functions, Graphs, and Limits

Study concepts, example questions & explanations for AP Calculus AB

All AP Calculus AB Resources

Example Questions

Example Question #41 : Functions, Graphs, And Limits

Example Question #42 : Functions, Graphs, And Limits

Example Question #43 : Functions, Graphs, And Limits

Example Question #44 : Functions, Graphs, And Limits

Example Question #45 : Functions, Graphs, And Limits

Example Question #46 : Functions, Graphs, And Limits

Example Question #47 : Functions, Graphs, And Limits

Example Question #11 : Comparing Relative Magnitudes Of Functions And Their Rates Of Change

Example Question #21 : Comparing Relative Magnitudes Of Functions And Their Rates Of Change

Example Question #22 : Comparing Relative Magnitudes Of Functions And Their Rates Of Change

All AP Calculus AB Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: